Question and Answers Forum
Question Number 8224 by trapti rathaur@ gmail.com last updated on 03/Oct/16

Commented by Yozzias last updated on 03/Oct/16
![RTP: If b∈(((−π)/4),(π/4))⇒b^2 =Σ_(r=1) ^∞ (−1)^(r−1) (1/r){Σ_(k=1) ^r (1/(2k−1))}tan^(2r) b. or b^2 =lim_(n→∞) [Σ_(r=1) ^n (−1)^(r−1) (1/r){Σ_(k=1) ^r (1/(2k−1))}tan^(2r) b]](Q8225.png)
Commented by trapti rathaur@ gmail.com last updated on 03/Oct/16
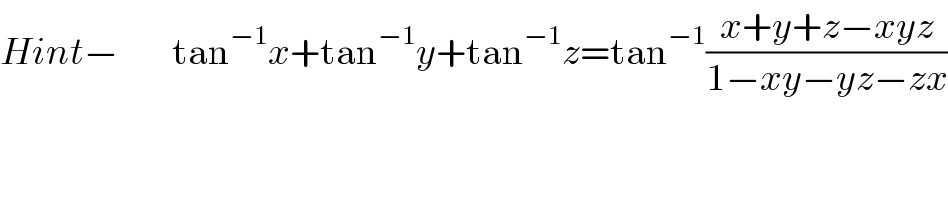
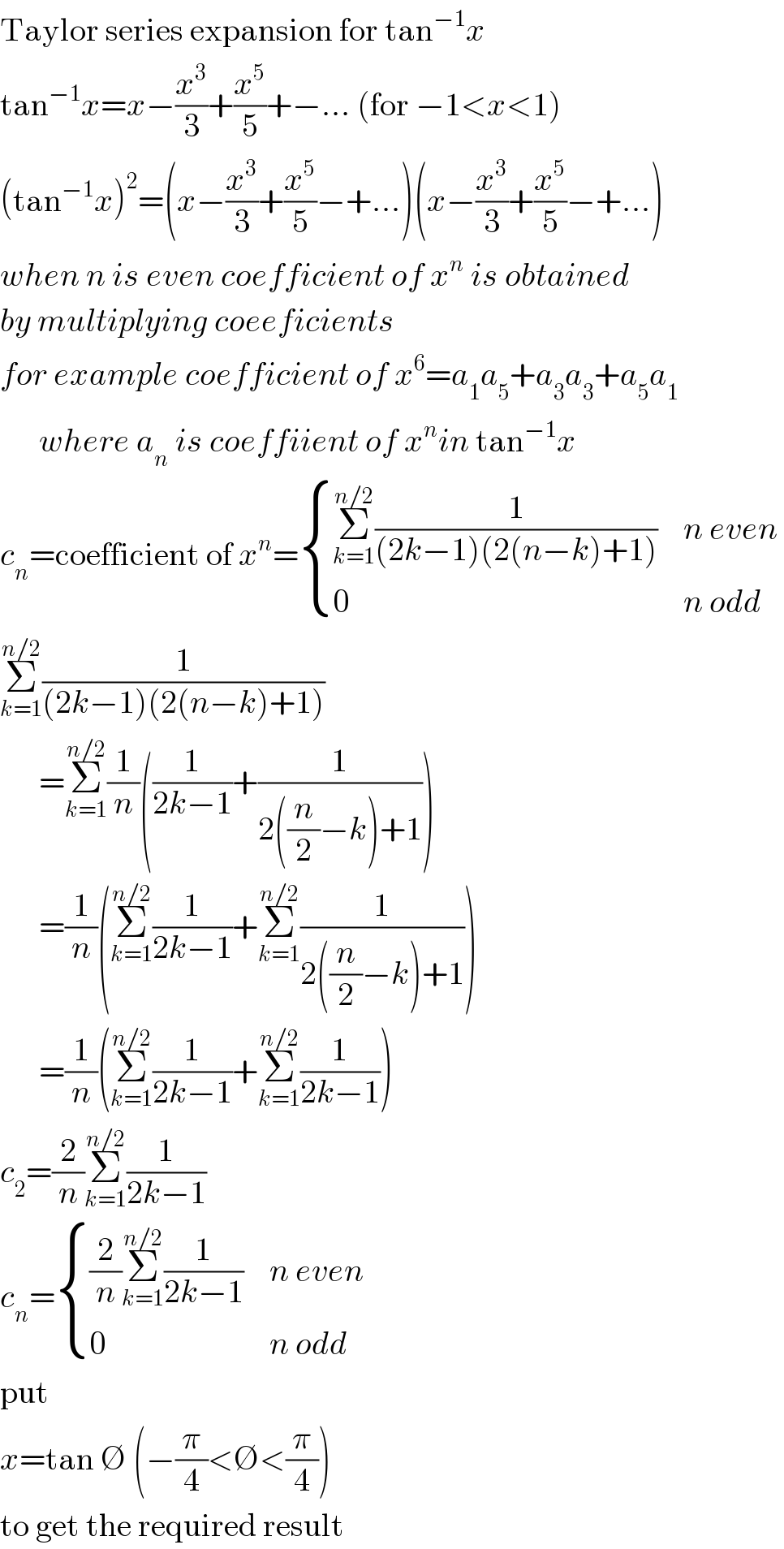
Commented by trapti rathaur@ gmail.com last updated on 03/Oct/16

Answered by prakash jain last updated on 03/Oct/16