Question Number 82247 by jagoll last updated on 19/Feb/20
$$\left({D}^{\mathrm{4}} +\mathrm{2}{D}^{\mathrm{2}} +\mathrm{1}\right){y}\:={x}^{\mathrm{2}} \:\mathrm{cos}\:{x}\: \\ $$
Commented by john santu last updated on 20/Feb/20
$${characteristic}\:{equation}\: \\ $$$$\lambda^{\mathrm{4}} +\mathrm{2}\lambda^{\mathrm{2}} +\mathrm{1}\:=\:\mathrm{0}\: \\ $$$$\left(\lambda^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} \:=\:\mathrm{0}\:\Rightarrow\left(\lambda^{\mathrm{2}} −{i}\right)^{\mathrm{2}\:} \left(\lambda^{\mathrm{2}} +{i}\right)^{\mathrm{2}} \:=\mathrm{0} \\ $$$$\lambda^{\mathrm{2}} \:=\:{i}\:\Rightarrow\:\lambda_{\mathrm{1},\mathrm{2}\:} \:=\:\pm\:\sqrt{\:{i}}\: \\ $$$$\lambda^{\mathrm{2}} \:=\:−{i}\:\Rightarrow\:\lambda_{\mathrm{3},\mathrm{4}} \:=\:\pm\:\sqrt{−{i}\:}\: \\ $$$${y}_{{h}} \:=\:{Ae}\:^{\sqrt{\:{i}}} \:+\:{Be}^{−\sqrt{{i}\:}} +{Ce}^{−\sqrt{−{i}\:}} +{De}^{\sqrt{\:−{i}\:}} \\ $$
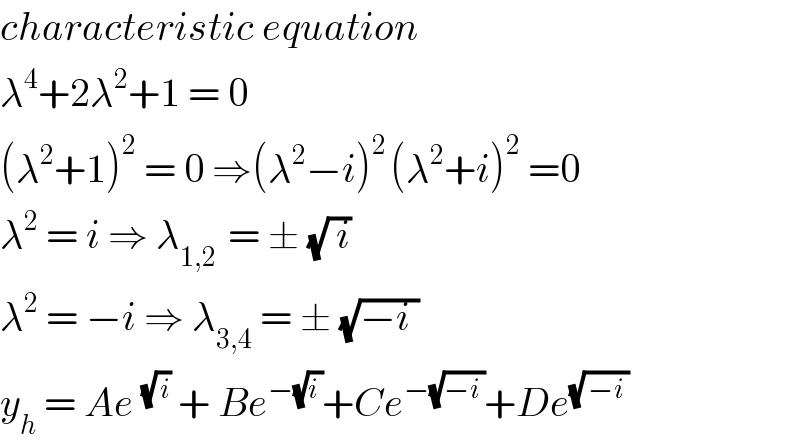
Answered by TANMAY PANACEA last updated on 20/Feb/20
![y=((x^2 cosx)/((D^2 +1)^2 )) y_(real) +y_(imaginary) =((x^2 e^(ix) )/((D^2 +1)^2 )) =(e^(ix) /([(D+i)^2 +1]^2 ))×x^2 =e^(ix) ×(1/((D^2 +2iD)^2 ))×x^2 =e^(ix) ×(1/D^2 )×(1/((D+2i)^2 ))×x^2 =e^(ix) ×(1/(−4(1+(D/(2i)))^2 ))×(x^4 /(12)) [(1/D^2 )×x^2 =(1/D)×(x^3 /3)=(x^4 /(12))] now using (1−a)^(−2) =1+2a+3a^2 +4a^3 +5a^4 +6a^5 +...+(r+1)a^r put a=−(D/(2i)) =(((cosx+isinx))/(−48))×(1−2.(D/(2i))+3×(D^2 /(−4))+4×(D^3 /(8i))+5×(D^4 /(16)) others terms ignored)x^4 =(((cosx+isinx))/(−48))(x^4 −((4x^3 )/i)−(3/4)×12x^2 +(1/(2i))×24x+(5/(16))×24) =((cosx+isinx)/(−48))(x^4 −9x^2 +((15)/2)+i×4x^3 −i×12x) required answer is real part =((cosx)/(−48))(x^4 −9x^2 +((15)/2))+((sinx)/(−48))×(−1)(4x^3 −12x) pls check mistakd if any](Q82295.png)
$${y}=\frac{{x}^{\mathrm{2}} {cosx}}{\left({D}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${y}_{{real}} +{y}_{{imaginary}} =\frac{{x}^{\mathrm{2}} {e}^{{ix}} }{\left({D}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\frac{{e}^{{ix}} }{\left[\left({D}+{i}\right)^{\mathrm{2}} +\mathrm{1}\right]^{\mathrm{2}} }×{x}^{\mathrm{2}} \\ $$$$={e}^{{ix}} ×\frac{\mathrm{1}}{\left({D}^{\mathrm{2}} +\mathrm{2}{iD}\right)^{\mathrm{2}} }×{x}^{\mathrm{2}} \\ $$$$={e}^{{ix}} ×\frac{\mathrm{1}}{{D}^{\mathrm{2}} }×\frac{\mathrm{1}}{\left({D}+\mathrm{2}{i}\right)^{\mathrm{2}} }×{x}^{\mathrm{2}} \\ $$$$={e}^{{ix}} ×\frac{\mathrm{1}}{−\mathrm{4}\left(\mathrm{1}+\frac{{D}}{\mathrm{2}{i}}\right)^{\mathrm{2}} }×\frac{{x}^{\mathrm{4}} }{\mathrm{12}}\:\:\:\left[\frac{\mathrm{1}}{{D}^{\mathrm{2}} }×{x}^{\mathrm{2}} =\frac{\mathrm{1}}{{D}}×\frac{{x}^{\mathrm{3}} }{\mathrm{3}}=\frac{{x}^{\mathrm{4}} }{\mathrm{12}}\right] \\ $$$${now}\:{using} \\ $$$$\left(\mathrm{1}−{a}\right)^{−\mathrm{2}} =\mathrm{1}+\mathrm{2}{a}+\mathrm{3}{a}^{\mathrm{2}} +\mathrm{4}{a}^{\mathrm{3}} +\mathrm{5}{a}^{\mathrm{4}} +\mathrm{6}{a}^{\mathrm{5}} +...+\left({r}+\mathrm{1}\right){a}^{{r}} \\ $$$${put}\:{a}=−\frac{{D}}{\mathrm{2}{i}} \\ $$$$=\frac{\left({cosx}+{isinx}\right)}{−\mathrm{48}}×\left(\mathrm{1}−\mathrm{2}.\frac{{D}}{\mathrm{2}{i}}+\mathrm{3}×\frac{{D}^{\mathrm{2}} }{−\mathrm{4}}+\mathrm{4}×\frac{{D}^{\mathrm{3}} }{\mathrm{8}{i}}+\mathrm{5}×\frac{{D}^{\mathrm{4}} }{\mathrm{16}}\:{others}\:{terms}\:{ignored}\right){x}^{\mathrm{4}} \\ $$$$=\frac{\left({cosx}+{isinx}\right)}{−\mathrm{48}}\left({x}^{\mathrm{4}} −\frac{\mathrm{4}{x}^{\mathrm{3}} }{{i}}−\frac{\mathrm{3}}{\mathrm{4}}×\mathrm{12}{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}{i}}×\mathrm{24}{x}+\frac{\mathrm{5}}{\mathrm{16}}×\mathrm{24}\right) \\ $$$$=\frac{{cosx}+{isinx}}{−\mathrm{48}}\left({x}^{\mathrm{4}} −\mathrm{9}{x}^{\mathrm{2}} +\frac{\mathrm{15}}{\mathrm{2}}+{i}×\mathrm{4}{x}^{\mathrm{3}} −{i}×\mathrm{12}{x}\right) \\ $$$${required}\:{answer}\:{is}\:{real}\:{part} \\ $$$$=\frac{{cosx}}{−\mathrm{48}}\left({x}^{\mathrm{4}} −\mathrm{9}{x}^{\mathrm{2}} +\frac{\mathrm{15}}{\mathrm{2}}\right)+\frac{{sinx}}{−\mathrm{48}}×\left(−\mathrm{1}\right)\left(\mathrm{4}{x}^{\mathrm{3}} −\mathrm{12}{x}\right) \\ $$$${pls}\:{check}\:{mistakd}\:{if}\:{any} \\ $$
Commented by jagoll last updated on 20/Feb/20
$${thank}\:{sir}\: \\ $$
Commented by TANMAY PANACEA last updated on 20/Feb/20
$${most}\:{welcome} \\ $$