
Question and Answers Forum
Question Number 82546 by M±th+et£s last updated on 22/Feb/20
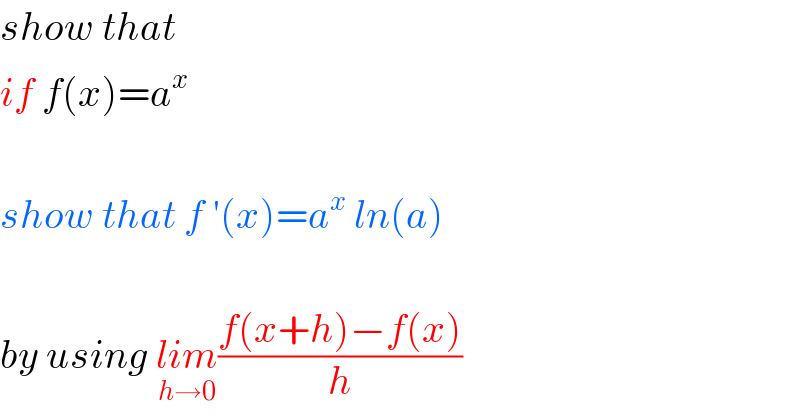
Commented by niroj last updated on 22/Feb/20
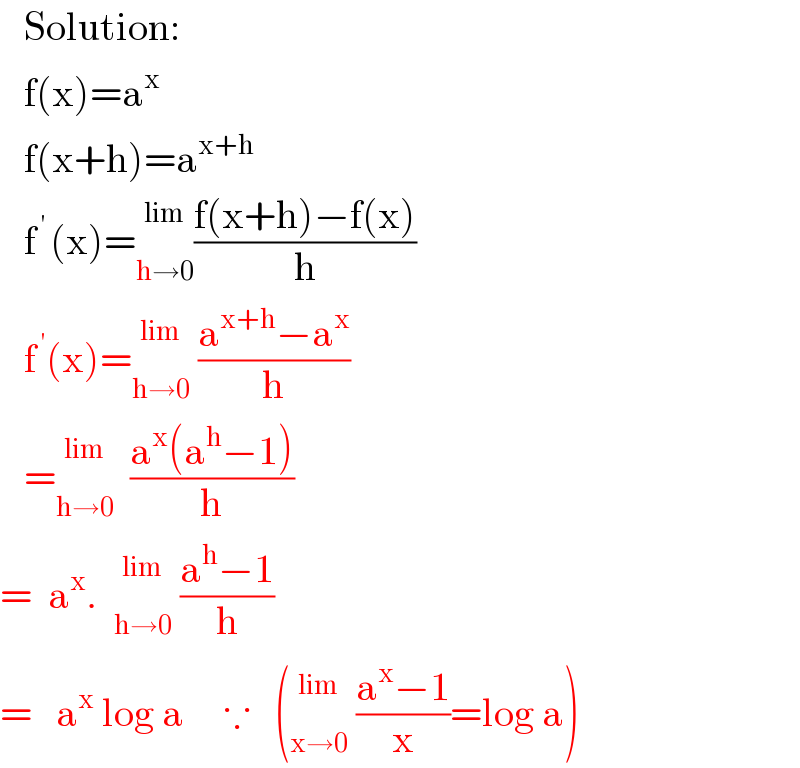
Answered by TANMAY PANACEA last updated on 22/Feb/20
![lim_(h→0) ((a^(x+h) −a^x )/h) a^x ×lim_(h→0) ((a^h −1)/h) [now a^h =e^(hlna) ] a^x ×lim_(h→0) ((e^(hlna) −1)/(hlna))×lna a^x ×1×lna a^x lna](Q82558.png)
| ||
Question and Answers Forum | ||
Question Number 82546 by M±th+et£s last updated on 22/Feb/20 | ||
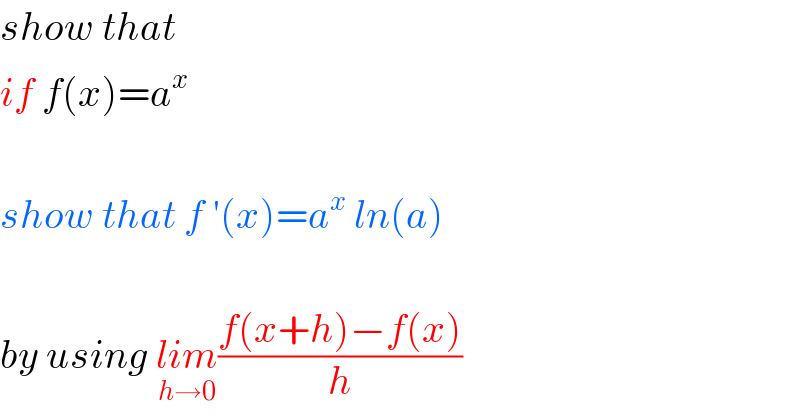 | ||
Commented by niroj last updated on 22/Feb/20 | ||
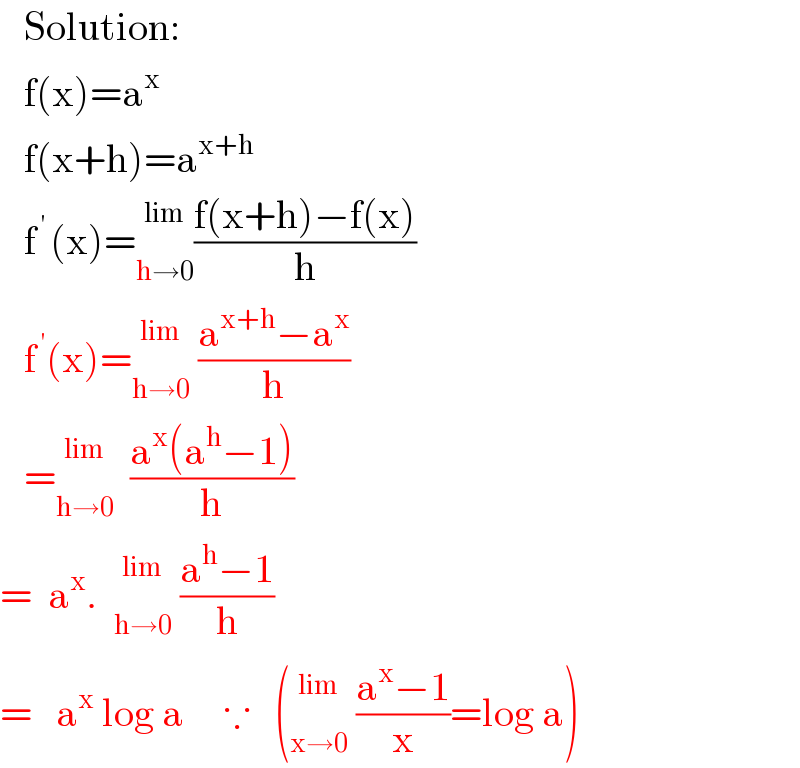 | ||
Answered by TANMAY PANACEA last updated on 22/Feb/20 | ||
![lim_(h→0) ((a^(x+h) −a^x )/h) a^x ×lim_(h→0) ((a^h −1)/h) [now a^h =e^(hlna) ] a^x ×lim_(h→0) ((e^(hlna) −1)/(hlna))×lna a^x ×1×lna a^x lna](Q82558.png) | ||
| ||