
Question and Answers Forum
Question Number 83202 by Rio Michael last updated on 28/Feb/20
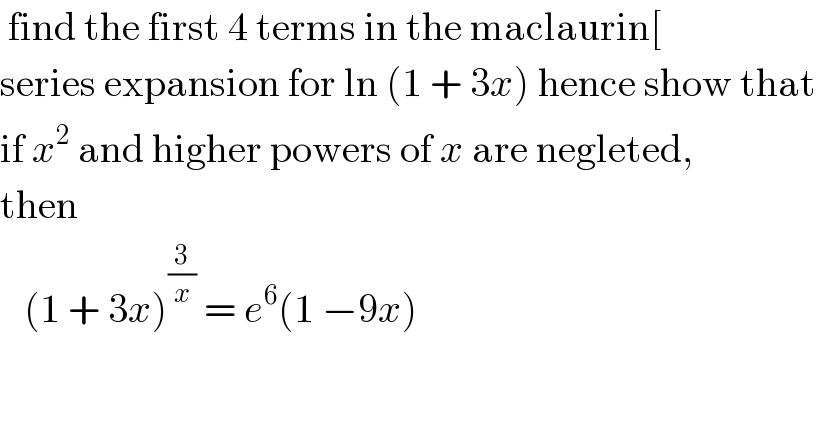
Commented by mr W last updated on 28/Feb/20

Commented by Rio Michael last updated on 28/Feb/20
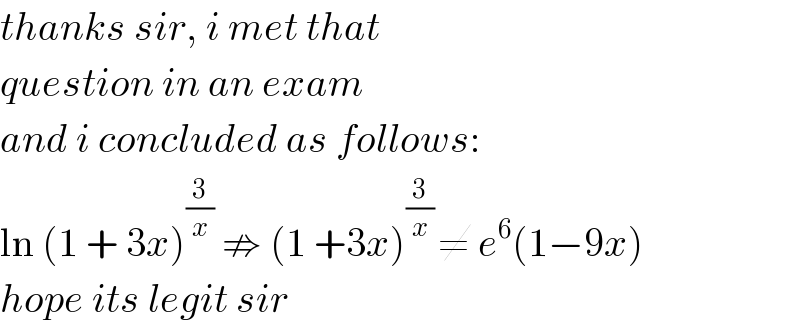
Commented by mr W last updated on 28/Feb/20

Commented by Rio Michael last updated on 28/Feb/20

