
Question and Answers Forum
Question Number 83781 by john santu last updated on 06/Mar/20
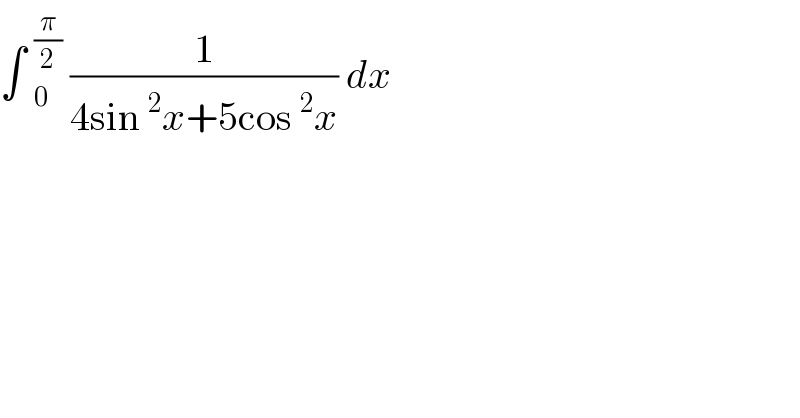
Commented by niroj last updated on 06/Mar/20
![∫_0 ^(π/2) (( 1)/(4sin^2 x+5cos^2 x))dx = ∫_0 ^(π/2) (( sec^2 x dx)/( 4tan^2 x+5)) put tan x=t sec^2 xdx=dt if x=(π/2)then t=∞ if x=0 then t=0 = ∫_0 ^( ∞) (1/(4t^2 +5))dt = (1/4)∫_0 ^( ∞) (1/(t^2 +(5/4)))dt = (1/4)[∫ (1/((t)^2 +(((√5)/2))^2 ))dt]_0 ^∞ = (1/4)[ (1/((√5)/2)) tan^(−1) (t/((√5)/2))]_0 ^∞ = (1/4)[ (2/(√5))tan^(−1) (∞).(2/(√5))−0] = (1/4).(2/(√5)). (π/2)= (π/(4(√5)))//.](Q83784.png)
Commented by john santu last updated on 06/Mar/20
![in short cut = ∫ _0^(π/2) (dx/(a^2 sin^2 x+b^2 cos^2 x)) = (π/(2ab)) [ a=(√4) = 2 , b =(√5) ] = (π/(2.2.(√5))) = (π/(4(√5))) ★](Q83785.png)
Answered by MJS last updated on 06/Mar/20
![∫(dx/(4sin^2 x +5cos^2 x))= [t=tan x → dx=(dt/(t^2 +1)); sin x =(t/(√(t^2 +1))); cos x =(1/(√(t^2 +1)))] =∫(dt/(4t^2 +5))=((√5)/(10))arctan ((2(√5)t)/5) = =((√5)/(10))arctan ((2(√5)tan x)/5) +C snswer is ((π(√5))/(20))](Q83801.png)
| ||
Question and Answers Forum | ||
Question Number 83781 by john santu last updated on 06/Mar/20 | ||
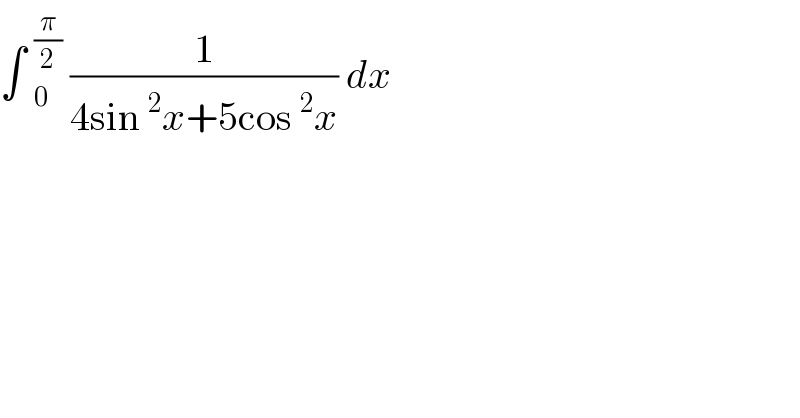 | ||
Commented by niroj last updated on 06/Mar/20 | ||
![∫_0 ^(π/2) (( 1)/(4sin^2 x+5cos^2 x))dx = ∫_0 ^(π/2) (( sec^2 x dx)/( 4tan^2 x+5)) put tan x=t sec^2 xdx=dt if x=(π/2)then t=∞ if x=0 then t=0 = ∫_0 ^( ∞) (1/(4t^2 +5))dt = (1/4)∫_0 ^( ∞) (1/(t^2 +(5/4)))dt = (1/4)[∫ (1/((t)^2 +(((√5)/2))^2 ))dt]_0 ^∞ = (1/4)[ (1/((√5)/2)) tan^(−1) (t/((√5)/2))]_0 ^∞ = (1/4)[ (2/(√5))tan^(−1) (∞).(2/(√5))−0] = (1/4).(2/(√5)). (π/2)= (π/(4(√5)))//.](Q83784.png) | ||
Commented by john santu last updated on 06/Mar/20 | ||
![in short cut = ∫ _0^(π/2) (dx/(a^2 sin^2 x+b^2 cos^2 x)) = (π/(2ab)) [ a=(√4) = 2 , b =(√5) ] = (π/(2.2.(√5))) = (π/(4(√5))) ★](Q83785.png) | ||
Answered by MJS last updated on 06/Mar/20 | ||
![∫(dx/(4sin^2 x +5cos^2 x))= [t=tan x → dx=(dt/(t^2 +1)); sin x =(t/(√(t^2 +1))); cos x =(1/(√(t^2 +1)))] =∫(dt/(4t^2 +5))=((√5)/(10))arctan ((2(√5)t)/5) = =((√5)/(10))arctan ((2(√5)tan x)/5) +C snswer is ((π(√5))/(20))](Q83801.png) | ||
| ||