
Question and Answers Forum
Question Number 85061 by M±th+et£s last updated on 18/Mar/20
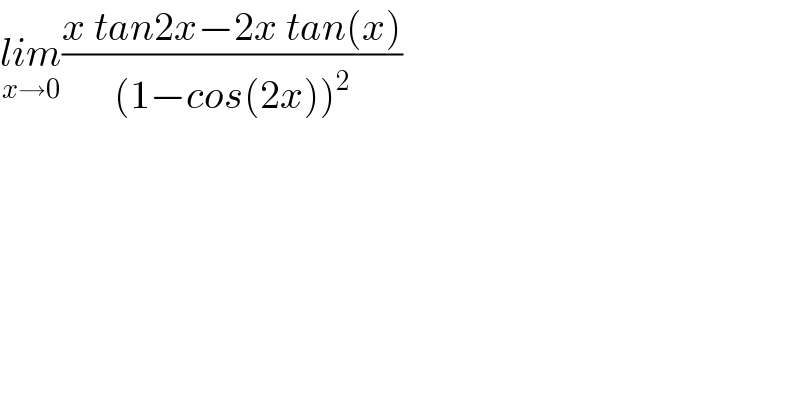
Commented by mathmax by abdo last updated on 18/Mar/20

Commented by M±th+et£s last updated on 18/Mar/20

Commented by mathmax by abdo last updated on 18/Mar/20

Answered by john santu last updated on 19/Mar/20

Commented by M±th+et£s last updated on 19/Mar/20

