Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 85127 by Rio Michael last updated on 19/Mar/20

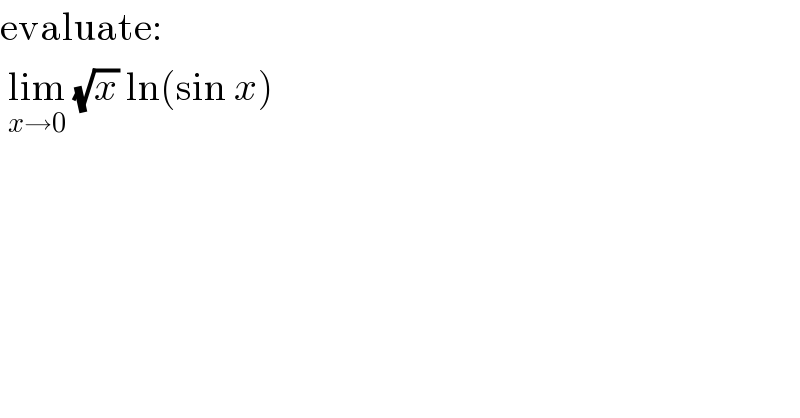
evaluate: lim_(x→0) (√x) ln(sin x)

$$\mathrm{evaluate}: \\ $$$$\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\sqrt{{x}}\:\mathrm{ln}\left(\mathrm{sin}\:{x}\right) \\ $$$$ \\ $$

Commented by john santu last updated on 19/Mar/20

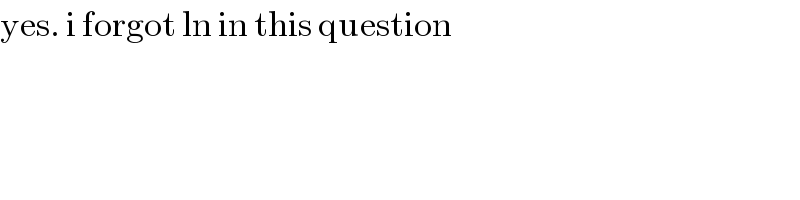
yes. i forgot ln in this question

$$\mathrm{yes}.\:\mathrm{i}\:\mathrm{forgot}\:\mathrm{ln}\:\mathrm{in}\:\mathrm{this}\:\mathrm{question} \\ $$

Commented by MJS last updated on 19/Mar/20

there′s something wrong

$$\mathrm{there}'\mathrm{s}\:\mathrm{something}\:\mathrm{wrong} \\ $$

Commented by jagoll last updated on 19/Mar/20

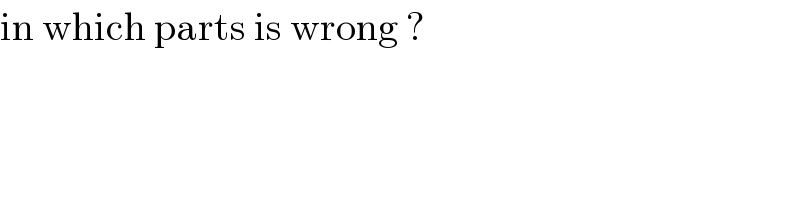
in which parts is wrong ?

$$\mathrm{in}\:\mathrm{which}\:\mathrm{parts}\:\mathrm{is}\:\mathrm{wrong}\:? \\ $$

Commented by MJS last updated on 19/Mar/20

(1) lim_(x→0) ln (sin x)^(√x) ≠lim_(x→0) (1+(sin x −1))^(√x) (2) how did you get the 3^(rd) line from the 2^(nd) one? lim_(x→0) ((1+u)^(1/u) )^(uv) ⇒^? e^(lim_(x→0) uv)

$$\left(\mathrm{1}\right)\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{ln}\:\left(\mathrm{sin}\:{x}\right)^{\sqrt{{x}}} \:\neq\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\mathrm{1}+\left(\mathrm{sin}\:{x}\:−\mathrm{1}\right)\right)^{\sqrt{{x}}} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{how}\:\mathrm{did}\:\mathrm{you}\:\mathrm{get}\:\mathrm{the}\:\mathrm{3}^{\mathrm{rd}} \:\mathrm{line}\:\mathrm{from}\:\mathrm{the}\:\mathrm{2}^{\mathrm{nd}} \:\mathrm{one}? \\ $$$$\:\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\left(\mathrm{1}+{u}\right)^{\frac{\mathrm{1}}{{u}}} \right)^{{uv}} \overset{?} {\Rightarrow}\:\mathrm{e}^{\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:{uv}} \\ $$

Answered by MJS last updated on 19/Mar/20

L=lim_(x→0) (√x)ln sin x =lim_(x→0) ((xln sin x)/(√x)) = =lim_(x→0) (((d/dx)[xln sin x])/((d/dx)[(√x)])) = lim_(x→0) (((x/(tan x))+ln sin x)/(1/(2(√x)))) = =2lim_(x→0) (((√x^3 )/(tan x))+(√x)ln sin x) ⇒ L=2L+2lim_(x→0) ((√x^3 )/(tan x)) ⇒ L=−2lim_(x→0) ((√x^3 )/(tan x)) =−2lim_(x→0) (((d/dx)[(√x^3 )])/((d/dx)[tan x])) = =−2lim_(x→0) (((3/2)(√x))/(1/(cos^2 x))) =−3lim_(x→0) (√x)cos^2 x =0

$${L}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\sqrt{{x}}\mathrm{ln}\:\mathrm{sin}\:{x}\:=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}\mathrm{ln}\:\mathrm{sin}\:{x}}{\sqrt{{x}}}\:= \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{{d}}{{dx}}\left[{x}\mathrm{ln}\:\mathrm{sin}\:{x}\right]}{\frac{{d}}{{dx}}\left[\sqrt{{x}}\right]}\:=\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{{x}}{\mathrm{tan}\:{x}}+\mathrm{ln}\:\mathrm{sin}\:{x}}{\frac{\mathrm{1}}{\mathrm{2}\sqrt{{x}}}}\:= \\ $$$$=\mathrm{2}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\frac{\sqrt{{x}^{\mathrm{3}} }}{\mathrm{tan}\:{x}}+\sqrt{{x}}\mathrm{ln}\:\mathrm{sin}\:{x}\right) \\ $$$$\Rightarrow \\ $$$${L}=\mathrm{2}{L}+\mathrm{2}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{{x}^{\mathrm{3}} }}{\mathrm{tan}\:{x}} \\ $$$$\Rightarrow \\ $$$${L}=−\mathrm{2}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{{x}^{\mathrm{3}} }}{\mathrm{tan}\:{x}}\:=−\mathrm{2}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{{d}}{{dx}}\left[\sqrt{{x}^{\mathrm{3}} }\right]}{\frac{{d}}{{dx}}\left[\mathrm{tan}\:{x}\right]}\:= \\ $$$$=−\mathrm{2}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\frac{\mathrm{3}}{\mathrm{2}}\sqrt{{x}}}{\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \:{x}}}\:=−\mathrm{3}\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\sqrt{{x}}\mathrm{cos}^{\mathrm{2}} \:{x}\:=\mathrm{0} \\ $$

Commented by Rio Michael last updated on 19/Mar/20

thanks y′all

$${thanks}\:{y}'{all} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com