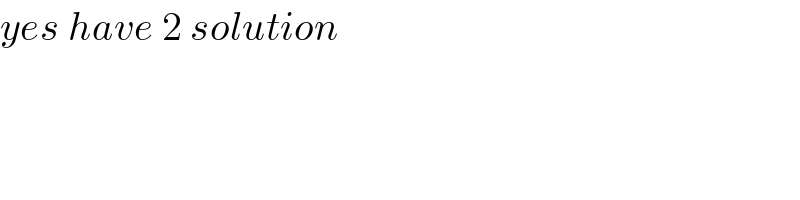Question and Answers Forum
Question Number 85667 by john santu last updated on 23/Mar/20
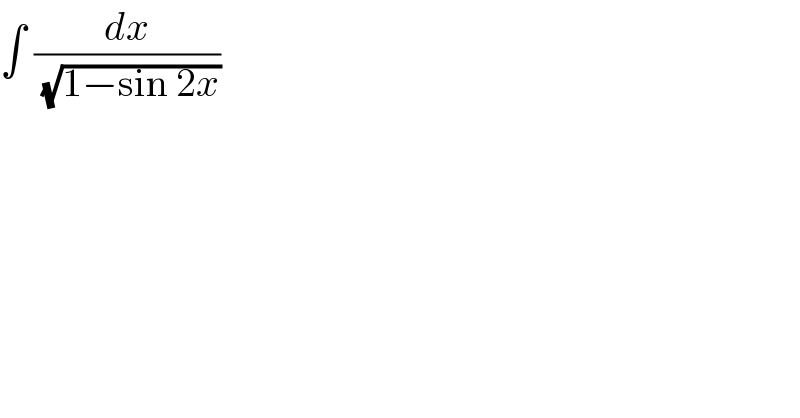
Answered by som(math1967) last updated on 24/Mar/20
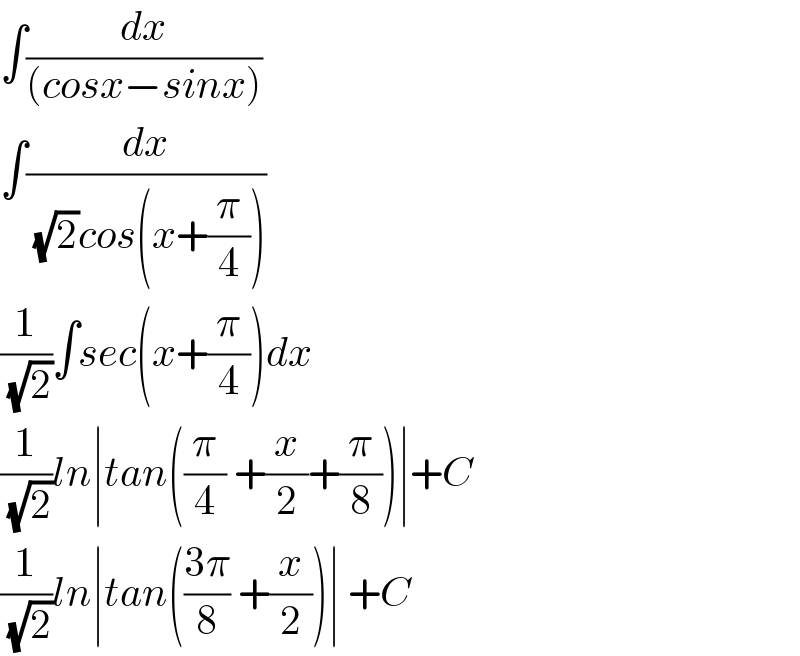
Commented by jagoll last updated on 24/Mar/20
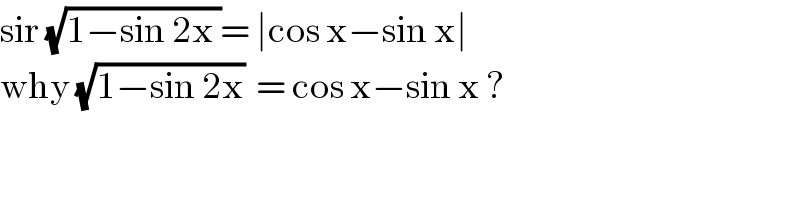
Commented by jagoll last updated on 24/Mar/20
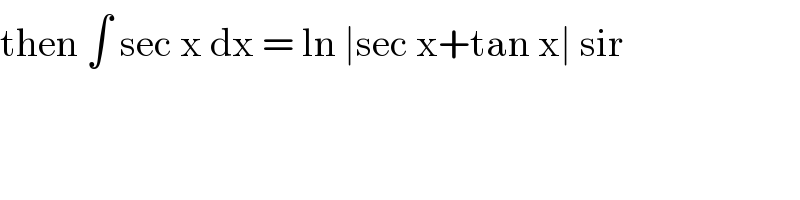
Commented by som(math1967) last updated on 24/Mar/20
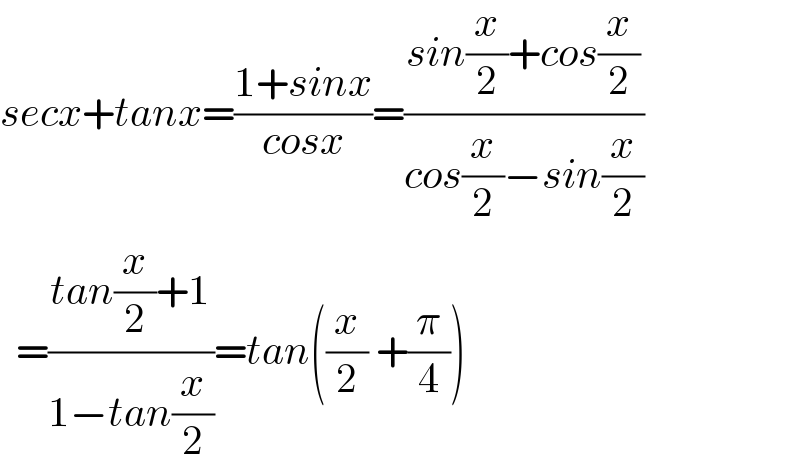
Commented by jagoll last updated on 24/Mar/20
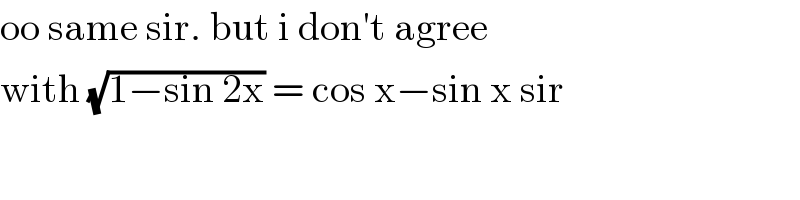
Commented by jagoll last updated on 24/Mar/20

Commented by som(math1967) last updated on 24/Mar/20
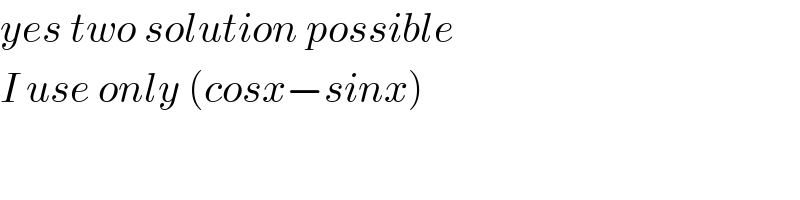
Commented by jagoll last updated on 24/Mar/20

Commented by john santu last updated on 24/Mar/20