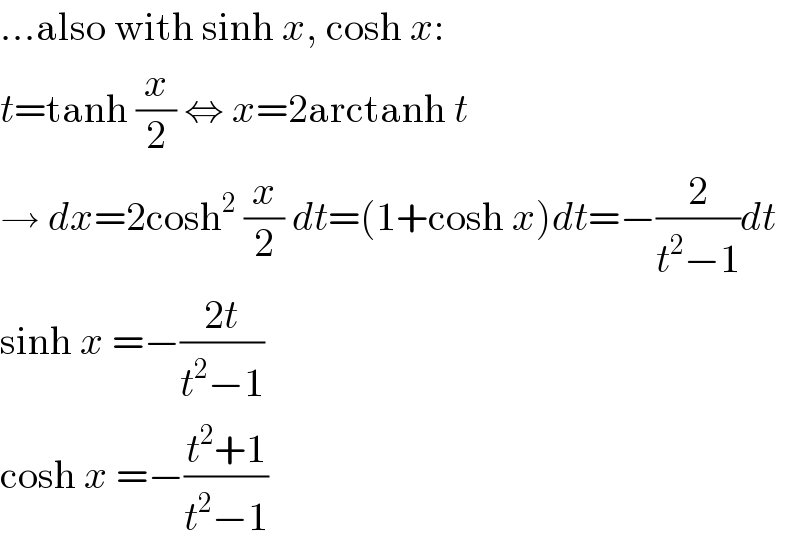Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 85776 by M±th+et£s last updated on 24/Mar/20

Answered by MJS last updated on 24/Mar/20

∫((a sin^2 x +2b sin x cos x +c cos^2 x)/(sin x +cos x))dx= [t=tan (x/2) → dx=(2/(t^2 +1))dt] =−2∫((ct^4 −4bt^3 +2(2a−c)t^2 +4bt+c)/((t^2 +1)^2 (t−1−(√2))(t−1+(√2))))dt= =2(a+2b−c)∫((t−((a−2b−c)/(a+2b−c)))/((t^2 +1)^2 ))dt+ +(a−2b−c)∫(dt/(t^2 +1))− −(((√2)(a−2b+c))/4)∫(dt/(t−1−(√2)))+ +(((√2)(a−2b+c))/4)∫(dt/(t−1+(√2))) I think you can solve these

$$\int\frac{{a}\:\mathrm{sin}^{\mathrm{2}} \:{x}\:+\mathrm{2}{b}\:\mathrm{sin}\:{x}\:\mathrm{cos}\:{x}\:+{c}\:\mathrm{cos}^{\mathrm{2}} \:{x}}{\mathrm{sin}\:{x}\:+\mathrm{cos}\:{x}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\:\rightarrow\:{dx}=\frac{\mathrm{2}}{{t}^{\mathrm{2}} +\mathrm{1}}{dt}\right] \\ $$$$=−\mathrm{2}\int\frac{{ct}^{\mathrm{4}} −\mathrm{4}{bt}^{\mathrm{3}} +\mathrm{2}\left(\mathrm{2}{a}−{c}\right){t}^{\mathrm{2}} +\mathrm{4}{bt}+{c}}{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} \left({t}−\mathrm{1}−\sqrt{\mathrm{2}}\right)\left({t}−\mathrm{1}+\sqrt{\mathrm{2}}\right)}{dt}= \\ $$$$=\mathrm{2}\left({a}+\mathrm{2}{b}−{c}\right)\int\frac{{t}−\frac{{a}−\mathrm{2}{b}−{c}}{{a}+\mathrm{2}{b}−{c}}}{\left({t}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }{dt}+ \\ $$$$\:\:\:\:\:+\left({a}−\mathrm{2}{b}−{c}\right)\int\frac{{dt}}{{t}^{\mathrm{2}} +\mathrm{1}}− \\ $$$$\:\:\:\:\:−\frac{\sqrt{\mathrm{2}}\left({a}−\mathrm{2}{b}+{c}\right)}{\mathrm{4}}\int\frac{{dt}}{{t}−\mathrm{1}−\sqrt{\mathrm{2}}}+ \\ $$$$\:\:\:\:\:+\frac{\sqrt{\mathrm{2}}\left({a}−\mathrm{2}{b}+{c}\right)}{\mathrm{4}}\int\frac{{dt}}{{t}−\mathrm{1}+\sqrt{\mathrm{2}}} \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{you}\:\mathrm{can}\:\mathrm{solve}\:\mathrm{these} \\ $$

Commented by M±th+et£s last updated on 24/Mar/20

yes sir thank you its easy to solve now

$${yes}\:{sir}\:{thank}\:{you}\:{its}\:{easy}\:{to}\:{solve}\:{now} \\ $$

Commented by MJS last updated on 24/Mar/20

you can always use this substitution (“Weierstrass”) for integrals with trigonometric functions t=tan (x/2) ⇔ x=2arctan t → dx=2cos^2 (x/2) dt=(1+cos x)dt=(2/(t^2 +1))dt sin x =((2t)/(t^2 +1)) cos x =−((t^2 −1)/(t^2 +1)) the main problem is to factorize the denominator...

$$\mathrm{you}\:\mathrm{can}\:\mathrm{always}\:\mathrm{use}\:\mathrm{this}\:\mathrm{substitution} \\ $$$$\left(``\mathrm{Weierstrass}''\right)\:\mathrm{for}\:\mathrm{integrals}\:\mathrm{with} \\ $$$$\mathrm{trigonometric}\:\mathrm{functions} \\ $$$${t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\:\Leftrightarrow\:{x}=\mathrm{2arctan}\:{t} \\ $$$$\rightarrow\:{dx}=\mathrm{2cos}^{\mathrm{2}} \:\frac{{x}}{\mathrm{2}}\:{dt}=\left(\mathrm{1}+\mathrm{cos}\:{x}\right){dt}=\frac{\mathrm{2}}{{t}^{\mathrm{2}} +\mathrm{1}}{dt} \\ $$$$\mathrm{sin}\:{x}\:=\frac{\mathrm{2}{t}}{{t}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{cos}\:{x}\:=−\frac{{t}^{\mathrm{2}} −\mathrm{1}}{{t}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{the}\:\mathrm{main}\:\mathrm{problem}\:\mathrm{is}\:\mathrm{to}\:\mathrm{factorize}\:\mathrm{the} \\ $$$$\mathrm{denominator}... \\ $$

Commented by MJS last updated on 24/Mar/20

...also with sinh x, cosh x: t=tanh (x/2) ⇔ x=2arctanh t → dx=2cosh^2 (x/2) dt=(1+cosh x)dt=−(2/(t^2 −1))dt sinh x =−((2t)/(t^2 −1)) cosh x =−((t^2 +1)/(t^2 −1))

$$...\mathrm{also}\:\mathrm{with}\:\mathrm{sinh}\:{x},\:\mathrm{cosh}\:{x}: \\ $$$${t}=\mathrm{tanh}\:\frac{{x}}{\mathrm{2}}\:\Leftrightarrow\:{x}=\mathrm{2arctanh}\:{t} \\ $$$$\rightarrow\:{dx}=\mathrm{2cosh}^{\mathrm{2}} \:\frac{{x}}{\mathrm{2}}\:{dt}=\left(\mathrm{1}+\mathrm{cosh}\:{x}\right){dt}=−\frac{\mathrm{2}}{{t}^{\mathrm{2}} −\mathrm{1}}{dt} \\ $$$$\mathrm{sinh}\:{x}\:=−\frac{\mathrm{2}{t}}{{t}^{\mathrm{2}} −\mathrm{1}} \\ $$$$\mathrm{cosh}\:{x}\:=−\frac{{t}^{\mathrm{2}} +\mathrm{1}}{{t}^{\mathrm{2}} −\mathrm{1}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com