Question Number 87306 by abdomathmax last updated on 03/Apr/20
$${calculate}\:{by}\:{complex}\:{method}\:\int_{\mathrm{1}} ^{+\infty} \:\frac{{xdx}}{{x}^{\mathrm{4}} \:+\mathrm{1}} \\ $$
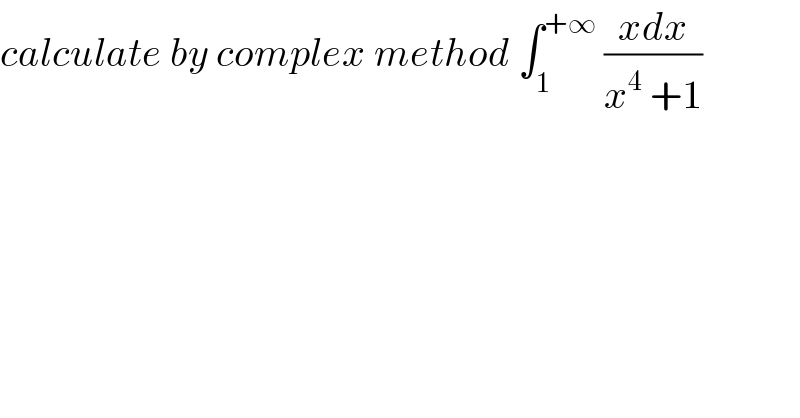
Commented by Ar Brandon last updated on 03/Apr/20
![My suggestion ∫_1 ^(+∞) (x/(x^4 +1))dx=∫_1 ^(+∞) (x/(1+(x^2 )^2 ))dx=(1/2)∫_1 ^(+∞) ((2x)/(1+(x^2 )^2 ))dx =(1/2)[arctan(x^2 )]_1 ^(+∞) =(1/2)((π/2))−(1/2)((π/4))=(π/8)](Q87320.png)
$${My}\:\:{suggestion} \\ $$$$\int_{\mathrm{1}} ^{+\infty} \frac{{x}}{{x}^{\mathrm{4}} +\mathrm{1}}{dx}=\int_{\mathrm{1}} ^{+\infty} \frac{{x}}{\mathrm{1}+\left({x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{2}{x}}{\mathrm{1}+\left({x}^{\mathrm{2}} \right)^{\mathrm{2}} }{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\left[{arctan}\left({x}^{\mathrm{2}} \right)\right]_{\mathrm{1}} ^{+\infty} =\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\pi}{\mathrm{2}}\right)−\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\pi}{\mathrm{4}}\right)=\frac{\pi}{\mathrm{8}} \\ $$
Commented by Ar Brandon last updated on 04/Apr/20
$$\boldsymbol{{By}}\:\:\boldsymbol{{complex}}\:\:\boldsymbol{{method}} \\ $$$${Let}\:\:{x}^{\mathrm{4}} +\mathrm{1}=\mathrm{0}\Rightarrow{x}^{\mathrm{4}} =−\mathrm{1} \\ $$$$\mid{x}^{\mathrm{4}} \mid=\mathrm{1}\:\:{and}\:\:{arg}\left({x}^{\mathrm{4}} \right)=\pi\:{mod}\left(\mathrm{2}\pi\right)\:\Rightarrow\mid{x}\mid=\mathrm{1}\:\:{and}\:\:{arg}\left({x}\right)=\frac{\pi}{\mathrm{4}}{mod}\left(\frac{\pi}{\mathrm{2}}\right) \\ $$$$\Rightarrow{x}_{{k}} ={e}^{\left(\mathrm{2}{k}+\mathrm{1}\right)\frac{\pi}{\mathrm{4}}{i}} \:\:\:\:{k}\in\mathbb{Z} \\ $$$$\Rightarrow{x}^{\mathrm{4}} +\mathrm{1}=\underset{{k}=−\mathrm{2}} {\overset{\mathrm{1}} {\prod}}\left({x}−{x}_{{k}} \right) \\ $$$$\Rightarrow\frac{{x}}{{x}^{\mathrm{4}} +\mathrm{1}}=\frac{{x}}{\underset{{k}=−\mathrm{2}} {\overset{\mathrm{1}} {\prod}}\left({x}−{x}_{{k}} \right)}=\underset{{k}=−\mathrm{2}} {\overset{\mathrm{1}} {\sum}}\frac{{a}_{{k}} }{\left({x}−{x}_{{k}} \right)} \\ $$$${a}_{{k}} =\frac{{x}_{{k}} }{\mathrm{4}{x}_{{k}} ^{\mathrm{3}} }\:\:\:{i}.{e}\:\frac{{P}\left({x}_{{k}} \right)}{{Q}'\left({x}_{{k}} \right)} \\ $$$$\Rightarrow\frac{{x}}{{x}^{\mathrm{4}} +\mathrm{1}}=\underset{{k}=−\mathrm{2}} {\overset{\mathrm{1}} {\sum}}\frac{\mathrm{1}}{\mathrm{4}\left({x}_{{k}} ^{\mathrm{2}} \right)\left({x}−{x}_{{k}} \right)} \\ $$$$\int_{\mathrm{1}} ^{+\infty} \frac{{x}}{{x}^{\mathrm{4}} +\mathrm{1}}{dx}=\int_{\mathrm{1}} ^{+\infty} \underset{{k}=−\mathrm{2}} {\overset{\mathrm{1}} {\sum}}\frac{\mathrm{1}}{\mathrm{4}\left({x}_{{k}} ^{\mathrm{2}} \right)\left({x}−{x}_{{k}} \right)}{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{1}}{\mathrm{4}{i}\left({x}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)}{dx}−\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{1}}{\mathrm{4}{i}\left({x}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)}{dx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{1}}{\mathrm{4}{i}\left({x}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)}{dx}−\int_{\mathrm{1}} ^{+\infty} \frac{\mathrm{1}}{\mathrm{4}{i}\left({x}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\right)}{dx} \\ $$
Commented by Ar Brandon last updated on 04/Apr/20
$$=\frac{{ln}\infty}{\mathrm{4}{i}}−\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right)−\frac{{ln}\infty}{\mathrm{4}{i}}+\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right) \\ $$$$\:\:+\frac{{ln}\infty}{\mathrm{4}{i}}−\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right)−\frac{{ln}\infty}{\mathrm{4}{i}}+\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right)−\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right) \\ $$$$\:\:+\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right)−\frac{\mathrm{1}}{\mathrm{4}{i}}{ln}\left(\mid\mathrm{1}+\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mid\right) \\ $$
Commented by mathmax by abdo last updated on 04/Apr/20
$${thanks}\:{sir} \\ $$
Commented by mathmax by abdo last updated on 04/Apr/20
![let decompose F(x)=(x/(x^4 +1)) poles of F? x^4 +1 =0 ⇔ x^4 =e^((2k+1)π) ⇒ x_k =e^((i(2k+1)π)/4) and k∈[[0,3]] F(x) =Σ_(k.=0) ^3 (a_k /(x−x_k )) with a_(k ) =(x_k /(4x_k ^3 )) =−(1/4)x_k ^2 =−(1/4)e^((i(2k+1)π)/2) =−(1/4) (i)^(2k+1) =−(1/4)(−1)^k i =(i/4)(−1)^k ⇒ ∫_1 ^(+∞) F(x)dx =(i/4) Σ_(k=0) ^3 (−1)^k ∫_1 ^(+∞) (dx/(x−x_k )) we have x_0 =e^((iπ)/4) , x_1 =e^((i3π)/4) , x_2 =e^(i((5π)/4)) =x_1 ^− , x_3 =e^((i7π)/4) =e^(i(((8π−π)/4))) =x_0 ^− ⇒ ∫_1 ^(+∞) F(x)dx =(i/4){ ∫_1 ^(+∞) (dx/(x−x_0 ))−∫_1 ^(+∞) (dx/(x−x_1 )) +∫_1 ^(+∞) (dx/(x−x_1 ^− )) −∫_1 ^(+∞) (dx/(x−x_0 ^− ))} =(i/4)[ln(((x−x_o )/(x−x_1 )))]_1 ^(+∞) +[ln(((x−x_1 ^− )/(x−x_0 ^− )))]_1 ^(+∞) =(i/4)(−ln(((1−x_0 )/(1−x_1 )))−ln(((1−x_1 ^− )/(1−x_0 ^− )))) =(i/4){ ln(((1−x_1 )/(1−x_0 )))+ln(((1−x_0 ^− )/(1−x_1 ^− )))} rest to finish the calculus](Q87460.png)
$${let}\:{decompose}\:{F}\left({x}\right)=\frac{{x}}{{x}^{\mathrm{4}} \:+\mathrm{1}}\:\:{poles}\:{of}\:{F}? \\ $$$${x}^{\mathrm{4}} +\mathrm{1}\:=\mathrm{0}\:\Leftrightarrow\:{x}^{\mathrm{4}} ={e}^{\left(\mathrm{2}{k}+\mathrm{1}\right)\pi} \:\Rightarrow\:{x}_{{k}} ={e}^{\frac{{i}\left(\mathrm{2}{k}+\mathrm{1}\right)\pi}{\mathrm{4}}} \:{and}\:{k}\in\left[\left[\mathrm{0},\mathrm{3}\right]\right] \\ $$$${F}\left({x}\right)\:=\sum_{{k}.=\mathrm{0}} ^{\mathrm{3}} \:\frac{{a}_{{k}} }{{x}−{x}_{{k}} }\:\:{with}\:{a}_{{k}\:} =\frac{{x}_{{k}} }{\mathrm{4}{x}_{{k}} ^{\mathrm{3}} }\:=−\frac{\mathrm{1}}{\mathrm{4}}{x}_{{k}} ^{\mathrm{2}} \:=−\frac{\mathrm{1}}{\mathrm{4}}{e}^{\frac{{i}\left(\mathrm{2}{k}+\mathrm{1}\right)\pi}{\mathrm{2}}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\:\left({i}\right)^{\mathrm{2}{k}+\mathrm{1}} \:=−\frac{\mathrm{1}}{\mathrm{4}}\left(−\mathrm{1}\right)^{{k}} \:{i}\:=\frac{{i}}{\mathrm{4}}\left(−\mathrm{1}\right)^{{k}} \:\Rightarrow \\ $$$$\int_{\mathrm{1}} ^{+\infty} \:{F}\left({x}\right){dx}\:=\frac{{i}}{\mathrm{4}}\:\sum_{{k}=\mathrm{0}} ^{\mathrm{3}} \:\left(−\mathrm{1}\right)^{{k}} \:\int_{\mathrm{1}} ^{+\infty} \:\:\frac{{dx}}{{x}−{x}_{{k}} } \\ $$$${we}\:{have}\:{x}_{\mathrm{0}} ={e}^{\frac{{i}\pi}{\mathrm{4}}} \:\:\:\:,\:{x}_{\mathrm{1}} ={e}^{\frac{{i}\mathrm{3}\pi}{\mathrm{4}}} \:\:,\:{x}_{\mathrm{2}} ={e}^{{i}\frac{\mathrm{5}\pi}{\mathrm{4}}} \:=\overset{−} {{x}}_{\mathrm{1}} \:\:\:,\:{x}_{\mathrm{3}} ={e}^{\frac{{i}\mathrm{7}\pi}{\mathrm{4}}} \:={e}^{{i}\left(\frac{\mathrm{8}\pi−\pi}{\mathrm{4}}\right)} =\overset{−} {{x}}_{\mathrm{0}} \\ $$$$\:\Rightarrow \\ $$$$\int_{\mathrm{1}} ^{+\infty} \:{F}\left({x}\right){dx}\:=\frac{{i}}{\mathrm{4}}\left\{\:\int_{\mathrm{1}} ^{+\infty} \:\frac{{dx}}{{x}−{x}_{\mathrm{0}} }−\int_{\mathrm{1}} ^{+\infty} \:\frac{{dx}}{{x}−{x}_{\mathrm{1}} }\:+\int_{\mathrm{1}} ^{+\infty} \:\frac{{dx}}{{x}−\overset{−} {{x}}_{\mathrm{1}} }\right. \\ $$$$\left.−\int_{\mathrm{1}} ^{+\infty} \:\frac{{dx}}{{x}−\overset{−} {{x}}_{\mathrm{0}} }\right\}\:=\frac{{i}}{\mathrm{4}}\left[{ln}\left(\frac{{x}−{x}_{{o}} }{{x}−{x}_{\mathrm{1}} }\right)\right]_{\mathrm{1}} ^{+\infty} \:+\left[{ln}\left(\frac{{x}−\overset{−} {{x}}_{\mathrm{1}} }{{x}−\overset{−} {{x}}_{\mathrm{0}} }\right)\right]_{\mathrm{1}} ^{+\infty} \\ $$$$=\frac{{i}}{\mathrm{4}}\left(−{ln}\left(\frac{\mathrm{1}−{x}_{\mathrm{0}} }{\mathrm{1}−{x}_{\mathrm{1}} }\right)−{ln}\left(\frac{\mathrm{1}−\overset{−} {{x}}_{\mathrm{1}} }{\mathrm{1}−\overset{−} {{x}}_{\mathrm{0}} }\right)\right)\: \\ $$$$=\frac{{i}}{\mathrm{4}}\left\{\:{ln}\left(\frac{\mathrm{1}−{x}_{\mathrm{1}} }{\mathrm{1}−{x}_{\mathrm{0}} }\right)+{ln}\left(\frac{\mathrm{1}−\overset{−} {{x}}_{\mathrm{0}} }{\mathrm{1}−\overset{−} {{x}}_{\mathrm{1}} }\right)\right\}\:\:{rest}\:{to}\:{finish}\:{the}\:{calculus} \\ $$
Commented by Ar Brandon last updated on 04/Apr/20
$${I}\:{should}\:{be}\:{the}\:{one}\:{thanking}\:{you}.\:{Haha}!\: \\ $$$${I}\:{learned}\:{the}\:{method}\:{from}\:{you}.\:{It}'{s}\:{the}\:{method}\:{you}\: \\ $$$${used}\:{when}\:{integrating}\:\frac{{x}^{\mathrm{6}} }{\mathrm{1}+{x}^{\mathrm{12}} } \\ $$$$ \\ $$
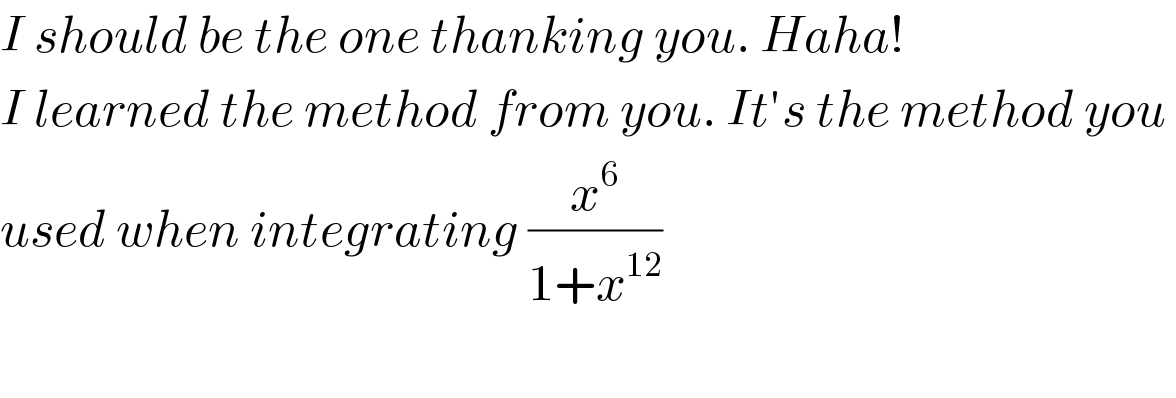
Commented by Ar Brandon last updated on 04/Apr/20
��
Commented by mind is power last updated on 04/Apr/20
$${nice}\:\:\:{the}\:\:{goal}\:{of}\:{this}\:{Forum} \\ $$$${is}\:{to}\:\:{help}\:{and}\:{learnd}\:{new}\:{tricks} \\ $$$${personaly}\:{i}\:{learnd}\:{new}\:\:{ideas}\:{in}\:{this}\:{forum}\: \\ $$$${sorry}\:{for}\:{my}\:{English} \\ $$$$ \\ $$
Commented by Ar Brandon last updated on 05/Apr/20
$${And}\:{I}'{m}\:{doing}\:{same}\:{here}. \\ $$
Answered by mind is power last updated on 04/Apr/20
![∫_0 ^1 ((xdx)/((x^4 +1)))=∫_1 ^(+∞) ((xdx)/((1+x^4 ))) ∫_1 ^(+∞) ((xdx)/(x^4 +1))=(1/2)∫_0 ^∞ ((xdx)/(x^4 +1)) f(z)=(z/(1+z^4 )),let C_R =[0,R]∪{Re^(iθ) ,θ∈[0,(π/2)]}_(=γ) ∪[iR,0];R>1 ∫_C_R f(z)dz=2iπRes(f,z=e^(i(π/4)) )=2iπ.(1/(4(e^(i(π/2)) )))=(π/2) ∫_γ f(z)dz=∫_0 ^(π/2) Rie^(iθ) f(Re^(iθ) )dθ =iR^2 ∫_0 ^(π/2) (e^(2iθ) /(R^4 e^(4iθ) +1))dθ,∣∫f(z)dz∣≤(π/2).(R^2 /(R^4 −1))→0 as R→∞ ∫_(iR) ^0 f(z)dz=i∫_R ^0 f(it)dz=∫_0 ^R (t/(t^4 +1))dt ⇒lim_(R→∞) ∫_C_R f(z)dz=lim_(R→∞) 2∫_0 ^R ((tdt)/(t^4 +1))=(π/2) ∫_0 ^∞ ((tdt)/(t^4 +1)).=(π/4) ∫_1 ^∞ ((xdx)/(x^4 +1))=(π/8) .](Q87331.png)
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{xdx}}{\left({x}^{\mathrm{4}} +\mathrm{1}\right)}=\int_{\mathrm{1}} ^{+\infty} \frac{{xdx}}{\left(\mathrm{1}+{x}^{\mathrm{4}} \right)} \\ $$$$\int_{\mathrm{1}} ^{+\infty} \frac{{xdx}}{{x}^{\mathrm{4}} +\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\infty} \frac{{xdx}}{{x}^{\mathrm{4}} +\mathrm{1}} \\ $$$${f}\left({z}\right)=\frac{{z}}{\mathrm{1}+{z}^{\mathrm{4}} },{let}\:{C}_{{R}} =\left[\mathrm{0},{R}\right]\cup\left\{{Re}^{{i}\theta} ,\theta\in\left[\mathrm{0},\frac{\pi}{\mathrm{2}}\right]\underset{=\gamma} {\right\}}\cup\left[{iR},\mathrm{0}\right];{R}>\mathrm{1} \\ $$$$\int_{{C}_{{R}} } {f}\left({z}\right){dz}=\mathrm{2}{i}\pi{Res}\left({f},{z}={e}^{{i}\frac{\pi}{\mathrm{4}}} \right)=\mathrm{2}{i}\pi.\frac{\mathrm{1}}{\mathrm{4}\left({e}^{{i}\frac{\pi}{\mathrm{2}}} \right)}=\frac{\pi}{\mathrm{2}} \\ $$$$\int_{\gamma} {f}\left({z}\right){dz}=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {Rie}^{{i}\theta} {f}\left({Re}^{{i}\theta} \right){d}\theta \\ $$$$={iR}^{\mathrm{2}} \int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{e}^{\mathrm{2}{i}\theta} }{{R}^{\mathrm{4}} {e}^{\mathrm{4}{i}\theta} +\mathrm{1}}{d}\theta,\mid\int{f}\left({z}\right){dz}\mid\leqslant\frac{\pi}{\mathrm{2}}.\frac{{R}^{\mathrm{2}} }{{R}^{\mathrm{4}} −\mathrm{1}}\rightarrow\mathrm{0}\:{as}\:{R}\rightarrow\infty \\ $$$$\int_{{iR}} ^{\mathrm{0}} {f}\left({z}\right){dz}={i}\int_{{R}} ^{\mathrm{0}} {f}\left({it}\right){dz}=\int_{\mathrm{0}} ^{{R}} \frac{{t}}{{t}^{\mathrm{4}} +\mathrm{1}}{dt} \\ $$$$\Rightarrow\underset{{R}\rightarrow\infty} {\mathrm{lim}}\int_{{C}_{{R}} } {f}\left({z}\right){dz}=\underset{{R}\rightarrow\infty} {\mathrm{lim}2}\int_{\mathrm{0}} ^{{R}} \frac{{tdt}}{{t}^{\mathrm{4}} +\mathrm{1}}=\frac{\pi}{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{tdt}}{{t}^{\mathrm{4}} +\mathrm{1}}.=\frac{\pi}{\mathrm{4}} \\ $$$$\int_{\mathrm{1}} ^{\infty} \frac{{xdx}}{{x}^{\mathrm{4}} +\mathrm{1}}=\frac{\pi}{\mathrm{8}} \\ $$$$ \\ $$$$. \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 04/Apr/20
$${thanks}\:{sir} \\ $$
Commented by mind is power last updated on 04/Apr/20
$${withe}\:{pleasur}\:{sir} \\ $$