Question and Answers Forum
Question Number 87538 by Power last updated on 04/Apr/20

Commented by mathmax by abdo last updated on 04/Apr/20
![I =∫_(−1) ^1 f^(−1) (x)dx changement f^(−1) (x)=t give x =f(t)⇒ dx =f^′ (t)dt ⇒ I =∫_(f^(−1) (−1)) ^(f^(−1) (1)) t f^′ (t)dt =_(by parts) =[(t^2 /2)f(t)]_(f^(−1) (−1)) ^(f^(−1) (1)) −∫_(f^(−1) (−1)) ^(f^(−1) (1)) (t^2 /2)f(t)dt =(1/2){ (f^(−1) (1))^2 + (f^(−1) (−1))^2 }−(1/2)∫_(f^(−1) (−1)) ^(f^(−1) (1)) t^2 (t^3 +t−1)dt we hsve ∫_(f^(−1) (−1)) ^(f^(−1) (1)) (t^5 +t^3 −t^2 )dt [(t^6 /6)+(t^4 /4)−(t^3 /3)]_(f^(−1) (−1)) ^(f^(−1) (1)) rest calculus of f^(−1) (1) and f^(−1) (−1) ...be continued...](Q87541.png)
Commented by Power last updated on 04/Apr/20

Commented by MJS last updated on 04/Apr/20

Commented by mathmax by abdo last updated on 04/Apr/20
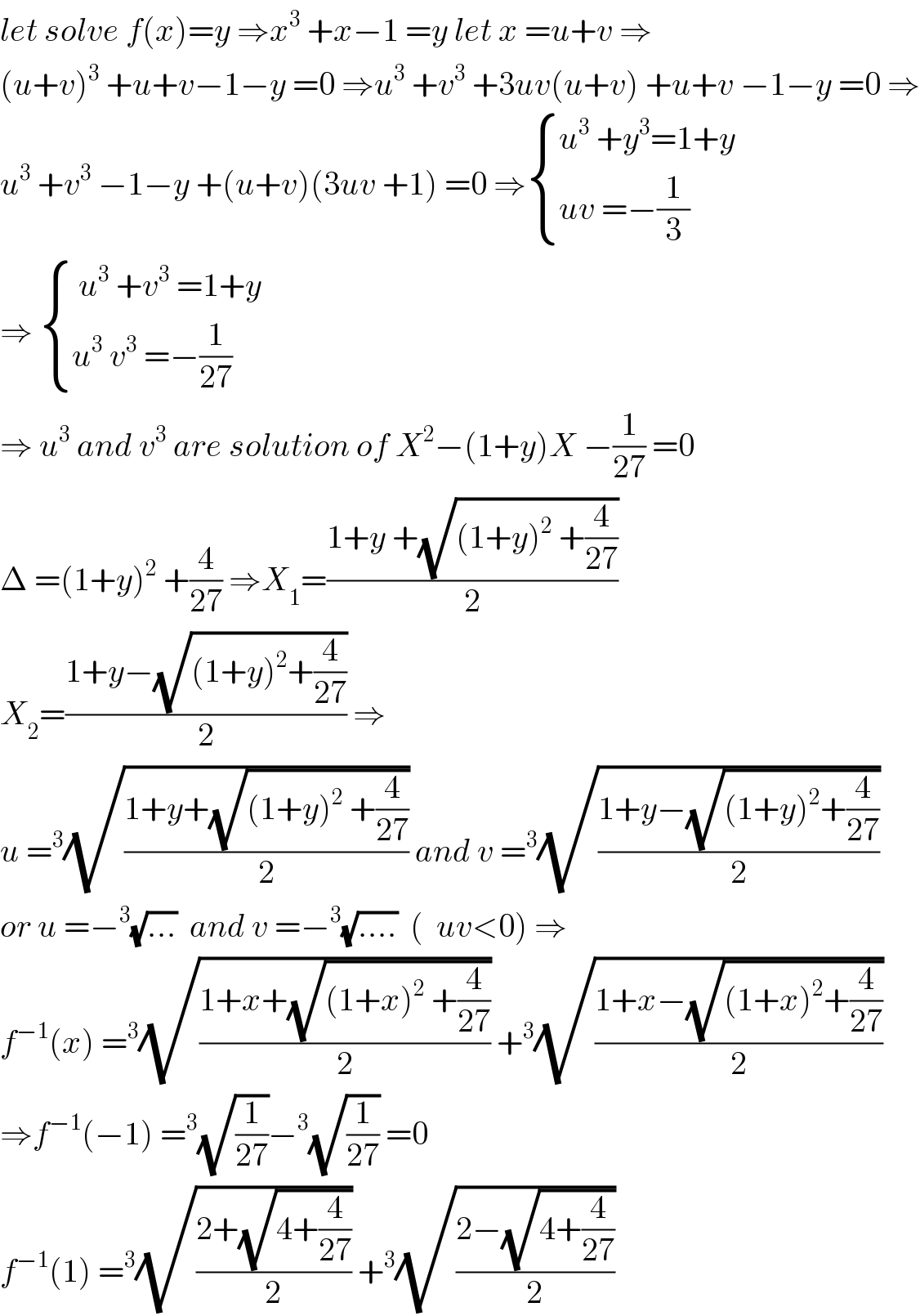
Commented by Power last updated on 05/Apr/20
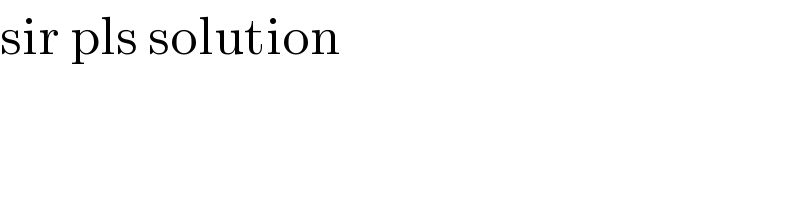
Commented by Power last updated on 05/Apr/20
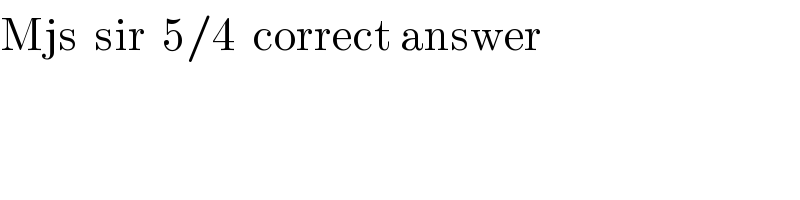
Commented by mathmax by abdo last updated on 05/Apr/20