
Question Number 88415 by abdomathmax last updated on 10/Apr/20
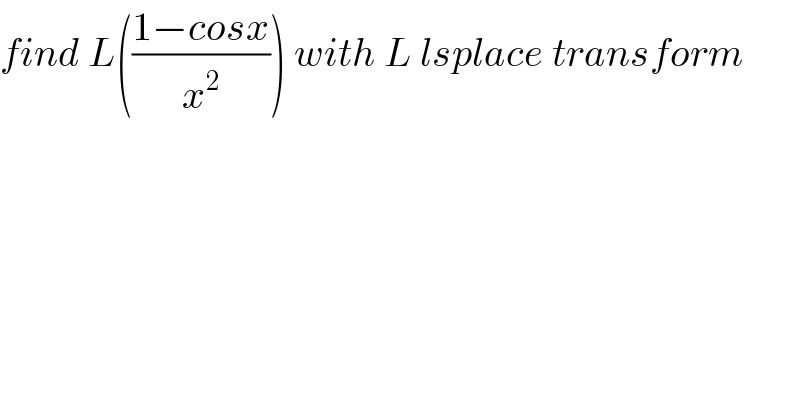
$${find}\:{L}\left(\frac{\mathrm{1}−{cosx}}{{x}^{\mathrm{2}} }\right)\:{with}\:{L}\:{lsplace}\:{transform} \\ $$
Commented by jagoll last updated on 10/Apr/20
![L(1−cos x) = 1−(x/(x^2 +1)) = ((x^2 −x+1)/(x^2 +1)) L(((1−cos x)/x)) = (d/dx) [((x^2 −x+1)/(x^2 +1))] = (((2x−1)(x^2 +1)−2x(x^2 −x+1))/((x^2 +1)^2 )) = ((2x^3 −x^2 +2x−1−2x^3 +2x^2 −2x)/((x^2 +1)^2 )) = ((x^2 −1)/((x^2 +1)^2 )) L(((1−cos x)/x^2 )) = (d/dx)[((x^2 −1)/((x^2 +1)^2 ))] = ((2x(x^2 +1)^2 −4x(x^2 +1)(x^2 −1))/((x^2 +1)^4 )) = ((2x(x^2 +1){x^2 +1−2(x^2 −1)})/((x^2 +1)^4 )) = ((2x(−x^2 +3))/((x^2 +1)^3 )) = ((−2x^3 +6x)/((x^2 +1)^3 ))](Q88428.png)
$$\mathscr{L}\left(\mathrm{1}−\mathrm{cos}\:\mathrm{x}\right)\:=\:\mathrm{1}−\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\:=\:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathscr{L}\left(\frac{\mathrm{1}−\mathrm{cos}\:\mathrm{x}}{\mathrm{x}}\right)\:=\:\frac{\mathrm{d}}{\mathrm{dx}}\:\left[\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\right] \\ $$$$=\:\frac{\left(\mathrm{2x}−\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)−\mathrm{2x}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{2x}^{\mathrm{3}} −\mathrm{x}^{\mathrm{2}} +\mathrm{2x}−\mathrm{1}−\mathrm{2x}^{\mathrm{3}} +\mathrm{2x}^{\mathrm{2}} −\mathrm{2x}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\mathscr{L}\left(\frac{\mathrm{1}−\mathrm{cos}\:\mathrm{x}}{\mathrm{x}^{\mathrm{2}} }\right)\:=\:\frac{\mathrm{d}}{\mathrm{dx}}\left[\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }\right] \\ $$$$=\:\frac{\mathrm{2x}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} −\mathrm{4x}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{4}} } \\ $$$$=\:\frac{\mathrm{2x}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)\left\{\mathrm{x}^{\mathrm{2}} +\mathrm{1}−\mathrm{2}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{1}\right)\right\}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{4}} } \\ $$$$=\:\frac{\mathrm{2x}\left(−\mathrm{x}^{\mathrm{2}} +\mathrm{3}\right)}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} }\:=\:\frac{−\mathrm{2x}^{\mathrm{3}} +\mathrm{6x}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{3}} } \\ $$
Commented by mathmax by abdo last updated on 10/Apr/20
![L(((1−cosx)/x^2 )) =∫_0 ^∞ f(t)e^(−xt) dt =∫_0 ^∞ ((1−cost)/t^2 ) e^(−xt) dt (x>0) =ϕ(x) ⇒ϕ^′ (x)=−∫_0 ^∞ ((1−cost)/t) e^(−xt) dt ⇒ ϕ^(′′) (x) =∫_0 ^∞ (1−cost)e^(−xt ) dt =∫_0 ^∞ e^(−xt) dt −∫_0 ^∞ e^(−xt) cost dt =[−(1/x)e^(−xt) ]_0 ^(+∞) −Re(∫_0 ^∞ e^(−xt+it) dt) =(1/x) −Re(∫_0 ^∞ e^((−x+i)t) dt) ∫_0 ^∞ e^((−x+i)t) dt =[(1/(−x+i))e^((−x+i)t) ]_0 ^(+∞) =((−1)/(−x+i)) =(1/(x−i)) =((x+i)/(x^2 +1)) ⇒ ϕ^(′′) (x)=(1/x)−(x/(x^2 +1)) ⇒ϕ^′ (x)=lnx −(1/2)ln(x^2 +1) +λ ⇒ ϕ(x)=xlnx−x −(1/2)∫ ln(x^2 +1)dx +λx +α ∫ ln(x^2 +1)dx =x ln(x^2 +1)−∫ x×((2x)/(x^2 +1))dx =xln(x^2 +1)−2 ∫ ((x^2 +1−1)/(x^2 +1))dx =xln(x^2 +1)−2x +2arctanx +c⇒ ϕ(x) =xlnx −x −(x/2)ln(x^2 +1) +x −arctanx +λx +c ⇒ϕ(x)=xlnx −(x/2)ln(x^2 +1)−arctanx +λx +c lim_(x→0) ϕ(x) =∫_0 ^∞ ((1−cost)/t^2 )dt =_(by parts) [−(1/t)(1−cost)]_0 ^∞ +∫_0 ^∞ (1/t)sint dt =(π/2) =c ⇒ ϕ(x)=xlnx −(x/2)ln(x^2 +1)−arctanx +λx +(π/2) rest to find λ .be continued...](Q88478.png)
$${L}\left(\frac{\mathrm{1}−{cosx}}{{x}^{\mathrm{2}} }\right)\:=\int_{\mathrm{0}} ^{\infty} \:\:{f}\left({t}\right){e}^{−{xt}} \:{dt}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}−{cost}}{{t}^{\mathrm{2}} }\:{e}^{−{xt}} \:{dt}\:\:\:\:\:\:\:\left({x}>\mathrm{0}\right) \\ $$$$=\varphi\left({x}\right)\:\Rightarrow\varphi^{'} \left({x}\right)=−\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{1}−{cost}}{{t}}\:{e}^{−{xt}} \:{dt}\:\Rightarrow \\ $$$$\varphi^{''} \left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \left(\mathrm{1}−{cost}\right){e}^{−{xt}\:} {dt}\:\:=\int_{\mathrm{0}} ^{\infty} \:{e}^{−{xt}} \:{dt}\:−\int_{\mathrm{0}} ^{\infty} \:{e}^{−{xt}} \:{cost}\:{dt} \\ $$$$=\left[−\frac{\mathrm{1}}{{x}}{e}^{−{xt}} \right]_{\mathrm{0}} ^{+\infty} \:−{Re}\left(\int_{\mathrm{0}} ^{\infty} \:{e}^{−{xt}+{it}} \:{dt}\right) \\ $$$$=\frac{\mathrm{1}}{{x}}\:−{Re}\left(\int_{\mathrm{0}} ^{\infty} \:{e}^{\left(−{x}+{i}\right){t}} \:{dt}\right) \\ $$$$\int_{\mathrm{0}} ^{\infty} \:{e}^{\left(−{x}+{i}\right){t}} {dt}\:=\left[\frac{\mathrm{1}}{−{x}+{i}}{e}^{\left(−{x}+{i}\right){t}} \right]_{\mathrm{0}} ^{+\infty} =\frac{−\mathrm{1}}{−{x}+{i}}\:=\frac{\mathrm{1}}{{x}−{i}}\:=\frac{{x}+{i}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$$\varphi^{''} \left({x}\right)=\frac{\mathrm{1}}{{x}}−\frac{{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow\varphi^{'} \left({x}\right)={lnx}\:−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\:+\lambda\:\Rightarrow \\ $$$$\varphi\left({x}\right)={xlnx}−{x}\:−\frac{\mathrm{1}}{\mathrm{2}}\int\:{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right){dx}\:+\lambda{x}\:+\alpha \\ $$$$\int\:{ln}\left({x}^{\mathrm{2}} +\mathrm{1}\right){dx}\:={x}\:{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)−\int\:{x}×\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx}\: \\ $$$$={xln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)−\mathrm{2}\:\int\:\:\frac{{x}^{\mathrm{2}} \:+\mathrm{1}−\mathrm{1}}{{x}^{\mathrm{2}} \:+\mathrm{1}}{dx} \\ $$$$={xln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)−\mathrm{2}{x}\:+\mathrm{2}{arctanx}\:+{c}\Rightarrow \\ $$$$\varphi\left({x}\right)\:={xlnx}\:−{x}\:−\frac{{x}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)\:+{x}\:−{arctanx}\:+\lambda{x}\:+{c} \\ $$$$\Rightarrow\varphi\left({x}\right)={xlnx}\:−\frac{{x}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)−{arctanx}\:+\lambda{x}\:+{c} \\ $$$${lim}_{{x}\rightarrow\mathrm{0}} \varphi\left({x}\right)\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}−{cost}}{{t}^{\mathrm{2}} }{dt}\:\:=_{{by}\:{parts}} \:\:\left[−\frac{\mathrm{1}}{{t}}\left(\mathrm{1}−{cost}\right)\right]_{\mathrm{0}} ^{\infty} \\ $$$$+\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{1}}{{t}}{sint}\:{dt}\:=\frac{\pi}{\mathrm{2}}\:={c}\:\Rightarrow \\ $$$$\varphi\left({x}\right)={xlnx}\:−\frac{{x}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)−{arctanx}\:+\lambda{x}\:+\frac{\pi}{\mathrm{2}} \\ $$$${rest}\:{to}\:{find}\:\lambda\:\:\:.{be}\:{continued}... \\ $$
