Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 88438 by M±th+et£s last updated on 10/Apr/20

∫((x^5 +1)/(x^5 −1))dx

$$\int\frac{{x}^{\mathrm{5}} +\mathrm{1}}{{x}^{\mathrm{5}} −\mathrm{1}}{dx} \\ $$

Answered by Kunal12588 last updated on 10/Apr/20

I=∫((x^5 −1)/(x^5 −1))dx+2∫(dx/(x^5 −1)) =x+2∫(dx/((x−1)(x^4 +x^3 +x^2 +x+1))) 2=a(x^4 +x^3 +x^2 +x+1)+(bx^3 +cx^2 +dx+e)(x−1) a=(2/5), b=((−2)/5),c=((−4)/5),d=((−6)/5)e=((−8)/5) I=x+(2/5)ln∣x−1∣−(2/5)∫((x^3 +2x^2 +3x+4)/(x^4 +x^3 +x^2 +x+1))dx I=x+(2/5)ln∣x−1∣−(2/5)ln∣x^4 +x^3 +x^2 +x+1∣−(2/5)∫(((x−1)(3x^2 +4x+1))/(x^4 +x^3 +x^2 +x+1))dx i don′t think this is any easier

$${I}=\int\frac{{x}^{\mathrm{5}} −\mathrm{1}}{{x}^{\mathrm{5}} −\mathrm{1}}{dx}+\mathrm{2}\int\frac{{dx}}{{x}^{\mathrm{5}} −\mathrm{1}} \\ $$$$={x}+\mathrm{2}\int\frac{{dx}}{\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}\right)} \\ $$$$\mathrm{2}={a}\left({x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}\right)+\left({bx}^{\mathrm{3}} +{cx}^{\mathrm{2}} +{dx}+{e}\right)\left({x}−\mathrm{1}\right) \\ $$$${a}=\frac{\mathrm{2}}{\mathrm{5}},\:{b}=\frac{−\mathrm{2}}{\mathrm{5}},{c}=\frac{−\mathrm{4}}{\mathrm{5}},{d}=\frac{−\mathrm{6}}{\mathrm{5}}{e}=\frac{−\mathrm{8}}{\mathrm{5}} \\ $$$${I}={x}+\frac{\mathrm{2}}{\mathrm{5}}{ln}\mid{x}−\mathrm{1}\mid−\frac{\mathrm{2}}{\mathrm{5}}\int\frac{{x}^{\mathrm{3}} +\mathrm{2}{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{4}}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$$${I}={x}+\frac{\mathrm{2}}{\mathrm{5}}{ln}\mid{x}−\mathrm{1}\mid−\frac{\mathrm{2}}{\mathrm{5}}{ln}\mid{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}\mid−\frac{\mathrm{2}}{\mathrm{5}}\int\frac{\left({x}−\mathrm{1}\right)\left(\mathrm{3}{x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{1}\right)}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}}{dx} \\ $$$${i}\:{don}'{t}\:{think}\:{this}\:{is}\:{any}\:{easier} \\ $$

Answered by MJS last updated on 10/Apr/20

x^5 −1=(x−1)(x^2 +((1−(√5))/2)x+1)(x^2 +((1+(√5))/2)x+1) ⇒ ((x^5 +1)/(x^5 −1))=1+(2/(5(x−1)))−(((1−(√5))(x−1−(√5)))/(5(x^2 +((1−(√5))/2)x+1)))−(((1+(√5))(x−1+(√5)))/(5(x^2 +((1+(√5))/2)x+1))) ∫((x^5 +1)/(x^5 −1))dx= =∫dx+ +(2/5)∫(dx/(x−1))− −((1−(√5))/5)∫((x−1−(√5))/(x^2 +((1−(√5))/2)x+1))dx− −((1+(√5))/5)∫((x−1+(√5))/(x^2 +((1+(√5))/2)x+1))dx= =x+ +(2/5)ln (x−1) − −((1−(√5))/(10))ln (x^2 +((1−(√5))/2)x+1) −((√(10+2(√5)))/5)arctan (((√(50−10(√5)))(4x+1−(√5)))/(20)) − −((1+(√5))/(10))ln (x^2 +((1+(√5))/2)x+1) −((√(10−2(√5)))/5)arctan (((√(50+10(√5)))(4x+1+(√5)))/(20)) + +C

$${x}^{\mathrm{5}} −\mathrm{1}=\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right) \\ $$$$\Rightarrow \\ $$$$\frac{{x}^{\mathrm{5}} +\mathrm{1}}{{x}^{\mathrm{5}} −\mathrm{1}}=\mathrm{1}+\frac{\mathrm{2}}{\mathrm{5}\left({x}−\mathrm{1}\right)}−\frac{\left(\mathrm{1}−\sqrt{\mathrm{5}}\right)\left({x}−\mathrm{1}−\sqrt{\mathrm{5}}\right)}{\mathrm{5}\left({x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)}−\frac{\left(\mathrm{1}+\sqrt{\mathrm{5}}\right)\left({x}−\mathrm{1}+\sqrt{\mathrm{5}}\right)}{\mathrm{5}\left({x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)} \\ $$$$\int\frac{{x}^{\mathrm{5}} +\mathrm{1}}{{x}^{\mathrm{5}} −\mathrm{1}}{dx}= \\ $$$$=\int{dx}+ \\ $$$$\:\:\:\:\:+\frac{\mathrm{2}}{\mathrm{5}}\int\frac{{dx}}{{x}−\mathrm{1}}− \\ $$$$\:\:\:\:\:−\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{5}}\int\frac{{x}−\mathrm{1}−\sqrt{\mathrm{5}}}{{x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx}− \\ $$$$\:\:\:\:\:−\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{5}}\int\frac{{x}−\mathrm{1}+\sqrt{\mathrm{5}}}{{x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx}= \\ $$$$={x}+ \\ $$$$\:\:\:\:\:+\frac{\mathrm{2}}{\mathrm{5}}\mathrm{ln}\:\left({x}−\mathrm{1}\right)\:− \\ $$$$\:\:\:\:\:−\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{10}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\:−\frac{\sqrt{\mathrm{10}+\mathrm{2}\sqrt{\mathrm{5}}}}{\mathrm{5}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{50}−\mathrm{10}\sqrt{\mathrm{5}}}\left(\mathrm{4}{x}+\mathrm{1}−\sqrt{\mathrm{5}}\right)}{\mathrm{20}}\:− \\ $$$$\:\:\:\:\:−\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{10}}\mathrm{ln}\:\left({x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\:−\frac{\sqrt{\mathrm{10}−\mathrm{2}\sqrt{\mathrm{5}}}}{\mathrm{5}}\mathrm{arctan}\:\frac{\sqrt{\mathrm{50}+\mathrm{10}\sqrt{\mathrm{5}}}\left(\mathrm{4}{x}+\mathrm{1}+\sqrt{\mathrm{5}}\right)}{\mathrm{20}}\:+ \\ $$$$\:\:\:\:\:+{C} \\ $$

Commented by MJS last updated on 10/Apr/20

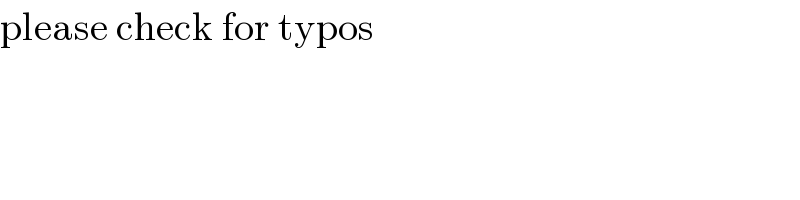
please check for typos

$$\mathrm{please}\:\mathrm{check}\:\mathrm{for}\:\mathrm{typos} \\ $$

Commented by M±th+et£s last updated on 10/Apr/20

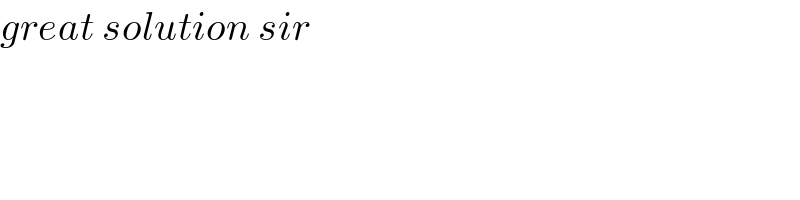
great solution sir

$${great}\:{solution}\:{sir} \\ $$

Commented by Ar Brandon last updated on 10/Apr/20

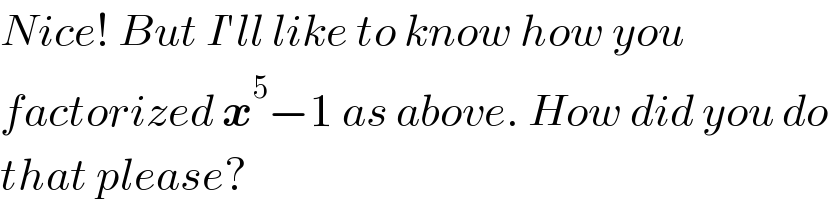
Nice! But I′ll like to know how you factorized x^5 −1 as above. How did you do that please?

$${Nice}!\:{But}\:{I}'{ll}\:{like}\:{to}\:{know}\:{how}\:{you} \\ $$$${factorized}\:\boldsymbol{{x}}^{\mathrm{5}} −\mathrm{1}\:{as}\:{above}.\:{How}\:{did}\:{you}\:{do} \\ $$$${that}\:{please}? \\ $$

Commented by MJS last updated on 11/Apr/20

generally factorizing x^4 +ax^3 +bx^2 +cx+d=0 first let x=t−(a/4), this leads to t^4 +pt^2 +qt+r=0 now we try to get 2 square factors (t^2 +αt+β)(t^2 −αt+γ)=t^4 +pt^2 +qt+r this can be solved by matching the factors solve 2 of the 3 equations for β and γ this leads to a solveable equation for α if we have luck, we get a useable “nice” solution... but in the case of x^5 =1 we can use the complex solutions x_(k+1) =e^(i((2πk)/5)) ; 0≤k≤4

$$\mathrm{generally}\:\mathrm{factorizing} \\ $$$${x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +{cx}+{d}=\mathrm{0} \\ $$$$\mathrm{first}\:\mathrm{let}\:{x}={t}−\frac{{a}}{\mathrm{4}},\:\mathrm{this}\:\mathrm{leads}\:\mathrm{to} \\ $$$${t}^{\mathrm{4}} +{pt}^{\mathrm{2}} +{qt}+{r}=\mathrm{0} \\ $$$$\mathrm{now}\:\mathrm{we}\:\mathrm{try}\:\mathrm{to}\:\mathrm{get}\:\mathrm{2}\:\mathrm{square}\:\mathrm{factors} \\ $$$$\left({t}^{\mathrm{2}} +\alpha{t}+\beta\right)\left({t}^{\mathrm{2}} −\alpha{t}+\gamma\right)={t}^{\mathrm{4}} +{pt}^{\mathrm{2}} +{qt}+{r} \\ $$$$\mathrm{this}\:\mathrm{can}\:\mathrm{be}\:\mathrm{solved}\:\mathrm{by}\:\mathrm{matching}\:\mathrm{the}\:\mathrm{factors} \\ $$$$\mathrm{solve}\:\mathrm{2}\:\mathrm{of}\:\mathrm{the}\:\mathrm{3}\:\mathrm{equations}\:\mathrm{for}\:\beta\:\mathrm{and}\:\gamma \\ $$$$\mathrm{this}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{a}\:\mathrm{solveable}\:\mathrm{equation}\:\mathrm{for}\:\alpha \\ $$$$\mathrm{if}\:\mathrm{we}\:\mathrm{have}\:\mathrm{luck},\:\mathrm{we}\:\mathrm{get}\:\mathrm{a}\:\mathrm{useable}\:``\mathrm{nice}'' \\ $$$$\mathrm{solution}... \\ $$$$\mathrm{but}\:\mathrm{in}\:\mathrm{the}\:\mathrm{case}\:\mathrm{of}\:{x}^{\mathrm{5}} =\mathrm{1}\:\mathrm{we}\:\mathrm{can}\:\mathrm{use}\:\mathrm{the} \\ $$$$\mathrm{complex}\:\mathrm{solutions} \\ $$$${x}_{{k}+\mathrm{1}} =\mathrm{e}^{\mathrm{i}\frac{\mathrm{2}\pi{k}}{\mathrm{5}}} ;\:\mathrm{0}\leqslant{k}\leqslant\mathrm{4} \\ $$

Commented by Ar Brandon last updated on 11/Apr/20

Oh thanks, but I′m more familiar with the complex solution. Just that in this case we get ((4π)/5) and ((2π)/5) and It′s difficult to simplify using identical angles.

$${Oh}\:{thanks},\:{but}\:{I}'{m}\:{more}\:{familiar}\:{with} \\ $$$${the}\:{complex}\:{solution}.\:{Just}\:{that}\:{in}\:{this}\:{case} \\ $$$${we}\:{get}\:\frac{\mathrm{4}\pi}{\mathrm{5}}\:{and}\:\frac{\mathrm{2}\pi}{\mathrm{5}}\:{and}\:{It}'{s}\:{difficult}\:{to}\:{simplify} \\ $$$${using}\:{identical}\:{angles}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com