Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 88490 by M±th+et£s last updated on 11/Apr/20

$${\int }_1^{\mathrm{\infty }}\frac{x^4}{4^x}dx=?$$

Commented by jagoll last updated on 11/Apr/20

Commented by Ar Brandon last updated on 11/Apr/20

$$cool!$$

Commented by M±th+et£s last updated on 11/Apr/20

$$thanxsir$$

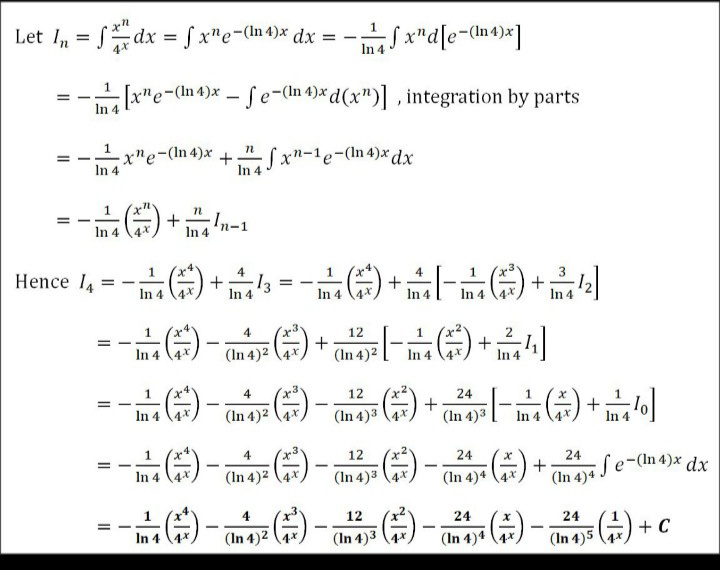
Commented by mathmax by abdo last updated on 11/Apr/20

$$I={\int }_1^{\mathrm{\infty }}\frac{x^4}{4^x}dx\Rightarrow I={\int }_1^{+\mathrm{\infty }}{x}^4{4}^{-x}dxwedothechangement$$$$4^{-x}=t\Rightarrow e^{-xln\left(4\right)}=t\Rightarrow -xln\left(4\right)=ln\left(t\right)\Rightarrow x=-\frac{ln\left(t\right)}{2ln\left(2\right)}\Rightarrow$$$$I=-\frac{1}{2ln2}{\int }_{\frac{1}{4}}^0\frac{{\left(lnt\right)}^4}{4{ln}^4\left(2\right)}\times t\times \frac{dt}{t}$$$$=\frac{1}{8{ln}^5\left(2\right)}{\int }_0^{\frac{1}{4}}{ln}^4\left(t\right)dtlet{u}_n=\int {ln}^n\left(t\right)dtbyparts$$$$u_n=t{ln}^n\left(t\right)-\int tn{\left(lnt\right)}^{n-1}\frac{dt}{t}=t{ln}^n\left(t\right)-n{u}_{n-1}$$$$\Rightarrow u_4=t{ln}^4\left(t\right)-4u_3=t{ln}^4\left(t\right)-4(t{ln}^3\left(t\right)-3u_2)$$$$=t{ln}^4\left(t\right)-4t{ln}^3\left(t\right)+12(t{ln}^2\left(t\right)-2u_1)$$$$=t{ln}^4\left(t\right)-4t{ln}^3\left(t\right)+12t{ln}^2\left(t\right)-24(tlnt-t)\Rightarrow$$$${\int }_0^{\frac{1}{4}}{ln}^4\left(t\right)dt={[t{ln}^4\left(t\right)-4t{ln}^3\left(t\right)+12{tln}^2\left(t\right)-24tln\left(t\right)+24t]}_0^{\frac{1}{4}}$$$$resttofinishthecalculus...$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com