∫_(−(√3) ) ^(√3) ∫_1 ^(√(4−x^2 )) (x^2 +y^2 )^(3/2) dydx


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 88710 by jagoll last updated on 12/Apr/20

$\underset{- \sqrt{3}}{\int^{\sqrt{3}}} \underset{1}{\int^{\sqrt{4 - x^{2}}}} {(x^{2} + y^{2})}^{3 / 2} d y d x$
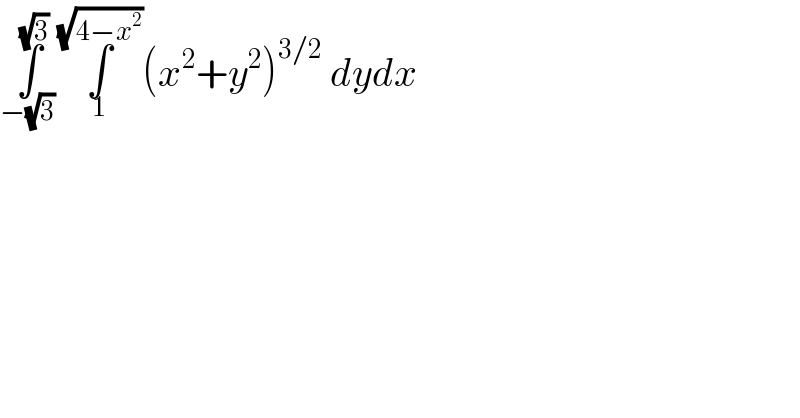
Commented by mathmax by abdo last updated on 12/Apr/20
$I =∫_(−(√3)) ^(√3) (∫_1 ^(√(4−x^2 )) (x^2 +y^2 )^(3/2) dy)dx =∫_(−(√3)) ^(√3) A(x)dx A(x) =∫_1 ^(√(4−x^2 )) (y^2 +x^2 )^(3/2) dy =_(y=∣x∣sh(t)) ∫_(argsh((1/(∣x∣)))) ^(argsh(((√(4−x^2 ))/(∣x∣)))) x^3 (ch^2 t)^(3/2) ∣x∣ch(t)dt =∫_(ln((1/(∣x∣))+(√(1+(1/x^2 ))))) ^(ln(((√(4−x^2 ))/(∣x∣))+(√(1+((4−x^2 )/x^2 ))))) x^3 ∣x∣ ch^4 t dt =x^3 ∣x∣ ∫_(α(x)) ^(β(x)) (((1+ch(2t))/2))^2 dt =(1/4)x^3 ∣x∣∫_(α(x)) ^(β(x)) (1+2ch(2t)+ch^2 (2t))dt =(1/4)x^3 ∣x∣(β(x)−α(x))+(1/4)[sh(2t)]_(α(x)) ^(β(x)) +(1/4)x^3 ∣x∣ ∫_(α(x)) ^(β(x)) (((1+ch(4t))/2))dt =(1/4)x^3 ∣x∣(β(x)−α(x))+(1/4)[((e^(2t) −e^(−2t) )/2)]_(α(x)) ^(β(x)) +(1/8)x^3 ∣x∣(β(x)−α(x)) +(1/(32))x^3 ∣x∣ [sh(4t)]_(α(x)) ^(β(x)) =(3/8)x^3 ∣x∣(β(x)−α(x))+(1/8){e^(2β(x)) −e^(−2α(x)) −e^(2α(x)) +e^(−2α(x)) } +(1/(64))x^3 ∣x∣{e^(4β(x)) −e^(−4β(x)) −e^(4α(x)) +e^(−4α(x)) }....be continued...$
$I = \int_{- \sqrt{3}}^{\sqrt{3}} (\int_{1}^{\sqrt{4 - x^{2}}} {(x^{2} + y^{2})}^{\frac{3}{2}} d y) d x = \int_{- \sqrt{3}}^{\sqrt{3}} A (x) d x$ $A (x) = \int_{1}^{\sqrt{4 - x^{2}}} {(y^{2} + x^{2})}^{\frac{3}{2}} d y =_{y =∣ x ∣ s h (t)} \int_{a r g s h (\frac{1}{∣ x ∣})}^{a r g s h (\frac{\sqrt{4 - x^{2}}}{∣ x ∣})} x^{3} {({c h}^{2} t)}^{\frac{3}{2}} ∣ x ∣ c h (t) d t$ $= \int_{l n (\frac{1}{∣ x ∣} + \sqrt{1 + \frac{1}{x^{2}}})}^{l n (\frac{\sqrt{4 - x^{2}}}{∣ x ∣} + \sqrt{1 + \frac{4 - x^{2}}{x^{2}}})} x^{3} ∣ x ∣ {c h}^{4} t d t$ $= x^{3} ∣ x ∣ \int_{α (x)}^{β (x)} {(\frac{1 + c h (2 t)}{2})}^{2} d t = \frac{1}{4} x^{3} ∣ x ∣ \int_{α (x)}^{β (x)} (1 + 2 c h (2 t) + {c h}^{2} (2 t)) d t$ $= \frac{1}{4} x^{3} ∣ x ∣ (β (x) - α (x)) + \frac{1}{4} {[s h (2 t)]}_{α (x)}^{β (x)} + \frac{1}{4} x^{3} ∣ x ∣ \int_{α (x)}^{β (x)} (\frac{1 + c h (4 t)}{2}) d t$ $= \frac{1}{4} x^{3} ∣ x ∣ (β (x) - α (x)) + \frac{1}{4} {[\frac{e^{2 t} - e^{- 2 t}}{2}]}_{α (x)}^{β (x)} + \frac{1}{8} x^{3} ∣ x ∣ (β (x) - α (x))$ $+ \frac{1}{32} x^{3} ∣ x ∣ {[s h (4 t)]}_{α (x)}^{β (x)}$ $= \frac{3}{8} x^{3} ∣ x ∣ (β (x) - α (x)) + \frac{1}{8} {e^{2 β (x)} - e^{- 2 α (x)} - e^{2 α (x)} + e^{- 2 α (x)}}$ $+ \frac{1}{64} x^{3} ∣ x ∣ {e^{4 β (x)} - e^{- 4 β (x)} - e^{4 α (x)} + e^{- 4 α (x)}} . . . . b e c o n t i n u e d . . .$
	Answered by john santu last updated on 12/Apr/20
	$let me try. to converting to polar coordinates y =(√(4−x^2 )) ⇒ { ((y=1)),((r=2 , θ = (π/6), ((5π)/6))) :} I = ∫_(π/6) ^((5π)/6) ∫_(csc θ) ^2 (r^2 )^(3/2) (r dr dθ) I = ∫_(π/6) ^((5π)/6) ∫_(csc θ) ^2 r^4 dr dθ I = ∫_(π/6) ^((5π)/6) {(1/5)r^5 ]_(csc θ) ^2 } dθ I = (1/5)∫_(π/6) ^((5π)/6) (32−csc^5 θ ) dθ I = (1/5)(((64π)/3) −∫_(π/6) ^((5π)/6) csc^5 θ dθ) I = ((64π)/(15)) −(1/5) [−(1/4) csc^3 θ cot θ − (3/8)csc θ cot θ −(3/8) ln ∣ csc θ + cot θ ∣ ]^((5π)/6) _( (π/6)) I = (1/(60)) ( 256π −9 ln (2+(√3) )−66(√3) )$
	$l e t m e t r y .$ $t o c o n v e r t i n g t o p o l a r c o o r d i n a t e s$ $y = \sqrt{4 - x^{2}} \Rightarrow {\begin{cases} y = 1 \\ r = 2, θ = \frac{π}{6}, \frac{5 π}{6} \end{cases}$ $I = \underset{\frac{π}{6}}{\int^{\frac{5 π}{6}}} \underset{c s c θ}{\int^{2}} {(r^{2})}^{\frac{3}{2}} (r d r d θ)$ $I = \underset{\frac{π}{6}}{\int^{\frac{5 π}{6}}} \underset{c s c θ}{\int^{2}} r^{4} d r d θ$ $I = \underset{\frac{π}{6}}{\int^{\frac{5 π}{6}}} {{\frac{1}{5} r^{5}]}_{c s c θ}^{2}} d θ$ $I = \frac{1}{5} \underset{\frac{π}{6}}{\int^{\frac{5 π}{6}}} (32 - c s c^{5} θ) d θ$ $I = \frac{1}{5} (\frac{64 π}{3} - \underset{\frac{π}{6}}{\int^{\frac{5 π}{6}}} c s c^{5} θ d θ)$ $I = \frac{64 π}{15} - \frac{1}{5} [- \frac{1}{4} c s c^{3} θ \cot θ$ $Missing \left or extra \right$ $I = \frac{1}{60} (256 π - 9 \ln (2 + \sqrt{3}) - 66 \sqrt{3})$
	Commented by jagoll last updated on 12/Apr/20

	$t h a n k y o u s i r .$
	Answered by mr W last updated on 12/Apr/20

	$I = \underset{- \sqrt{3}}{\int^{\sqrt{3}}} \underset{1}{\int^{\sqrt{4 - x^{2}}}} {(x^{2} + y^{2})}^{3 / 2} d y d x$ $= 2 \underset{0}{\int^{\sqrt{3}}} \underset{1}{\int^{\sqrt{4 - x^{2}}}} {(x^{2} + y^{2})}^{3 / 2} d y d x$ $= 2 \int_{\frac{π}{6}}^{\frac{π}{2}} \int_{\frac{1}{\sin θ}}^{2} r^{4} d r d θ$ $= \frac{2}{5} \int_{\frac{π}{6}}^{\frac{π}{2}} (2^{5} - \frac{1}{\sin^{5} θ}) d θ$ $= \frac{2}{5} [32 (\frac{π}{2} - \frac{π}{6}) - \int_{\frac{π}{6}}^{\frac{π}{2}} \frac{d θ}{\sin^{5} θ}]$ $= \frac{2}{5} [\frac{32 π}{3} - \int_{\frac{π}{6}}^{\frac{π}{2}} \frac{d θ}{\sin^{5} θ}]$ $= \frac{2}{5} [\frac{32 π}{3} - \frac{11 \sqrt{3}}{4} - \frac{3 \ln (2 + \sqrt{3})}{8}]$ $= \frac{1}{60} [256 π - 66 \sqrt{3} - 9 \ln (2 + \sqrt{3})]$ $\approx 11.301$ $\int_{\frac{π}{6}}^{\frac{π}{2}} \frac{d θ}{\sin^{5} θ}$ $= \int_{\frac{π}{6}}^{\frac{π}{2}} \frac{\sin θ d θ}{\sin^{6} θ}$ $= - \int_{\frac{π}{6}}^{\frac{π}{2}} \frac{d (\cos θ)}{{(1 - \cos^{2} θ)}^{3}}$ $= \int_{0}^{\frac{\sqrt{3}}{2}} \frac{d u}{{(1 - u^{2})}^{3}}$ $. . . . . .$ $= \frac{3}{8} \ln (2 + \sqrt{3}) + \frac{11 \sqrt{3}}{4}$
	Commented by mr W last updated on 12/Apr/20

	${\begin{cases} θ = \frac{π}{6} \\ θ = \frac{5 π}{6} \end{cases}$ $e q u a l t o 2 t i m e s θ f r o m \frac{π}{6} t o \frac{π}{2} d u e t o$ $s y m m e t r y$
	Commented by john santu last updated on 12/Apr/20

	$s i r, i f r = 2, y = 1$ $t h e n 2 \sin θ = 1 {\begin{cases} θ = \frac{π}{6} \\ θ = \frac{5 π}{6} \end{cases}$
	Commented by mr W last updated on 12/Apr/20

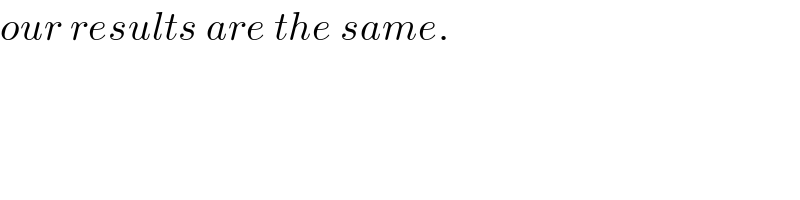
	$o u r r e s u l t s a r e t h e s a m e .$
	Commented by john santu last updated on 12/Apr/20

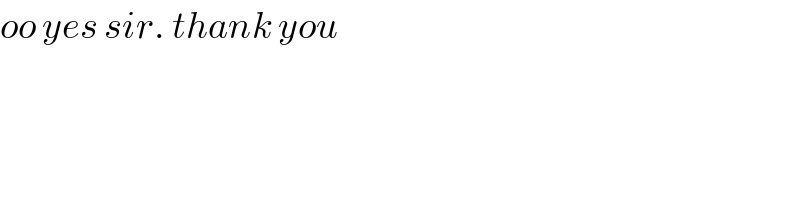
	$o o y e s s i r . t h a n k y o u$
	Commented by jagoll last updated on 12/Apr/20

	$t h a n k y o u m r W$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum