
Question and Answers Forum
Question Number 89891 by mathmax by abdo last updated on 19/Apr/20
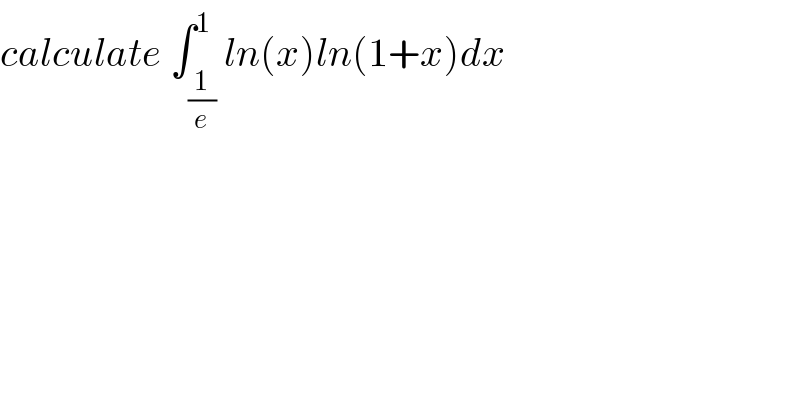
Commented by mathmax by abdo last updated on 20/Apr/20
![let take a try I =∫_e^(−1) ^e ln(x)ln(1+x)dx we have ln^′ (1+x)=Σ_(n=0) ^∞ (−1)^n x^n ⇒ln(1+x)=Σ_(n=0) ^∞ (−1)^n (x^(n+1) /(n+1)) =Σ_(n=1) ^∞ (−1)^(n−1) (x^n /n) ⇒I =∫_e^− ^e ln(x)(Σ_(n=1) ^∞ (−1)^(n−1) (x^n /n))dx =Σ_(n=1) ^∞ (((−1)^(n−1) )/n) ∫_e^(−1) ^e x^n ln(x)dx A_n =∫_e^(−1) ^e x^n ln(x)dx =_(bypsrts) [(x^(n+1) /(n+1))ln(x)]_e^(−1) ^e −∫_e^(−1) ^e (x^n /(n+1))dx =(1/((n+1))){e^(n+1) +(e^(−1) )^(n+1) }−(1/((n+1)))[(1/(n+1))x^(n+1) ]_e^(−1) ^e =(e^(n+1) /((n+1))) +(e^(−n−1) /(n+1))−(1/((n+1)^2 ))(e^(n+1) −e^(−n−1) ) ⇒ I =Σ_(n=1) ^∞ (((−1)^(n−1) e^(n+1) )/(n(n+1))) +Σ_(n=1) ^∞ (((−1)^(n−1) e^(−n−1) )/(n(n+1))) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 ))e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 ))e^(−n−1) we have Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)))e^(n+1) =Σ_(n=1) ^∞ ((1/n)−(1/(n+1)))(−1)^(n−1) e^(n+1) =eΣ_(n=1) ^∞ (((−1)^(n−1) )/n) e^n −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n+1))e^(n+1) =eln(1+e)+Σ_(n=2) ^∞ (((−1)^(n−1) )/n)e^n =eln(1+e)+ln(1+e) =(1+e)ln(1+e) let decompose F(x)=(1/(x(x+1)^2 )) =(a/x)+(b/(x+1)) +(c/((x+1)^2 )) c=−1 lim_(x→+∞) xF(x)=0=a+b ⇒b=−a ⇒ F(x)=(a/x)−(a/(x+1))−(1/((x+1)^2 )) F(1)=(1/4) =a−(a/2)−(1/4) =(a/2)−(1/4) ⇒(1/2)=(a/2) ⇒a=1 ⇒ F(x)=(1/x)−(1/(x+1))−(1/((x+1)^2 )) ⇒ Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 )) e^(n+1) =Σ_(n=1) ^∞ (−1)^(n−1) ((1/n)−(1/(n+1))−(1/((n+1)^2 )))e^(n+1) =Σ_(n=1) ^∞ (((−1)^(n−1) )/n) e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n+1)) e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/((n+1)^2 ))e^(n+1) ...be continued...](Q90006.png)
| ||
Question and Answers Forum | ||
Question Number 89891 by mathmax by abdo last updated on 19/Apr/20 | ||
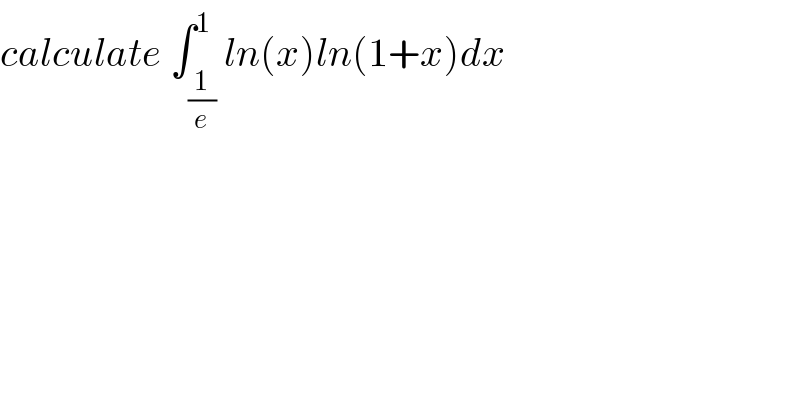 | ||
Commented by mathmax by abdo last updated on 20/Apr/20 | ||
![let take a try I =∫_e^(−1) ^e ln(x)ln(1+x)dx we have ln^′ (1+x)=Σ_(n=0) ^∞ (−1)^n x^n ⇒ln(1+x)=Σ_(n=0) ^∞ (−1)^n (x^(n+1) /(n+1)) =Σ_(n=1) ^∞ (−1)^(n−1) (x^n /n) ⇒I =∫_e^− ^e ln(x)(Σ_(n=1) ^∞ (−1)^(n−1) (x^n /n))dx =Σ_(n=1) ^∞ (((−1)^(n−1) )/n) ∫_e^(−1) ^e x^n ln(x)dx A_n =∫_e^(−1) ^e x^n ln(x)dx =_(bypsrts) [(x^(n+1) /(n+1))ln(x)]_e^(−1) ^e −∫_e^(−1) ^e (x^n /(n+1))dx =(1/((n+1))){e^(n+1) +(e^(−1) )^(n+1) }−(1/((n+1)))[(1/(n+1))x^(n+1) ]_e^(−1) ^e =(e^(n+1) /((n+1))) +(e^(−n−1) /(n+1))−(1/((n+1)^2 ))(e^(n+1) −e^(−n−1) ) ⇒ I =Σ_(n=1) ^∞ (((−1)^(n−1) e^(n+1) )/(n(n+1))) +Σ_(n=1) ^∞ (((−1)^(n−1) e^(−n−1) )/(n(n+1))) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 ))e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 ))e^(−n−1) we have Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)))e^(n+1) =Σ_(n=1) ^∞ ((1/n)−(1/(n+1)))(−1)^(n−1) e^(n+1) =eΣ_(n=1) ^∞ (((−1)^(n−1) )/n) e^n −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n+1))e^(n+1) =eln(1+e)+Σ_(n=2) ^∞ (((−1)^(n−1) )/n)e^n =eln(1+e)+ln(1+e) =(1+e)ln(1+e) let decompose F(x)=(1/(x(x+1)^2 )) =(a/x)+(b/(x+1)) +(c/((x+1)^2 )) c=−1 lim_(x→+∞) xF(x)=0=a+b ⇒b=−a ⇒ F(x)=(a/x)−(a/(x+1))−(1/((x+1)^2 )) F(1)=(1/4) =a−(a/2)−(1/4) =(a/2)−(1/4) ⇒(1/2)=(a/2) ⇒a=1 ⇒ F(x)=(1/x)−(1/(x+1))−(1/((x+1)^2 )) ⇒ Σ_(n=1) ^∞ (((−1)^(n−1) )/(n(n+1)^2 )) e^(n+1) =Σ_(n=1) ^∞ (−1)^(n−1) ((1/n)−(1/(n+1))−(1/((n+1)^2 )))e^(n+1) =Σ_(n=1) ^∞ (((−1)^(n−1) )/n) e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/(n+1)) e^(n+1) −Σ_(n=1) ^∞ (((−1)^(n−1) )/((n+1)^2 ))e^(n+1) ...be continued...](Q90006.png) | ||