
Question and Answers Forum
Question Number 90629 by Josephbaraka@gmail.com last updated on 25/Apr/20
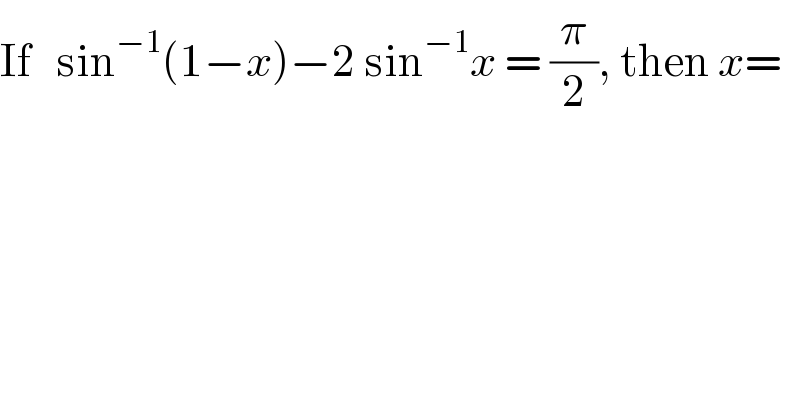
Commented by jagoll last updated on 25/Apr/20
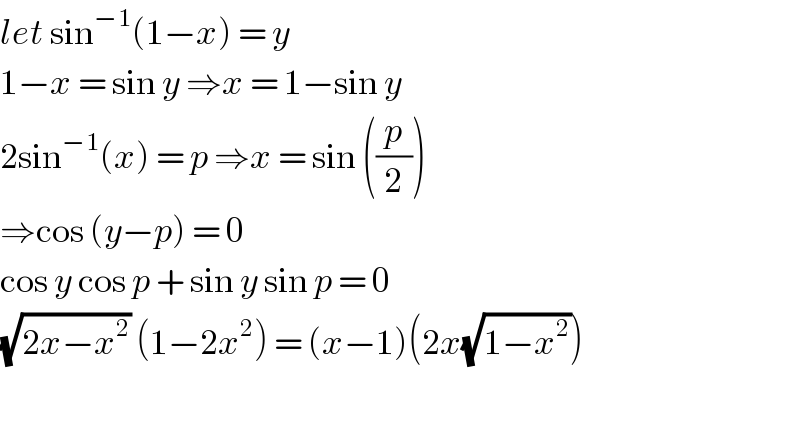
Answered by TANMAY PANACEA. last updated on 25/Apr/20

| ||
Question and Answers Forum | ||
Question Number 90629 by Josephbaraka@gmail.com last updated on 25/Apr/20 | ||
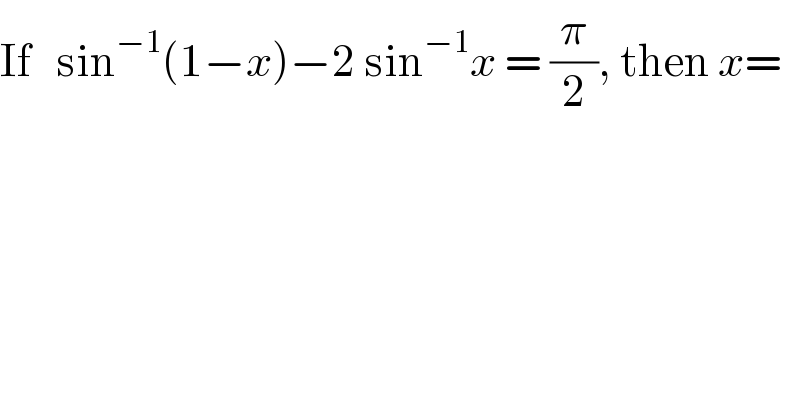 | ||
Commented by jagoll last updated on 25/Apr/20 | ||
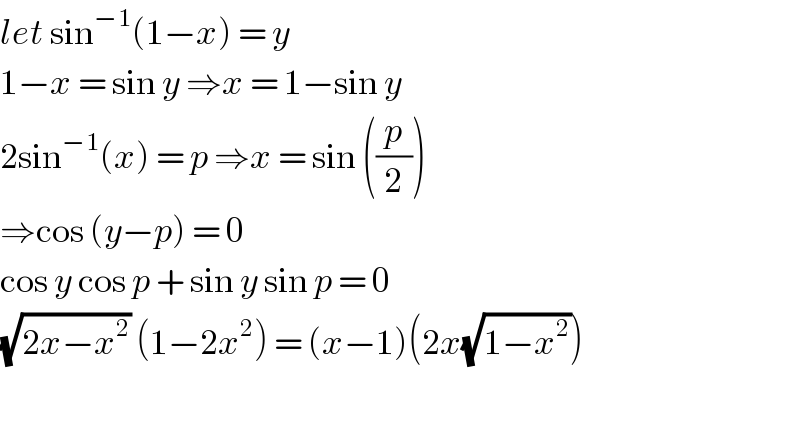 | ||
Answered by TANMAY PANACEA. last updated on 25/Apr/20 | ||
 | ||
| ||