
Question and Answers Forum
Question Number 90784 by jagoll last updated on 26/Apr/20
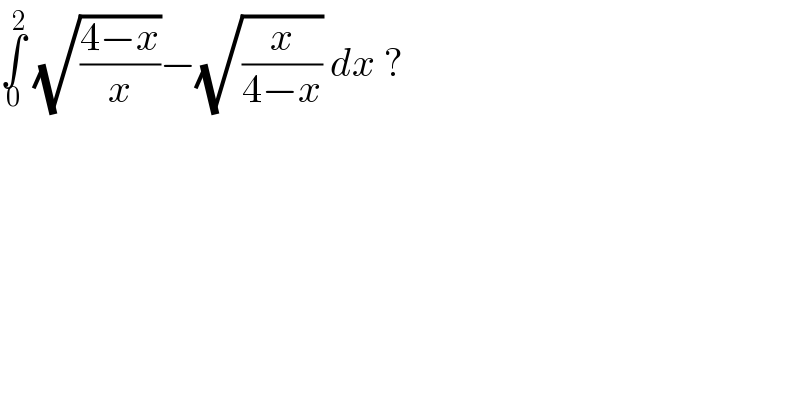
Commented by jagoll last updated on 26/Apr/20
![∫_0 ^2 ((((√(4−x)))^2 −((√x))^2 )/((√x) ((√(4−x)) ) )) = ∫_0 ^2 ((4−2x)/(√(4x−x^2 ))) dx = ∫_0 ^2 ((d(4x−x^2 ))/(√(4x−x^2 ))) = 2 (√(4x−x^2 )) ]_0 ^2 = 2 (√(8−4)) = 2×2 = 4](Q90785.png)
Commented by abdomathmax last updated on 27/Apr/20
![A=∫_0 ^2 (√((4−x)/x))dx−∫_0 ^2 (√(x/(4−x)))dx =H−K changement (√((4−x)/x))=t give ((4−x)/x)=t^2 ⇒ 4−x =t^2 x ⇒(1+t^2 )x =4 ⇒x=(4/(t^2 +1)) ⇒ H =−∫_1 ^(+∞) t×(−((4(2t))/((t^2 +1)^2 )))dt =8 ∫_1 ^(+∞) ((t^2 +1−1)/((t^2 +1)^2 ))dt =8 ∫_1 ^(+∞) (dt/(1+t^2 ))−8∫_1 ^(+∞) (dt/((1+t^2 )^2 )) ∫_1 ^(+∞) (dt/(1+t^2 )) =(π/2)−(π/4) =(π/4) ∫_1 ^(+∞) (dt/((1+t^2 )^2 )) =_(t=tanθ) ∫_(π/4) ^(π/2) ((1+tan^2 θ)/((1+tan^2 θ)^2 ))dθ =∫_(π/4) ^(π/2) cos^2 θ =(1/2)∫_(π/4) ^(π/2) (1+cos(2θ))dθ =(1/2)×(π/4) +(1/4)[sin(2θ)]_(π/4) ^(π/2) =(π/8)+(1/4)(−1) ⇒ H =2π−8{(π/8)−(1/4)}=π+2 and K=∫_0 ^2 (√(x/(4−x)))dx we do the changement (√(x/(4−x)))=t ⇒ (x/(4−x)) =t^2 ⇒x =4t^2 −t^2 x ⇒(1+t^2 )x =4t^2 ⇒ x =((4t^2 )/(t^2 +1)) ⇒(dx/dt) =((8t(t^2 +1)−4t^2 (2t))/((t^2 +1)^2 ))=((8t)/((t^2 +1)^2 )) K =∫_0 ^1 t×((8t)/((t^2 +1)^2 ))dt =8 ∫_0 ^1 ((t^2 +1−1)/((t^2 +1)^2 ))dt =8 ∫_0 ^1 (dt/(t^2 +1))−8 ∫_0 ^1 (dt/((t^2 +1)^2 )) =8×(π/4)−8{ ∫_0 ^(π/4) (((1+tan^2 θ)dθ)/((1+tan^2 θ)^2 ))} (t=tanθ) =2π −8{∫_0 ^(π/4) cos^2 θ dθ} =2π−4∫_0 ^(π/4) (1+cos(2θ))dθ =π−2 [sin(2θ)]_0 ^(π/4) =π−2{1} =π−2 A =H−K =π +2−π +2 =4](Q90939.png)
| ||
Question and Answers Forum | ||
Question Number 90784 by jagoll last updated on 26/Apr/20 | ||
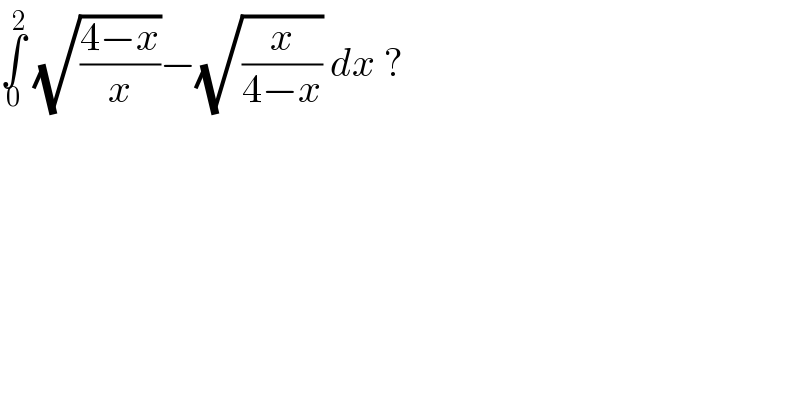 | ||
Commented by jagoll last updated on 26/Apr/20 | ||
![∫_0 ^2 ((((√(4−x)))^2 −((√x))^2 )/((√x) ((√(4−x)) ) )) = ∫_0 ^2 ((4−2x)/(√(4x−x^2 ))) dx = ∫_0 ^2 ((d(4x−x^2 ))/(√(4x−x^2 ))) = 2 (√(4x−x^2 )) ]_0 ^2 = 2 (√(8−4)) = 2×2 = 4](Q90785.png) | ||
Commented by abdomathmax last updated on 27/Apr/20 | ||
![A=∫_0 ^2 (√((4−x)/x))dx−∫_0 ^2 (√(x/(4−x)))dx =H−K changement (√((4−x)/x))=t give ((4−x)/x)=t^2 ⇒ 4−x =t^2 x ⇒(1+t^2 )x =4 ⇒x=(4/(t^2 +1)) ⇒ H =−∫_1 ^(+∞) t×(−((4(2t))/((t^2 +1)^2 )))dt =8 ∫_1 ^(+∞) ((t^2 +1−1)/((t^2 +1)^2 ))dt =8 ∫_1 ^(+∞) (dt/(1+t^2 ))−8∫_1 ^(+∞) (dt/((1+t^2 )^2 )) ∫_1 ^(+∞) (dt/(1+t^2 )) =(π/2)−(π/4) =(π/4) ∫_1 ^(+∞) (dt/((1+t^2 )^2 )) =_(t=tanθ) ∫_(π/4) ^(π/2) ((1+tan^2 θ)/((1+tan^2 θ)^2 ))dθ =∫_(π/4) ^(π/2) cos^2 θ =(1/2)∫_(π/4) ^(π/2) (1+cos(2θ))dθ =(1/2)×(π/4) +(1/4)[sin(2θ)]_(π/4) ^(π/2) =(π/8)+(1/4)(−1) ⇒ H =2π−8{(π/8)−(1/4)}=π+2 and K=∫_0 ^2 (√(x/(4−x)))dx we do the changement (√(x/(4−x)))=t ⇒ (x/(4−x)) =t^2 ⇒x =4t^2 −t^2 x ⇒(1+t^2 )x =4t^2 ⇒ x =((4t^2 )/(t^2 +1)) ⇒(dx/dt) =((8t(t^2 +1)−4t^2 (2t))/((t^2 +1)^2 ))=((8t)/((t^2 +1)^2 )) K =∫_0 ^1 t×((8t)/((t^2 +1)^2 ))dt =8 ∫_0 ^1 ((t^2 +1−1)/((t^2 +1)^2 ))dt =8 ∫_0 ^1 (dt/(t^2 +1))−8 ∫_0 ^1 (dt/((t^2 +1)^2 )) =8×(π/4)−8{ ∫_0 ^(π/4) (((1+tan^2 θ)dθ)/((1+tan^2 θ)^2 ))} (t=tanθ) =2π −8{∫_0 ^(π/4) cos^2 θ dθ} =2π−4∫_0 ^(π/4) (1+cos(2θ))dθ =π−2 [sin(2θ)]_0 ^(π/4) =π−2{1} =π−2 A =H−K =π +2−π +2 =4](Q90939.png) | ||