Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 90787 by Maclaurin Stickker last updated on 26/Apr/20

$$Findtheinfinitesum$$$$\underset{n=1}{\sum ^{\mathrm{\infty }}}\frac{1}{(2n-1)(2n+1)(2n+3)}$$

Commented by mathmax by abdo last updated on 26/Apr/20

$$letdecomposeF\left(x\right)=\frac{1}{(2x-1)(2x+1)(2x+3)}$$$$F\left(x\right)=\frac{a}{2x-1}+\frac{b}{2x+1}+\frac{c}{2x+3}$$$$a=(2x-1)F\left(x\right){\mid }_{x=\frac{1}{2}}=\frac{1}{\left(2\right)\left(4\right)}=\frac{1}{8}$$$$b=(2x+1)F\left(x\right){\mid }_{x=-\frac{1}{2}}=\frac{1}{(-2)\left(2\right)}=-\frac{1}{4}$$$$c=(2x+3)F\left(x\right){\mid }_{x=-\frac{3}{2}}=\frac{1}{(-4)(-2)}=\frac{1}{8}\Rightarrow$$$$F\left(x\right)=\frac{1}{8(2x-1)}-\frac{1}{4(2x+1)}+\frac{1}{8(2x+3)}let$$$$S_n=\sum _{k=1}^n\frac{1}{(2k-1)(2k+1)(2k+3)}\Rightarrow S_n=\mathrm{\Sigma }F\left(k\right)$$$$=\frac{1}{8}\sum _{k=1}^n\frac{1}{2k-1}-\frac{1}{4}\sum _{k=1}^n\frac{1}{2k+1}+\frac{1}{8}\sum _{k=1}^n\frac{1}{2k+3}wehave$$$$\sum _{k=1}^n\frac{1}{2k-1}=1+\frac{1}{3}+\frac{1}{5}+....+\frac{1}{2n-1}$$$$=1+\frac{1}{2}+\frac{1}{3}+...+\frac{1}{2n-1}+\frac{1}{2n}-\frac{1}{2}-\frac{1}{4}-....-\frac{1}{2n}$$$$=H_{2n}-\frac{1}{2}{H}_n$$$$\sum _{k=1}^n\frac{1}{2k+1}{=}_{k=j-1}\sum _{j=2}^{n+1}\frac{1}{2j-1}=\sum _{j=1}^{n+1}\frac{1}{2j-1}-1$$$$=H_{2n+2}-\frac{1}{2}{H}_{n+1}-1$$$$\sum _{k=1}^n\frac{1}{2k+3}{=}_{k=j-2}\sum _{j=3}^{n+2}\frac{1}{2j-1}=\sum _{j=1}^{n+2}\frac{1}{2j-1}-1-\frac{1}{3}$$$$=H_{2n+4}-\frac{1}{2}{H}_{n+2}-\frac{4}{3}\Rightarrow$$$$8S_n=H_{2n}-\frac{1}{2}{H}_n-2(H_{2n+2}-\frac{1}{2}{H}_{n+1}-1)+H_{2n+4}-\frac{1}{2}{H}_{n+2}-\frac{4}{3}$$$$=H_{2n}-\frac{1}{2}{H}_n-2H_{2n+2}+H_{n+1}+2+H_{2n+4}-\frac{1}{2}{H}_{n+2}-\frac{4}{3}$$$$\sim ln\left(2n\right)+\gamma -\frac{1}{2}ln\left(n\right)-\frac{\gamma }{2}-2ln(2n+2)-2\gamma +ln(n+1)+\gamma$$$$+ln(2n+4)+\gamma -\frac{1}{2}ln(n+2)-\frac{\gamma }{2}+\frac{2}{3}\Rightarrow$$$$8S_n\sim ln\left(2\right)+ln\left(n\right)-\frac{1}{2}ln\left(n\right)-2ln\left(2\right)-2ln\left(n\right)+ln\left(n\right)$$$$+ln\left(2\right)+ln\left(n\right)-\frac{1}{2}ln\left(n\right)+\frac{2}{3}\Rightarrow 8S_n\sim \frac{2}{3}\Rightarrow S_n\sim \frac{1}{12}$$$$\Rightarrow {lim}_{n\to +\mathrm{\infty }}{S}_n=\frac{1}{12}$$

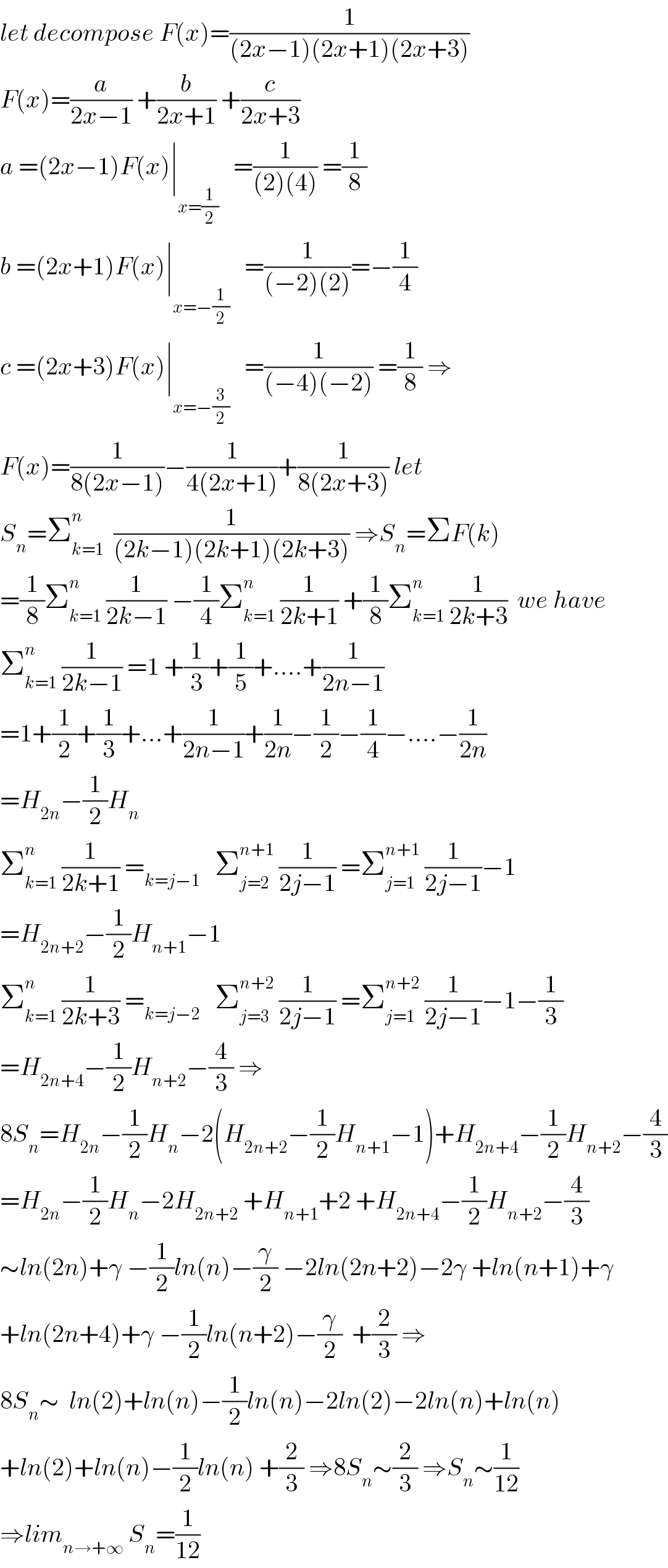
Commented by Maclaurin Stickker last updated on 26/Apr/20

$$Thankyou,Iappreciateyourwork.$$

Commented by abdomathmax last updated on 27/Apr/20

$$youarewelcome$$

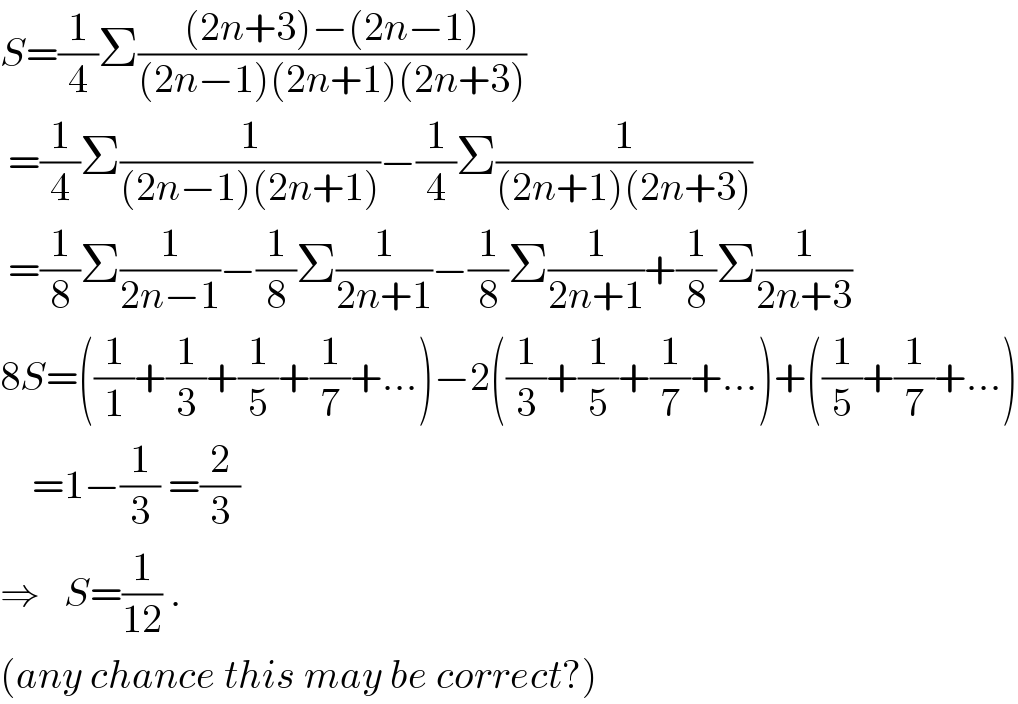
Answered by ajfour last updated on 26/Apr/20

$$S=\frac{1}{4}\mathrm{\Sigma }\frac{(2n+3)-(2n-1)}{(2n-1)(2n+1)(2n+3)}$$$$=\frac{1}{4}\mathrm{\Sigma }\frac{1}{(2n-1)(2n+1)}-\frac{1}{4}\mathrm{\Sigma }\frac{1}{(2n+1)(2n+3)}$$$$=\frac{1}{8}\mathrm{\Sigma }\frac{1}{2n-1}-\frac{1}{8}\mathrm{\Sigma }\frac{1}{2n+1}-\frac{1}{8}\mathrm{\Sigma }\frac{1}{2n+1}+\frac{1}{8}\mathrm{\Sigma }\frac{1}{2n+3}$$$$8S=(\frac{1}{1}+\frac{1}{3}+\frac{1}{5}+\frac{1}{7}+...)-2(\frac{1}{3}+\frac{1}{5}+\frac{1}{7}+...)+(\frac{1}{5}+\frac{1}{7}+...)$$$$=1-\frac{1}{3}=\frac{2}{3}$$$$\Rightarrow S=\frac{1}{12}.$$$$(anychancethismaybecorrect?)$$

Commented by mathmax by abdo last updated on 26/Apr/20

$$correctsirajfour$$

Commented by Maclaurin Stickker last updated on 26/Apr/20

$$Perfect!$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com