
Previous in Relation and Functions Next in Relation and Functions
Question Number 90968 by abdomathmax last updated on 27/Apr/20
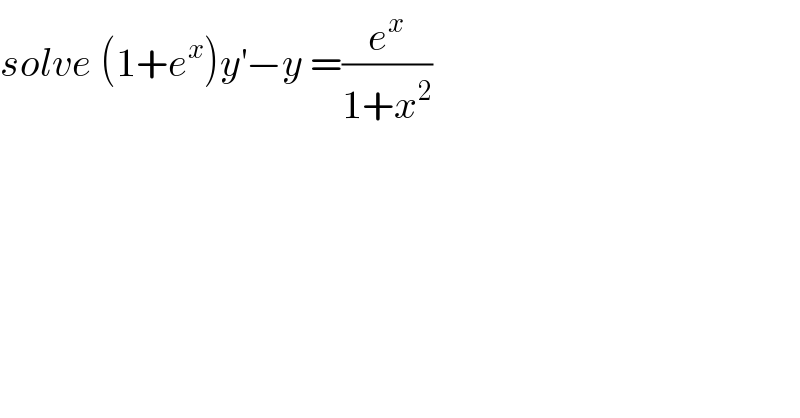
$${solve}\:\left(\mathrm{1}+{e}^{{x}} \right){y}^{'} −{y}\:=\frac{{e}^{{x}} }{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$
Commented by niroj last updated on 27/Apr/20
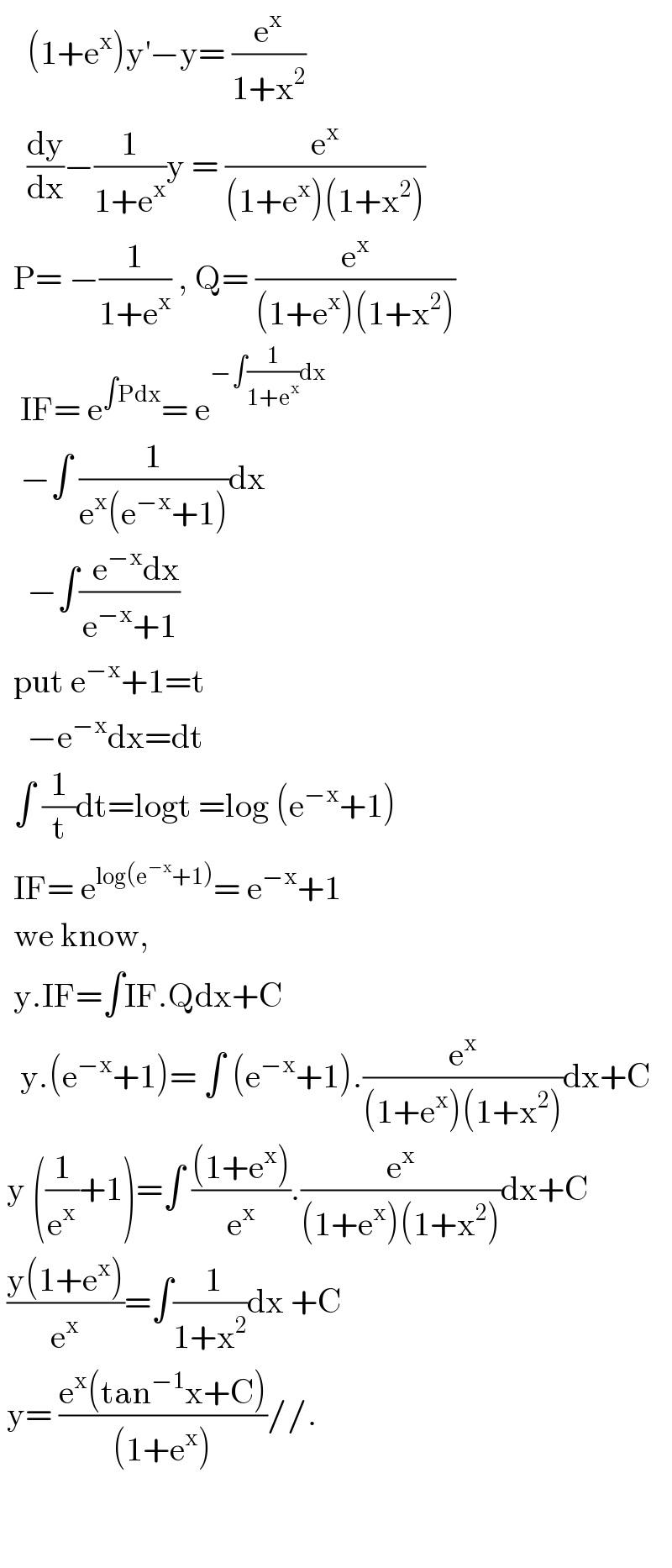
$$\:\:\:\:\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)\mathrm{y}^{'} −\mathrm{y}=\:\frac{\mathrm{e}^{\mathrm{x}} }{\mathrm{1}+\mathrm{x}^{\mathrm{2}} } \\ $$$$\:\:\:\:\frac{\mathrm{dy}}{\mathrm{dx}}−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{e}^{\mathrm{x}} }\mathrm{y}\:=\:\frac{\mathrm{e}^{\mathrm{x}} }{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)} \\ $$$$\:\:\mathrm{P}=\:−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{e}^{\mathrm{x}} }\:,\:\mathrm{Q}=\:\frac{\mathrm{e}^{\mathrm{x}} }{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)} \\ $$$$\:\:\:\mathrm{IF}=\:\mathrm{e}^{\int\mathrm{Pdx}} =\:\mathrm{e}^{−\int\frac{\mathrm{1}}{\mathrm{1}+\mathrm{e}^{\mathrm{x}} }\mathrm{dx}} \\ $$$$\:\:\:−\int\:\frac{\mathrm{1}}{\mathrm{e}^{\mathrm{x}} \left(\mathrm{e}^{−\mathrm{x}} +\mathrm{1}\right)}\mathrm{dx} \\ $$$$\:\:\:\:−\int\frac{\:\:\mathrm{e}^{−\mathrm{x}} \mathrm{dx}}{\mathrm{e}^{−\mathrm{x}} +\mathrm{1}} \\ $$$$\:\:\mathrm{put}\:\mathrm{e}^{−\mathrm{x}} +\mathrm{1}=\mathrm{t} \\ $$$$\:\:\:\:−\mathrm{e}^{−\mathrm{x}} \mathrm{dx}=\mathrm{dt} \\ $$$$\:\:\int\:\frac{\mathrm{1}}{\mathrm{t}}\mathrm{dt}=\mathrm{logt}\:=\mathrm{log}\:\left(\mathrm{e}^{−\mathrm{x}} +\mathrm{1}\right) \\ $$$$\:\:\mathrm{IF}=\:\mathrm{e}^{\mathrm{log}\left(\mathrm{e}^{−\mathrm{x}} +\mathrm{1}\right)} =\:\mathrm{e}^{−\mathrm{x}} +\mathrm{1} \\ $$$$\:\:\mathrm{we}\:\mathrm{know}, \\ $$$$\:\:\mathrm{y}.\mathrm{IF}=\int\mathrm{IF}.\mathrm{Qdx}+\mathrm{C} \\ $$$$\:\:\:\mathrm{y}.\left(\mathrm{e}^{−\mathrm{x}} +\mathrm{1}\right)=\:\int\:\left(\mathrm{e}^{−\mathrm{x}} +\mathrm{1}\right).\frac{\mathrm{e}^{\mathrm{x}} }{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}\mathrm{dx}+\mathrm{C} \\ $$$$\:\mathrm{y}\:\left(\frac{\mathrm{1}}{\mathrm{e}^{\mathrm{x}} }+\mathrm{1}\right)=\int\:\frac{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}{\mathrm{e}^{\mathrm{x}} }.\frac{\mathrm{e}^{\mathrm{x}} }{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)}\mathrm{dx}+\mathrm{C} \\ $$$$\:\frac{\mathrm{y}\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}{\mathrm{e}^{\mathrm{x}} }=\int\frac{\:\mathrm{1}}{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }\mathrm{dx}\:+\mathrm{C} \\ $$$$\:\mathrm{y}=\:\frac{\mathrm{e}^{\mathrm{x}} \left(\mathrm{tan}^{−\mathrm{1}} \mathrm{x}+\mathrm{C}\right)}{\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}//. \\ $$$$ \\ $$$$ \\ $$
Commented by mathmax by abdo last updated on 28/Apr/20
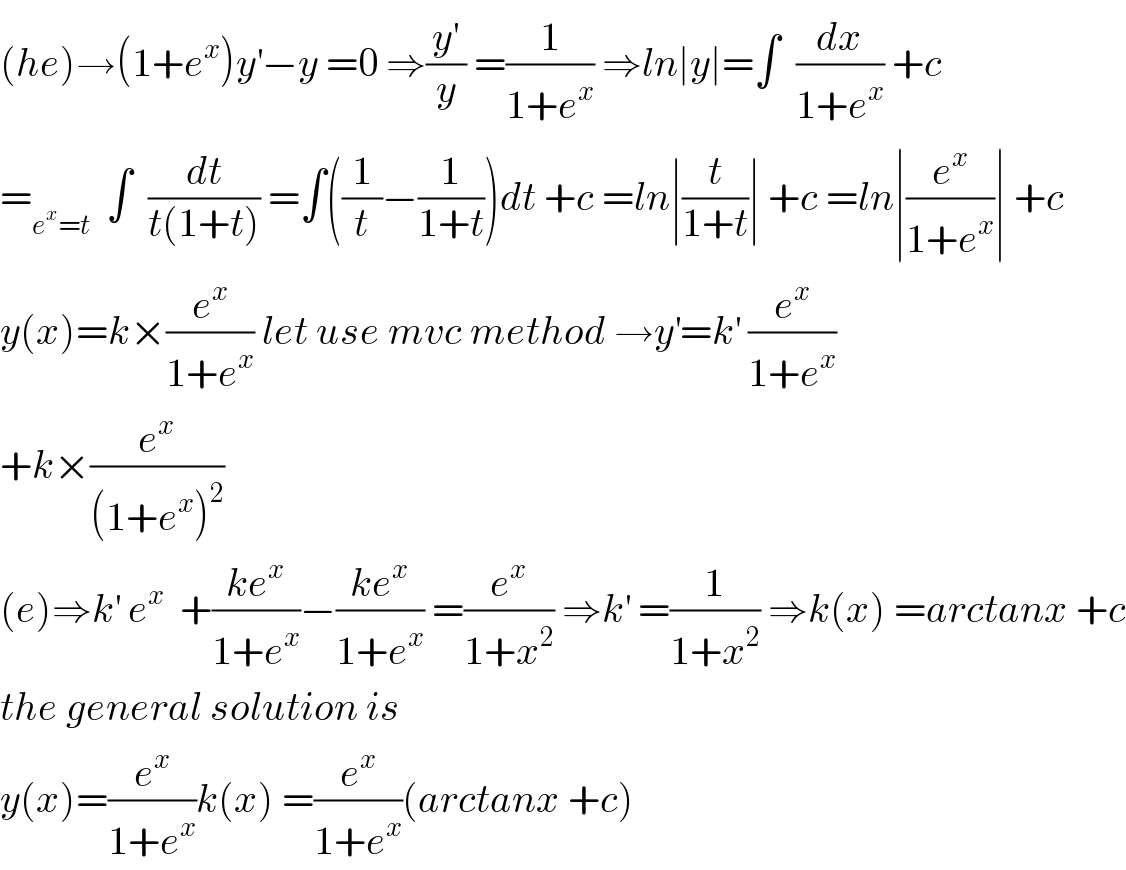
$$\left({he}\right)\rightarrow\left(\mathrm{1}+{e}^{{x}} \right){y}^{'} −{y}\:=\mathrm{0}\:\Rightarrow\frac{{y}^{'} }{{y}}\:=\frac{\mathrm{1}}{\mathrm{1}+{e}^{{x}} }\:\Rightarrow{ln}\mid{y}\mid=\int\:\:\frac{{dx}}{\mathrm{1}+{e}^{{x}} }\:+{c} \\ $$$$=_{{e}^{{x}} ={t}} \:\:\int\:\:\frac{{dt}}{{t}\left(\mathrm{1}+{t}\right)}\:=\int\left(\frac{\mathrm{1}}{{t}}−\frac{\mathrm{1}}{\mathrm{1}+{t}}\right){dt}\:+{c}\:={ln}\mid\frac{{t}}{\mathrm{1}+{t}}\mid\:+{c}\:={ln}\mid\frac{{e}^{{x}} }{\mathrm{1}+{e}^{{x}} }\mid\:+{c} \\ $$$${y}\left({x}\right)={k}×\frac{{e}^{{x}} }{\mathrm{1}+{e}^{{x}} }\:{let}\:{use}\:{mvc}\:{method}\:\rightarrow{y}^{'} ={k}^{'} \:\frac{{e}^{{x}} }{\mathrm{1}+{e}^{{x}} } \\ $$$$+{k}×\frac{{e}^{{x}} }{\left(\mathrm{1}+{e}^{{x}} \right)^{\mathrm{2}} } \\ $$$$\left({e}\right)\Rightarrow{k}^{'} \:{e}^{{x}} \:\:+\frac{{ke}^{{x}} }{\mathrm{1}+{e}^{{x}} }−\frac{{ke}^{{x}} }{\mathrm{1}+{e}^{{x}} }\:=\frac{{e}^{{x}} }{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow{k}^{'} \:=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow{k}\left({x}\right)\:={arctanx}\:+{c} \\ $$$${the}\:{general}\:{solution}\:{is} \\ $$$${y}\left({x}\right)=\frac{{e}^{{x}} }{\mathrm{1}+{e}^{{x}} }{k}\left({x}\right)\:=\frac{{e}^{{x}} }{\mathrm{1}+{e}^{{x}} }\left({arctanx}\:+{c}\right) \\ $$
