Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 91620 by abdomathmax last updated on 02/May/20

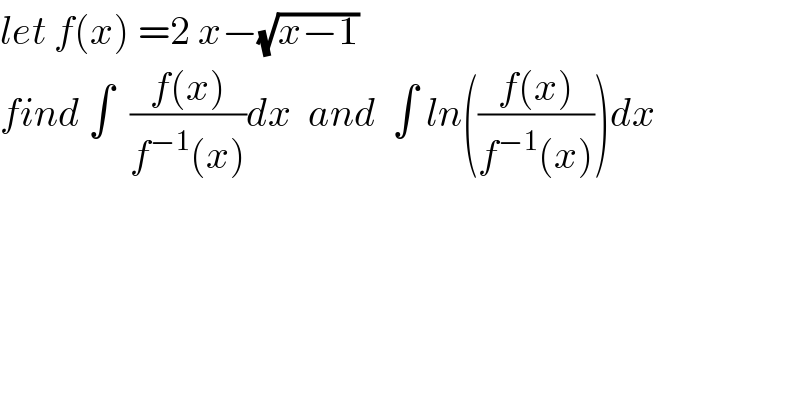
let f(x) =2 x−(√(x−1)) find ∫ ((f(x))/(f^(−1) (x)))dx and ∫ ln(((f(x))/(f^(−1) (x))))dx

$${let}\:{f}\left({x}\right)\:=\mathrm{2}\:{x}−\sqrt{{x}−\mathrm{1}} \\ $$$${find}\:\int\:\:\frac{{f}\left({x}\right)}{{f}^{−\mathrm{1}} \left({x}\right)}{dx}\:\:{and}\:\:\int\:{ln}\left(\frac{{f}\left({x}\right)}{{f}^{−\mathrm{1}} \left({x}\right)}\right){dx} \\ $$

Commented by mathmax by abdo last updated on 02/May/20

f(x)=y ⇔x =f^(−1) (y) (x≥1) f(x)=y ⇔2x−(√(x−1))=y ⇒2x−y =(√(x−1)) ⇒(2x−y)^2 =x−1 ⇒ 4x^2 −4yx +y^2 −x+1 =0 ⇒4x^2 −(4y+1)x +y^2 +1 =0 Δ =(4y+1)^2 −16(y^2 +1) =16y^2 +8y+1−16y^2 −16 =8y−15 if y≥((15)/8)x_1 =((4y+1+(√(8y−15)))/8) and x_2 =((4y+1−(√(8y−15)))/8) we must have x≥1 so x_1 −1 =((4y+1+(√(8y−15)))/8)−1 =((4y+1+(√(8y−15))−8)/8) =((4y−7+(√(8y−15)))/8) y≥((15)/8) ⇒4y−7 ≥((15)/2)−7>0 ⇒x_1 >1 ⇒ f^(−1) (x) =((4x+1+(√(8x−15)))/8) ⇒ ∫ ((f(x))/(f^(−1) (x)))dx =8 ∫ ((2x−(√(x−1)))/(4x+1+(√(8x−15))))dx changement (√(x−1))=t give x−1=t^2 ⇒∫ ((f(x))/(f^(−1) (x)))dx =8 ∫ ((2(1+t^2 )−t)/(4(1+t^2 )+1+(√(8(1+t^2 )−15))))(2t)dt =16 ∫ ((2t^3 −t^2 +2t)/(5+4t^2 +(√(8t^2 −7)))) dt changement t =(√(7/8))ch(u) give I =16 ∫ ((2((√(7/8)))^3 ch^3 (u)−(7/8)ch^2 (u) +2(√(7/8))ch(u))/(5+(7/2)ch^2 u +(√8)×(√(7/8))shu))×(√(7/8))shu du ...be continued...

$${f}\left({x}\right)={y}\:\Leftrightarrow{x}\:={f}^{−\mathrm{1}} \left({y}\right)\:\left({x}\geqslant\mathrm{1}\right) \\ $$$${f}\left({x}\right)={y}\:\Leftrightarrow\mathrm{2}{x}−\sqrt{{x}−\mathrm{1}}={y}\:\Rightarrow\mathrm{2}{x}−{y}\:=\sqrt{{x}−\mathrm{1}}\:\Rightarrow\left(\mathrm{2}{x}−{y}\right)^{\mathrm{2}} ={x}−\mathrm{1}\:\Rightarrow \\ $$$$\mathrm{4}{x}^{\mathrm{2}} −\mathrm{4}{yx}\:+{y}^{\mathrm{2}} −{x}+\mathrm{1}\:=\mathrm{0}\:\Rightarrow\mathrm{4}{x}^{\mathrm{2}} −\left(\mathrm{4}{y}+\mathrm{1}\right){x}\:+{y}^{\mathrm{2}} \:+\mathrm{1}\:=\mathrm{0} \\ $$$$\Delta\:=\left(\mathrm{4}{y}+\mathrm{1}\right)^{\mathrm{2}} −\mathrm{16}\left({y}^{\mathrm{2}} +\mathrm{1}\right)\:=\mathrm{16}{y}^{\mathrm{2}} \:+\mathrm{8}{y}+\mathrm{1}−\mathrm{16}{y}^{\mathrm{2}} −\mathrm{16} \\ $$$$=\mathrm{8}{y}−\mathrm{15}\:{if}\:{y}\geqslant\frac{\mathrm{15}}{\mathrm{8}}{x}_{\mathrm{1}} =\frac{\mathrm{4}{y}+\mathrm{1}+\sqrt{\mathrm{8}{y}−\mathrm{15}}}{\mathrm{8}}\:{and}\:{x}_{\mathrm{2}} =\frac{\mathrm{4}{y}+\mathrm{1}−\sqrt{\mathrm{8}{y}−\mathrm{15}}}{\mathrm{8}} \\ $$$${we}\:{must}\:{have}\:{x}\geqslant\mathrm{1}\:{so} \\ $$$${x}_{\mathrm{1}} −\mathrm{1}\:=\frac{\mathrm{4}{y}+\mathrm{1}+\sqrt{\mathrm{8}{y}−\mathrm{15}}}{\mathrm{8}}−\mathrm{1}\:=\frac{\mathrm{4}{y}+\mathrm{1}+\sqrt{\mathrm{8}{y}−\mathrm{15}}−\mathrm{8}}{\mathrm{8}} \\ $$$$=\frac{\mathrm{4}{y}−\mathrm{7}+\sqrt{\mathrm{8}{y}−\mathrm{15}}}{\mathrm{8}}\:\:\:\:\:\:{y}\geqslant\frac{\mathrm{15}}{\mathrm{8}}\:\Rightarrow\mathrm{4}{y}−\mathrm{7}\:\geqslant\frac{\mathrm{15}}{\mathrm{2}}−\mathrm{7}>\mathrm{0}\:\Rightarrow{x}_{\mathrm{1}} >\mathrm{1}\:\Rightarrow \\ $$$${f}^{−\mathrm{1}} \left({x}\right)\:=\frac{\mathrm{4}{x}+\mathrm{1}+\sqrt{\mathrm{8}{x}−\mathrm{15}}}{\mathrm{8}}\:\Rightarrow \\ $$$$\int\:\frac{{f}\left({x}\right)}{{f}^{−\mathrm{1}} \left({x}\right)}{dx}\:=\mathrm{8}\:\int\:\:\frac{\mathrm{2}{x}−\sqrt{{x}−\mathrm{1}}}{\mathrm{4}{x}+\mathrm{1}+\sqrt{\mathrm{8}{x}−\mathrm{15}}}{dx}\:\:{changement}\:\sqrt{{x}−\mathrm{1}}={t}\:{give} \\ $$$${x}−\mathrm{1}={t}^{\mathrm{2}} \:\Rightarrow\int\:\frac{{f}\left({x}\right)}{{f}^{−\mathrm{1}} \left({x}\right)}{dx}\:=\mathrm{8}\:\int\:\:\frac{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)−{t}}{\mathrm{4}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)+\mathrm{1}+\sqrt{\mathrm{8}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)−\mathrm{15}}}\left(\mathrm{2}{t}\right){dt} \\ $$$$=\mathrm{16}\:\int\:\:\frac{\mathrm{2}{t}^{\mathrm{3}} −{t}^{\mathrm{2}} +\mathrm{2}{t}}{\mathrm{5}+\mathrm{4}{t}^{\mathrm{2}} +\sqrt{\mathrm{8}{t}^{\mathrm{2}} −\mathrm{7}}}\:{dt}\:\:\:{changement}\:\:{t}\:=\sqrt{\frac{\mathrm{7}}{\mathrm{8}}}{ch}\left({u}\right)\:{give} \\ $$$${I}\:=\mathrm{16}\:\int\:\:\frac{\mathrm{2}\left(\sqrt{\frac{\mathrm{7}}{\mathrm{8}}}\right)^{\mathrm{3}} {ch}^{\mathrm{3}} \left({u}\right)−\frac{\mathrm{7}}{\mathrm{8}}{ch}^{\mathrm{2}} \left({u}\right)\:+\mathrm{2}\sqrt{\frac{\mathrm{7}}{\mathrm{8}}}{ch}\left({u}\right)}{\mathrm{5}+\frac{\mathrm{7}}{\mathrm{2}}{ch}^{\mathrm{2}} {u}\:+\sqrt{\mathrm{8}}×\sqrt{\frac{\mathrm{7}}{\mathrm{8}}}{shu}}×\sqrt{\frac{\mathrm{7}}{\mathrm{8}}}{shu}\:{du} \\ $$$$...{be}\:{continued}... \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com