
Question and Answers Forum
Question Number 91892 by john santu last updated on 03/May/20
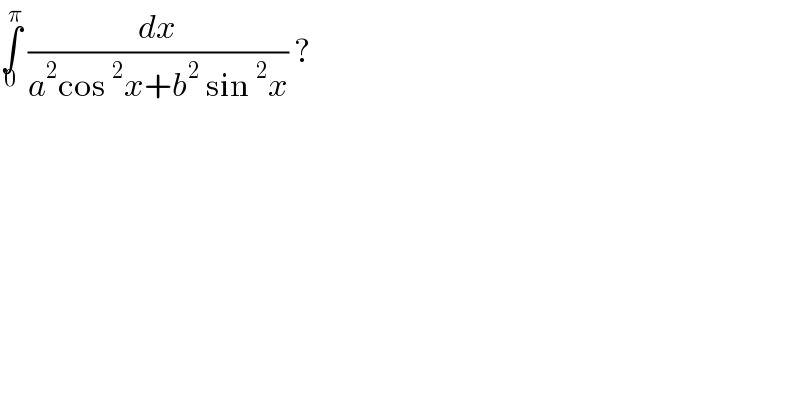
Commented by john santu last updated on 03/May/20
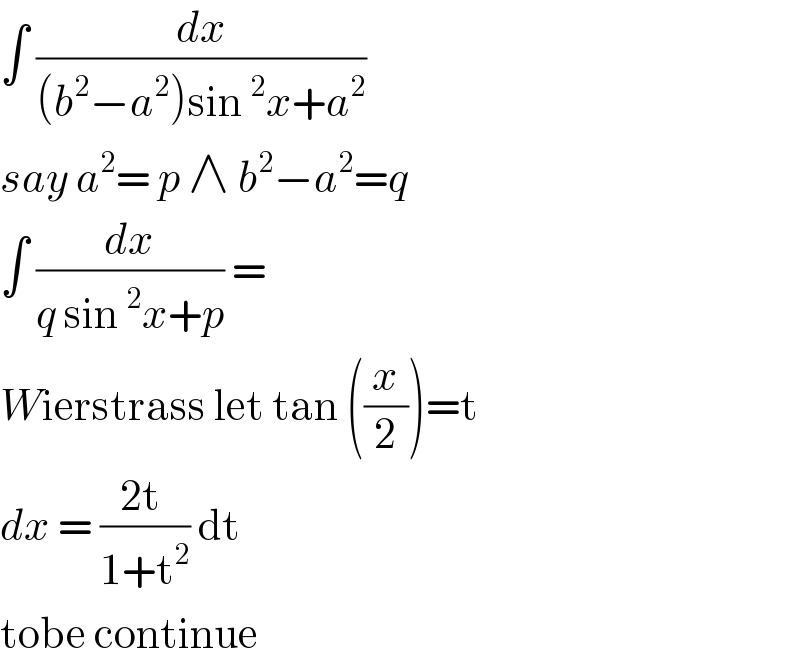
Commented by Prithwish Sen 1 last updated on 03/May/20
![∫_0 ^π ((sec^2 x dx)/(a^2 +b^2 tan^2 x)) = (1/(ab))[tan^(−1) (((btan x)/a))]_0 ^π](Q91908.png)
Commented by mathmax by abdo last updated on 03/May/20

Commented by john santu last updated on 04/May/20
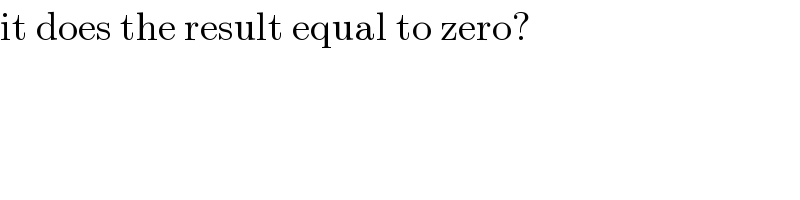
Commented by john santu last updated on 04/May/20

