Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 92521 by hovero clinton last updated on 07/May/20

Commented by hovero clinton last updated on 07/May/20

my post here never get answers y??

$${my}\:{post}\:{here}\:{never}\: \\ $$$${get}\:{answers}\:{y}?? \\ $$

Commented by mr W last updated on 07/May/20

it′s not due you personally, but mainly due to your question, maybe the question is just too hard for the people here.

$${it}'{s}\:{not}\:{due}\:{you}\:{personally},\:{but}\:{mainly} \\ $$$${due}\:{to}\:{your}\:{question},\:{maybe}\:{the} \\ $$$${question}\:{is}\:{just}\:{too}\:{hard}\:{for}\:{the} \\ $$$${people}\:{here}. \\ $$

Commented by hovero clinton last updated on 07/May/20

ok sir but I post questions I need help in

Commented by mr W last updated on 07/May/20

what′s your logic sir? you need help doesn′t mean that other people can and should help you. as i have said, maybe your question is too hard, other people want to, but are not able to help you.

$${what}'{s}\:{your}\:{logic}\:{sir}?\:{you}\:{need}\:{help} \\ $$$${doesn}'{t}\:{mean}\:{that}\:{other}\:{people}\:{can} \\ $$$${and}\:{should}\:{help}\:{you}.\:\:{as}\:{i}\:{have}\:{said}, \\ $$$${maybe}\:{your}\:{question}\:{is}\:{too}\:{hard},\:{other} \\ $$$${people}\:{want}\:{to},\:{but}\:{are}\:{not}\:{able}\:{to} \\ $$$${help}\:{you}. \\ $$

Commented by prakash jain last updated on 07/May/20

x^4 +1=(x^2 −i)(x^2 +i) =(x−(√i))(x+(√i))(x−i(√i))(x+i(√i)) x^4 +1 has 4 roots (4^(th) root of umiy) For ease in typing let us write that as a,−a,b,−b (1/((x^4 +1)^2 ))=(1/((x+a)^2 (x−a)^2 (x+b)^2 (x−b)^2 )) if we use partial fraction you will get following form (A/((x−a)))+(B/((x−a)^2 ))+... basically ((constant)/(linear))+((constant)/(quadratic)) with these step the final 8 integrals will be of two forms ((Cx)/((x+k)^2 (√(x^2 −x+1)))), ((Cx)/((x+k)(√(x^2 −x+1)))) =which can be integrated using standard formulas

$${x}^{\mathrm{4}} +\mathrm{1}=\left({x}^{\mathrm{2}} −{i}\right)\left({x}^{\mathrm{2}} +{i}\right) \\ $$$$=\left({x}−\sqrt{{i}}\right)\left({x}+\sqrt{{i}}\right)\left({x}−{i}\sqrt{{i}}\right)\left({x}+{i}\sqrt{{i}}\right) \\ $$$${x}^{\mathrm{4}} +\mathrm{1}\:\mathrm{has}\:\mathrm{4}\:\mathrm{roots}\:\left(\mathrm{4}^{\mathrm{th}} \mathrm{root}\:\mathrm{of}\:\mathrm{umiy}\right) \\ $$$$\mathrm{For}\:\mathrm{ease}\:\mathrm{in}\:\mathrm{typing}\:\mathrm{let}\:\mathrm{us}\:\mathrm{write}\:\mathrm{that}\:\mathrm{as} \\ $$$${a},−{a},{b},−{b} \\ $$$$\frac{\mathrm{1}}{\left({x}^{\mathrm{4}} +\mathrm{1}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{\left({x}+{a}\right)^{\mathrm{2}} \left({x}−{a}\right)^{\mathrm{2}} \left({x}+{b}\right)^{\mathrm{2}} \left({x}−{b}\right)^{\mathrm{2}} } \\ $$$${if}\:{we}\:{use}\:{partial}\:{fraction}\:{you}\:{will} \\ $$$${get}\:{following}\:{form} \\ $$$$\frac{{A}}{\left({x}−{a}\right)}+\frac{{B}}{\left({x}−{a}\right)^{\mathrm{2}} }+...\: \\ $$$${basically}\:\frac{{constant}}{{linear}}+\frac{{constant}}{{quadratic}} \\ $$$${with}\:{these}\:{step}\: \\ $$$${the}\:{final}\:\mathrm{8}\:{integrals}\:{will}\:{be}\:{of}\:{two} \\ $$$${forms}\: \\ $$$$\frac{{Cx}}{\left({x}+{k}\right)^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}},\:\frac{{Cx}}{\left({x}+{k}\right)\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}} \\ $$$$={which}\:{can}\:{be}\:{integrated}\:{using} \\ $$$${standard}\:{formulas} \\ $$

Commented by prakash jain last updated on 07/May/20

You will get very long answer value for integral What is the source of question

$$\mathrm{You}\:\mathrm{will}\:\mathrm{get}\:\mathrm{very}\:\mathrm{long}\:\mathrm{answer}\:\mathrm{value}\:\mathrm{for}\:\mathrm{integral} \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{source}\:\mathrm{of}\:\mathrm{question} \\ $$

Commented by MJS last updated on 07/May/20

I tried almost everything I know but I cannot solve this integral...

$$\mathrm{I}\:\mathrm{tried}\:\mathrm{almost}\:\mathrm{everything}\:\mathrm{I}\:\mathrm{know}\:\mathrm{but}\:\mathrm{I}\:\mathrm{cannot} \\ $$$$\mathrm{solve}\:\mathrm{this}\:\mathrm{integral}... \\ $$

Commented by turbo msup by abdo last updated on 08/May/20

i will post snswer by i cant complet it

$${i}\:{will}\:{post}\:{snswer}\:{by}\:{i}\:{cant}\:{complet} \\ $$$${it} \\ $$

Commented by abdomathmax last updated on 08/May/20

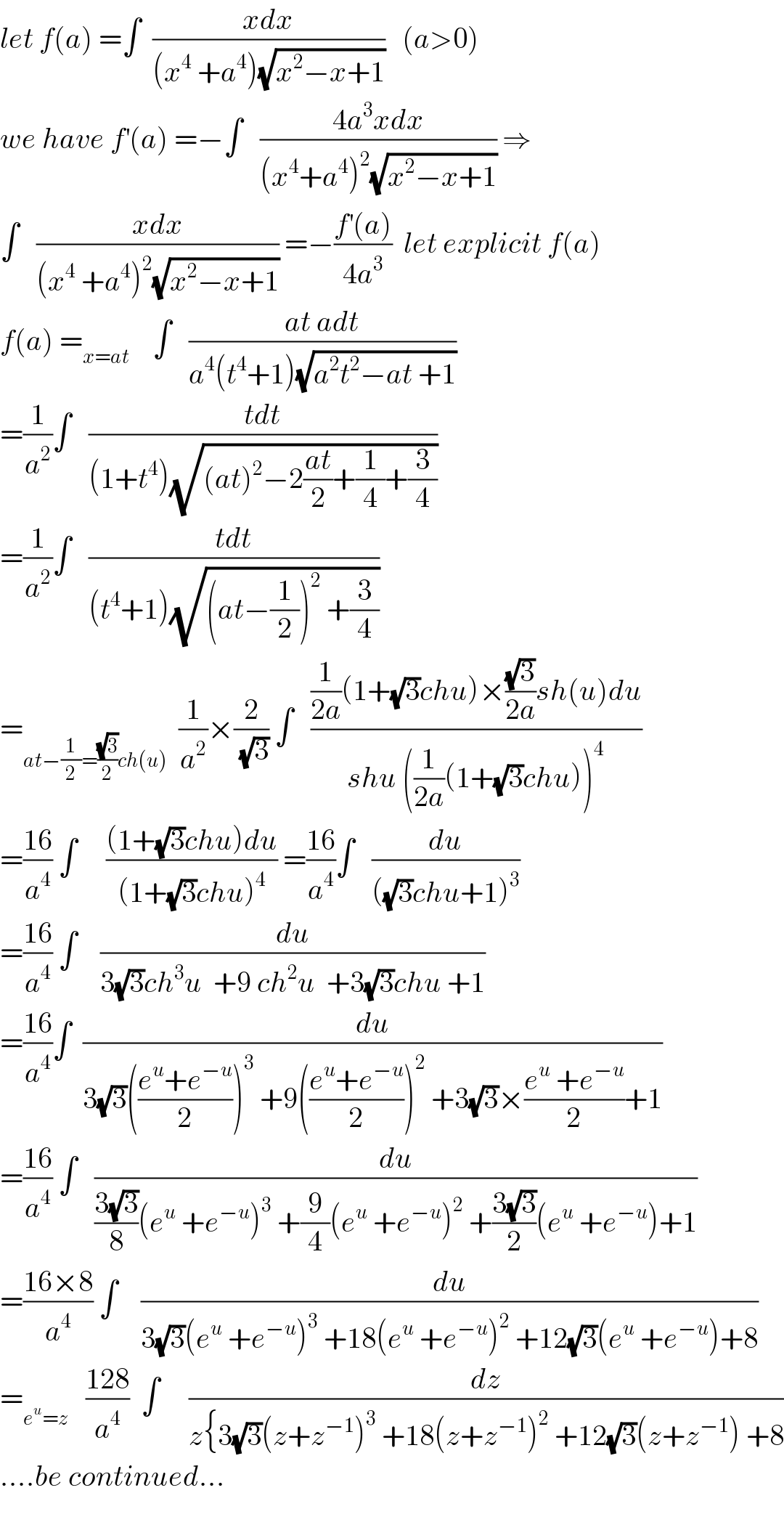
let f(a) =∫ ((xdx)/((x^4 +a^4 )(√(x^2 −x+1)))) (a>0) we have f^′ (a) =−∫ ((4a^3 xdx)/((x^4 +a^4 )^2 (√(x^2 −x+1)))) ⇒ ∫ ((xdx)/((x^4 +a^4 )^2 (√(x^2 −x+1)))) =−((f^′ (a))/(4a^3 )) let explicit f(a) f(a) =_(x=at) ∫ ((at adt)/(a^4 (t^4 +1)(√(a^2 t^2 −at +1)))) =(1/a^2 )∫ ((tdt)/((1+t^4 )(√((at)^2 −2((at)/2)+(1/4)+(3/4))))) =(1/a^2 )∫ ((tdt)/((t^4 +1)(√((at−(1/2))^2 +(3/4))))) =_(at−(1/2)=((√3)/2)ch(u)) (1/a^2 )×(2/(√3)) ∫ (((1/(2a))(1+(√3)chu)×((√3)/(2a))sh(u)du)/(shu ((1/(2a))(1+(√3)chu))^4 )) =((16)/a^4 ) ∫ (((1+(√3)chu)du)/((1+(√3)chu)^4 )) =((16)/a^4 )∫ (du/(((√3)chu+1)^3 )) =((16)/a^4 ) ∫ (du/(3(√3)ch^3 u +9 ch^2 u +3(√3)chu +1)) =((16)/a^4 )∫ (du/(3(√3)(((e^u +e^(−u) )/2))^3 +9(((e^u +e^(−u) )/2))^2 +3(√3)×((e^u +e^(−u) )/2)+1)) =((16)/a^4 ) ∫ (du/(((3(√3))/8)(e^u +e^(−u) )^3 +(9/4)(e^u +e^(−u) )^2 +((3(√3))/2)(e^u +e^(−u) )+1)) =((16×8)/a^4 ) ∫ (du/(3(√3)(e^u +e^(−u) )^3 +18(e^u +e^(−u) )^2 +12(√3)(e^u +e^(−u) )+8)) =_(e^u =z) ((128)/a^4 ) ∫ (dz/(z{3(√3)(z+z^(−1) )^3 +18(z+z^(−1) )^2 +12(√3)(z+z^(−1) ) +8)) ....be continued...

$${let}\:{f}\left({a}\right)\:=\int\:\:\frac{{xdx}}{\left({x}^{\mathrm{4}} \:+{a}^{\mathrm{4}} \right)\sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\:\:\:\left({a}>\mathrm{0}\right) \\ $$$${we}\:{have}\:{f}^{'} \left({a}\right)\:=−\int\:\:\:\frac{\mathrm{4}{a}^{\mathrm{3}} {xdx}}{\left({x}^{\mathrm{4}} +{a}^{\mathrm{4}} \right)^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\:\Rightarrow \\ $$$$\int\:\:\:\frac{{xdx}}{\left({x}^{\mathrm{4}} \:+{a}^{\mathrm{4}} \right)^{\mathrm{2}} \sqrt{{x}^{\mathrm{2}} −{x}+\mathrm{1}}}\:=−\frac{{f}^{'} \left({a}\right)}{\mathrm{4}{a}^{\mathrm{3}} }\:\:{let}\:{explicit}\:{f}\left({a}\right) \\ $$$${f}\left({a}\right)\:=_{{x}={at}} \:\:\:\:\int\:\:\:\frac{{at}\:{adt}}{{a}^{\mathrm{4}} \left({t}^{\mathrm{4}} +\mathrm{1}\right)\sqrt{{a}^{\mathrm{2}} {t}^{\mathrm{2}} −{at}\:+\mathrm{1}}} \\ $$$$=\frac{\mathrm{1}}{{a}^{\mathrm{2}} }\int\:\:\:\frac{{tdt}}{\left(\mathrm{1}+{t}^{\mathrm{4}} \right)\sqrt{\left({at}\right)^{\mathrm{2}} −\mathrm{2}\frac{{at}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$$$=\frac{\mathrm{1}}{{a}^{\mathrm{2}} }\int\:\:\:\frac{{tdt}}{\left({t}^{\mathrm{4}} +\mathrm{1}\right)\sqrt{\left({at}−\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} \:+\frac{\mathrm{3}}{\mathrm{4}}}} \\ $$$$=_{{at}−\frac{\mathrm{1}}{\mathrm{2}}=\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{ch}\left({u}\right)} \:\:\frac{\mathrm{1}}{{a}^{\mathrm{2}} }×\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}\:\int\:\:\:\frac{\frac{\mathrm{1}}{\mathrm{2}{a}}\left(\mathrm{1}+\sqrt{\mathrm{3}}{chu}\right)×\frac{\sqrt{\mathrm{3}}}{\mathrm{2}{a}}{sh}\left({u}\right){du}}{{shu}\:\left(\frac{\mathrm{1}}{\mathrm{2}{a}}\left(\mathrm{1}+\sqrt{\mathrm{3}}{chu}\right)\right)^{\mathrm{4}} } \\ $$$$=\frac{\mathrm{16}}{{a}^{\mathrm{4}} }\:\int\:\:\:\:\:\frac{\left(\mathrm{1}+\sqrt{\mathrm{3}}{chu}\right){du}}{\left(\mathrm{1}+\sqrt{\mathrm{3}}{chu}\right)^{\mathrm{4}} }\:=\frac{\mathrm{16}}{{a}^{\mathrm{4}} }\int\:\:\:\frac{{du}}{\left(\sqrt{\mathrm{3}}{chu}+\mathrm{1}\right)^{\mathrm{3}} } \\ $$$$=\frac{\mathrm{16}}{{a}^{\mathrm{4}} }\:\int\:\:\:\:\frac{{du}}{\mathrm{3}\sqrt{\mathrm{3}}{ch}^{\mathrm{3}} {u}\:\:+\mathrm{9}\:{ch}^{\mathrm{2}} {u}\:\:+\mathrm{3}\sqrt{\mathrm{3}}{chu}\:+\mathrm{1}} \\ $$$$=\frac{\mathrm{16}}{{a}^{\mathrm{4}} }\int\:\:\frac{{du}}{\mathrm{3}\sqrt{\mathrm{3}}\left(\frac{{e}^{{u}} +{e}^{−{u}} }{\mathrm{2}}\right)^{\mathrm{3}} \:+\mathrm{9}\left(\frac{{e}^{{u}} +{e}^{−{u}} }{\mathrm{2}}\right)^{\mathrm{2}} \:+\mathrm{3}\sqrt{\mathrm{3}}×\frac{{e}^{{u}} \:+{e}^{−{u}} }{\mathrm{2}}+\mathrm{1}} \\ $$$$=\frac{\mathrm{16}}{{a}^{\mathrm{4}} }\:\int\:\:\:\frac{{du}}{\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{8}}\left({e}^{{u}} \:+{e}^{−{u}} \right)^{\mathrm{3}} \:+\frac{\mathrm{9}}{\mathrm{4}}\left({e}^{{u}} \:+{e}^{−{u}} \right)^{\mathrm{2}} \:+\frac{\mathrm{3}\sqrt{\mathrm{3}}}{\mathrm{2}}\left({e}^{{u}} \:+{e}^{−{u}} \right)+\mathrm{1}} \\ $$$$=\frac{\mathrm{16}×\mathrm{8}}{{a}^{\mathrm{4}} }\:\int\:\:\:\:\frac{{du}}{\mathrm{3}\sqrt{\mathrm{3}}\left({e}^{{u}} \:+{e}^{−{u}} \right)^{\mathrm{3}} \:+\mathrm{18}\left({e}^{{u}} \:+{e}^{−{u}} \right)^{\mathrm{2}} \:+\mathrm{12}\sqrt{\mathrm{3}}\left({e}^{{u}} \:+{e}^{−{u}} \right)+\mathrm{8}} \\ $$$$=_{{e}^{{u}} ={z}} \:\:\:\frac{\mathrm{128}}{{a}^{\mathrm{4}} }\:\:\int\:\:\:\:\:\frac{{dz}}{{z}\left\{\mathrm{3}\sqrt{\mathrm{3}}\left({z}+{z}^{−\mathrm{1}} \right)^{\mathrm{3}} \:+\mathrm{18}\left({z}+{z}^{−\mathrm{1}} \right)^{\mathrm{2}} \:+\mathrm{12}\sqrt{\mathrm{3}}\left({z}+{z}^{−\mathrm{1}} \right)\:+\mathrm{8}\right.} \\ $$$$....{be}\:{continued}... \\ $$$$ \\ $$

Commented by  M±th+et+s last updated on 08/May/20

its nice to see you again in the forum sir prakash jain

$${its}\:{nice}\:{to}\:{see}\:{you}\:{again}\:{in}\:{the}\:{forum} \\ $$$${sir}\:{prakash}\:{jain} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com