Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 92936 by mathmax by abdo last updated on 09/May/20

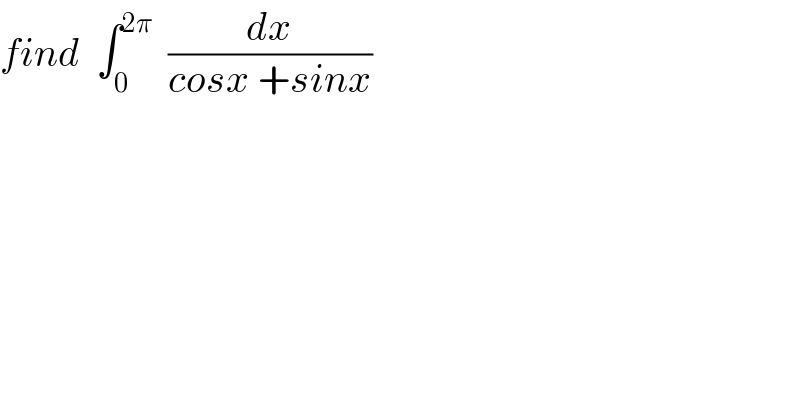
find ∫_0 ^(2π) (dx/(cosx +sinx))

$${find}\:\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{dx}}{{cosx}\:+{sinx}} \\ $$

Commented by abdomathmax last updated on 10/May/20

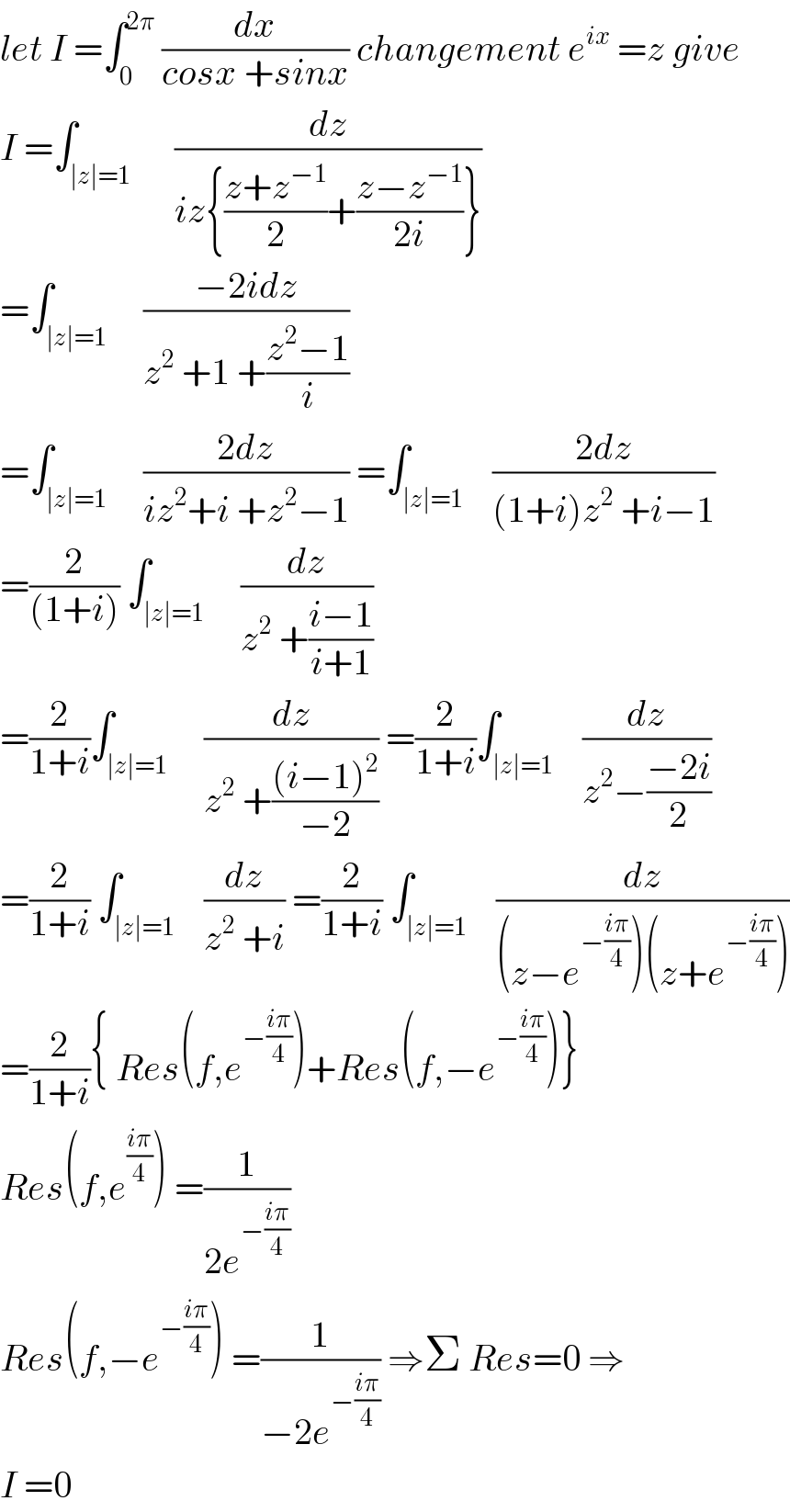
let I =∫_0 ^(2π) (dx/(cosx +sinx)) changement e^(ix) =z give I =∫_(∣z∣=1) (dz/(iz{((z+z^(−1) )/2)+((z−z^(−1) )/(2i))})) =∫_(∣z∣=1) ((−2idz)/(z^2 +1 +((z^2 −1)/i))) =∫_(∣z∣=1) ((2dz)/(iz^2 +i +z^2 −1)) =∫_(∣z∣=1) ((2dz)/((1+i)z^2 +i−1)) =(2/((1+i))) ∫_(∣z∣=1) (dz/(z^2 +((i−1)/(i+1)))) =(2/(1+i))∫_(∣z∣=1) (dz/(z^2 +(((i−1)^2 )/(−2)))) =(2/(1+i))∫_(∣z∣=1) (dz/(z^2 −((−2i)/2))) =(2/(1+i)) ∫_(∣z∣=1) (dz/(z^2 +i)) =(2/(1+i)) ∫_(∣z∣=1) (dz/((z−e^(−((iπ)/4)) )(z+e^(−((iπ)/4)) ))) =(2/(1+i)){ Res(f,e^(−((iπ)/4)) )+Res(f,−e^(−((iπ)/4)) )} Res(f,e^((iπ)/4) ) =(1/(2e^(−((iπ)/4)) )) Res(f,−e^(−((iπ)/4)) ) =(1/(−2e^(−((iπ)/4)) )) ⇒Σ Res=0 ⇒ I =0

$${let}\:{I}\:=\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\frac{{dx}}{{cosx}\:+{sinx}}\:{changement}\:{e}^{{ix}} \:={z}\:{give} \\ $$$${I}\:=\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\frac{{dz}}{{iz}\left\{\frac{{z}+{z}^{−\mathrm{1}} }{\mathrm{2}}+\frac{{z}−{z}^{−\mathrm{1}} }{\mathrm{2}{i}}\right\}} \\ $$$$=\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\frac{−\mathrm{2}{idz}}{{z}^{\mathrm{2}} \:+\mathrm{1}\:+\frac{{z}^{\mathrm{2}} −\mathrm{1}}{{i}}}\: \\ $$$$=\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\frac{\mathrm{2}{dz}}{{iz}^{\mathrm{2}} +{i}\:+{z}^{\mathrm{2}} −\mathrm{1}}\:=\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\frac{\mathrm{2}{dz}}{\left(\mathrm{1}+{i}\right){z}^{\mathrm{2}} \:+{i}−\mathrm{1}} \\ $$$$=\frac{\mathrm{2}}{\left(\mathrm{1}+{i}\right)}\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\frac{{dz}}{{z}^{\mathrm{2}} \:+\frac{{i}−\mathrm{1}}{{i}+\mathrm{1}}} \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}+{i}}\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\frac{{dz}}{{z}^{\mathrm{2}} \:+\frac{\left({i}−\mathrm{1}\right)^{\mathrm{2}} }{−\mathrm{2}}}\:=\frac{\mathrm{2}}{\mathrm{1}+{i}}\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\frac{{dz}}{{z}^{\mathrm{2}} −\frac{−\mathrm{2}{i}}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}+{i}}\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\frac{{dz}}{{z}^{\mathrm{2}} \:+{i}}\:=\frac{\mathrm{2}}{\mathrm{1}+{i}}\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\frac{{dz}}{\left({z}−{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)\left({z}+{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)} \\ $$$$=\frac{\mathrm{2}}{\mathrm{1}+{i}}\left\{\:{Res}\left({f},{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)+{Res}\left({f},−{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)\right\} \\ $$$${Res}\left({f},{e}^{\frac{{i}\pi}{\mathrm{4}}} \right)\:=\frac{\mathrm{1}}{\mathrm{2}{e}^{−\frac{{i}\pi}{\mathrm{4}}} } \\ $$$${Res}\left({f},−{e}^{−\frac{{i}\pi}{\mathrm{4}}} \right)\:=\frac{\mathrm{1}}{−\mathrm{2}{e}^{−\frac{{i}\pi}{\mathrm{4}}} }\:\Rightarrow\Sigma\:{Res}=\mathrm{0}\:\Rightarrow \\ $$$${I}\:=\mathrm{0} \\ $$

Commented by i jagooll last updated on 10/May/20

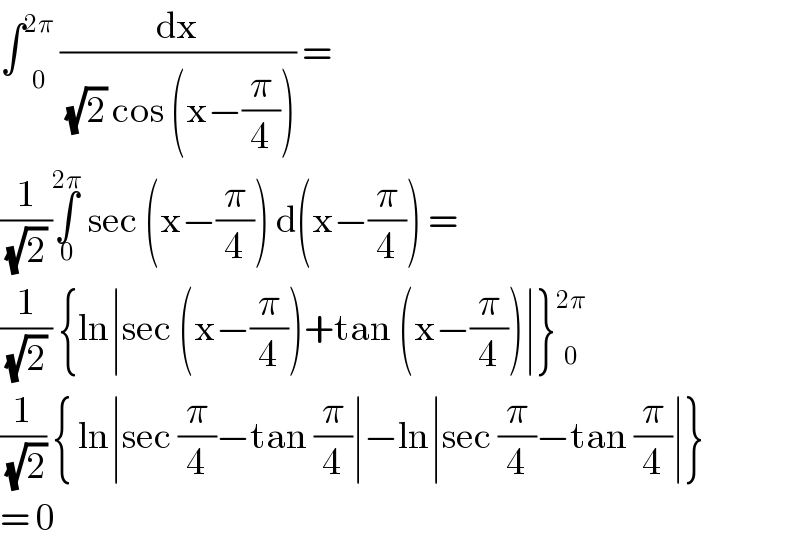
∫ _0 ^(2π) (dx/((√2) cos (x−(π/4)))) = (1/((√2) ))∫_0 ^(2π) sec (x−(π/4)) d(x−(π/4)) = (1/((√2) )) {ln∣sec (x−(π/4))+tan (x−(π/4))∣} _0 ^(2π) (1/(√2)) { ln∣sec (π/4)−tan (π/4)∣−ln∣sec (π/4)−tan (π/4)∣} = 0

$$\int\underset{\mathrm{0}} {\overset{\mathrm{2}\pi} {\:}}\:\frac{\mathrm{dx}}{\sqrt{\mathrm{2}}\:\mathrm{cos}\:\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)}\:=\: \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{2}}\:}\underset{\mathrm{0}} {\overset{\mathrm{2}\pi} {\int}}\:\mathrm{sec}\:\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)\:\mathrm{d}\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)\:=\: \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{2}}\:}\:\left\{\mathrm{ln}\mid\mathrm{sec}\:\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)+\mathrm{tan}\:\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)\mid\right\}\underset{\mathrm{0}} {\overset{\mathrm{2}\pi} {\:}} \\ $$$$\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\left\{\:\mathrm{ln}\mid\mathrm{sec}\:\frac{\pi}{\mathrm{4}}−\mathrm{tan}\:\frac{\pi}{\mathrm{4}}\mid−\mathrm{ln}\mid\mathrm{sec}\:\frac{\pi}{\mathrm{4}}−\mathrm{tan}\:\frac{\pi}{\mathrm{4}}\mid\right\} \\ $$$$=\:\mathrm{0}\: \\ $$

Answered by niroj last updated on 09/May/20

I= ∫_0 ^( 2π) (( dx)/(cos x+sin x)) let , sin x= ((2tan (x/2))/(1+tan^2 (x/2))) cos x= ((1−tan^2 (x/2))/(1+tan^2 (x/2))) [ ∫ (( 1)/(((1−tan^2 (x/2))/(1+tan^2 (x/2))) + ((2tan (x/2))/(1+ tan^2 (x/2)))))dx]_0 ^(2π) = [ ∫ (( sec^2 (x/2)dx)/(1−tan^2 (x/2) +2tan(x/2)))]_0 ^(2π) Put, tan (x/2)= t sec^2 (x/2)dx=2dt [ ∫ ((2 dt)/(1−t^2 +2t)) ]_0 ^(2π) = [−2 ∫ (1/(t^2 −2t−1))dt]_0 ^(2π) =[−2∫ (1/((t)^2 −2.t.1+(1)^2 −2))dt]_0 ^(2π) = [ −2∫ (1/((t−1)^2 −2))]_0 ^(2π) = [ 2∫ (1/(((√2) )^2 −(t−1)^2 ))]_0 ^(2π) =[ 2 .(1/(2.(√2)))log (( (√2)+(t−1))/( (√2) −(t−1)))]_0 ^(2π) = [ (1/(√2)) log (( (√2) +(tan(x/2)−1))/( (√2) −(tan(x/2) −1)))]_0 ^(2π) = [ (1/(√2)) log (( (√2) + (tan((2π)/2)−1))/( (√2) −(tan ((2π)/2)−1))) −(1/(√2))log (( (√2)+(0−1))/( (√2) −(0−1)))] = (1/(√2)) log (( (√2) −1)/( (√2) +1)) −(1/(√2)) log (( (√2)−1)/( (√2) +1)) =0 //.

$$\:\:\mathrm{I}=\:\int_{\mathrm{0}} ^{\:\mathrm{2}\pi} \:\:\frac{\:\:\boldsymbol{\mathrm{dx}}}{\boldsymbol{\mathrm{cos}}\:\mathrm{x}+\mathrm{sin}\:\mathrm{x}} \\ $$$$\:\:\:\:\mathrm{let}\:,\:\:\mathrm{sin}\:\mathrm{x}=\:\frac{\mathrm{2tan}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}} \\ $$$$\:\:\:\:\mathrm{cos}\:\mathrm{x}=\:\frac{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}} \\ $$$$\:\:\left[\:\int\:\:\frac{\:\mathrm{1}}{\frac{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}\:\:+\:\frac{\mathrm{2tan}\:\frac{\mathrm{x}}{\mathrm{2}}}{\mathrm{1}+\:\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}}}\mathrm{dx}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\:\left[\:\int\:\frac{\:\:\mathrm{sec}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}}{\mathrm{1}−\mathrm{tan}^{\mathrm{2}} \frac{\mathrm{x}}{\mathrm{2}}\:+\mathrm{2tan}\frac{\mathrm{x}}{\mathrm{2}}}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:\:\mathrm{Put},\:\:\mathrm{tan}\:\frac{\mathrm{x}}{\mathrm{2}}=\:\mathrm{t} \\ $$$$\:\:\:\:\mathrm{sec}^{\mathrm{2}} \:\frac{\mathrm{x}}{\mathrm{2}}\mathrm{dx}=\mathrm{2dt} \\ $$$$\:\:\left[\:\int\:\frac{\mathrm{2}\:\mathrm{dt}}{\mathrm{1}−\mathrm{t}^{\mathrm{2}} +\mathrm{2t}}\:\:\right]_{\mathrm{0}} ^{\mathrm{2}\pi} =\:\left[−\mathrm{2}\:\int\:\frac{\mathrm{1}}{\mathrm{t}^{\mathrm{2}} −\mathrm{2t}−\mathrm{1}}\mathrm{dt}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\left[−\mathrm{2}\int\:\frac{\mathrm{1}}{\left(\mathrm{t}\right)^{\mathrm{2}} −\mathrm{2}.\mathrm{t}.\mathrm{1}+\left(\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}}\mathrm{dt}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\:\left[\:−\mathrm{2}\int\:\frac{\mathrm{1}}{\left(\mathrm{t}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{2}}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\:\left[\:\mathrm{2}\int\:\frac{\mathrm{1}}{\left(\sqrt{\mathrm{2}}\:\right)^{\mathrm{2}} −\left(\mathrm{t}−\mathrm{1}\right)^{\mathrm{2}} }\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\left[\:\mathrm{2}\:.\frac{\mathrm{1}}{\mathrm{2}.\sqrt{\mathrm{2}}}\mathrm{log}\:\frac{\:\:\sqrt{\mathrm{2}}+\left(\mathrm{t}−\mathrm{1}\right)}{\:\sqrt{\mathrm{2}}\:−\left(\mathrm{t}−\mathrm{1}\right)}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\:\:\left[\:\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\mathrm{log}\:\:\frac{\:\sqrt{\mathrm{2}}\:+\left(\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}−\mathrm{1}\right)}{\:\sqrt{\mathrm{2}}\:−\left(\mathrm{tan}\frac{\mathrm{x}}{\mathrm{2}}\:−\mathrm{1}\right)}\right]_{\mathrm{0}} ^{\mathrm{2}\pi} \\ $$$$\:=\:\left[\:\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\mathrm{log}\:\frac{\:\sqrt{\mathrm{2}}\:\:+\:\left(\mathrm{tan}\frac{\mathrm{2}\pi}{\mathrm{2}}−\mathrm{1}\right)}{\:\:\sqrt{\mathrm{2}}\:−\left(\mathrm{tan}\:\frac{\mathrm{2}\pi}{\mathrm{2}}−\mathrm{1}\right)}\:−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\mathrm{log}\:\frac{\:\:\sqrt{\mathrm{2}}+\left(\mathrm{0}−\mathrm{1}\right)}{\:\sqrt{\mathrm{2}}\:−\left(\mathrm{0}−\mathrm{1}\right)}\right] \\ $$$$\:=\:\:\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\mathrm{log}\:\frac{\:\sqrt{\mathrm{2}}\:−\mathrm{1}}{\:\:\sqrt{\mathrm{2}}\:\:\:\:+\mathrm{1}}\:−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\:\mathrm{log}\:\frac{\:\sqrt{\mathrm{2}}−\mathrm{1}}{\:\sqrt{\mathrm{2}}\:+\mathrm{1}}\:=\mathrm{0}\://. \\ $$$$ \\ $$$$\: \\ $$

Commented by mathmax by abdo last updated on 10/May/20

thankx sir.

$${thankx}\:{sir}. \\ $$

Commented by i jagooll last updated on 10/May/20

������

Answered by Ar Brandon last updated on 10/May/20

∫_0 ^(2π) (dx/(cosx +sinx)) I=(1/(√2))∫_0 ^(2π) (1/(cos(x−(π/4))))dx ⇒I=_(u=x−(π/4)) (1/(√2))∫_(−(π/4)) ^((7π)/4) (1/(cos u))du ⇒I=(1/(√2))[ln∣sec u+tan u∣]_(−(π/4)) ^((7π)/4) ⇒I=(1/(√2))[ln∣−(√2)+1∣−ln∣(√2)−1∣] ∫_0 ^(2π) (dx/(cosx +sinx))=((√2)/2)ln∣(((√2)−1)/((√2)−1))∣=0

$$\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{dx}}{{cosx}\:+{sinx}} \\ $$$$\mathrm{I}=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int_{\mathrm{0}} ^{\mathrm{2}\pi} \frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{x}−\frac{\pi}{\mathrm{4}}\right)}\mathrm{dx} \\ $$$$\Rightarrow\mathrm{I}\underset{\mathrm{u}=\mathrm{x}−\frac{\pi}{\mathrm{4}}} {=}\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\mathrm{7}\pi}{\mathrm{4}}} \frac{\mathrm{1}}{\mathrm{cos}\:\mathrm{u}}\mathrm{du} \\ $$$$\Rightarrow\mathrm{I}=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\left[\mathrm{ln}\mid\mathrm{sec}\:\mathrm{u}+\mathrm{tan}\:\mathrm{u}\mid\right]_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\mathrm{7}\pi}{\mathrm{4}}} \\ $$$$\Rightarrow\mathrm{I}=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\left[\mathrm{ln}\mid−\sqrt{\mathrm{2}}+\mathrm{1}\mid−\mathrm{ln}\mid\sqrt{\mathrm{2}}−\mathrm{1}\mid\right] \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{dx}}{{cosx}\:+{sinx}}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\mathrm{ln}\mid\frac{\sqrt{\mathrm{2}}−\mathrm{1}}{\sqrt{\mathrm{2}}−\mathrm{1}}\mid=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com