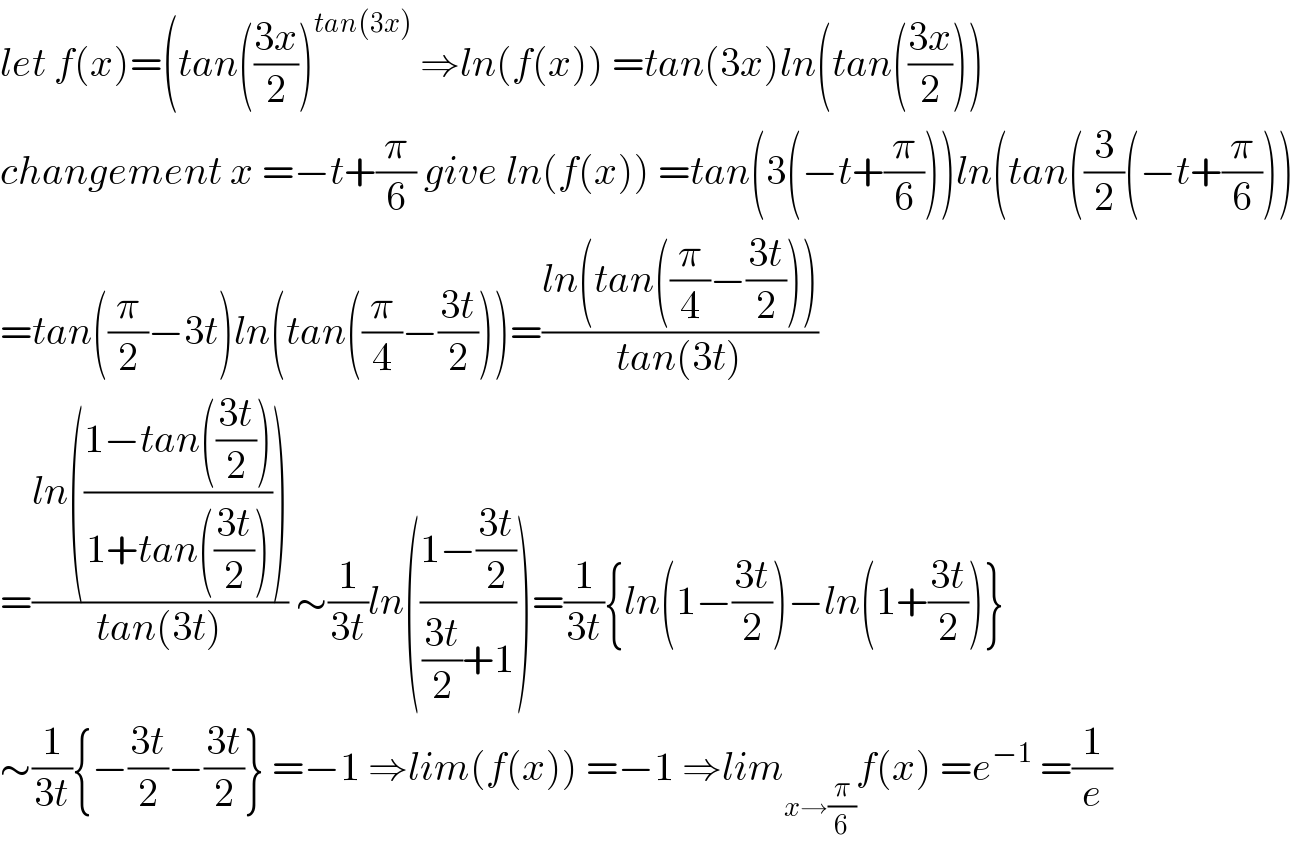lim_(x→π/6) (tan (((3x)/2)))^(tan (3x)) =?


Question and Answers Forum

All Questions Topic List
Limits Questions
Previous in All Question Next in All Question
Previous in Limits Next in Limits
Question Number 93033 by i jagooll last updated on 10/May/20

$\lim_{x \to π / 6} {(\tan (\frac{3 x}{2}))}^{\tan (3 x)} = ?$
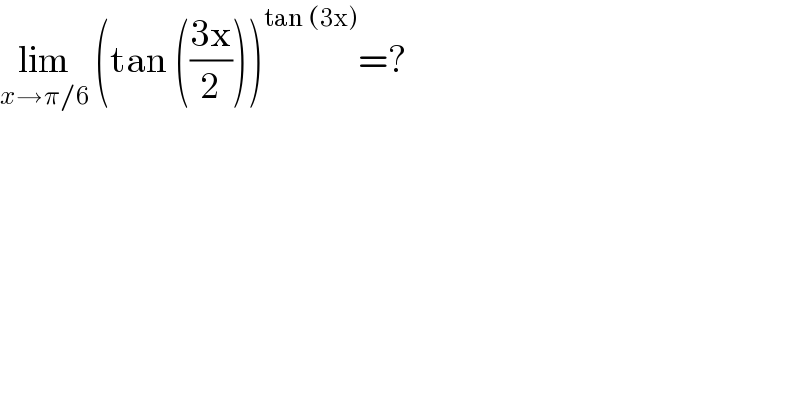
Commented by john santu last updated on 10/May/20

$let \frac{3 x}{2} = t \Rightarrow 3 x = 2 t$ $with t \to \frac{π}{4}$ $\lim_{t \to π / 4} {(\tan t)}^{\tan 2 t} =$ $\lim_{t \to π / 4} \ln y = \lim_{t \to π / 4} \tan 2 t \ln (\tan t)$ $\lim_{t \to π / 4} \ln y = \lim_{t \to π / 4} \frac{\ln (\tan t)}{\cot 2 t}$ $= \lim_{t \to π / 4} \frac{\sec^{2} t}{\tan t} \times (- \frac{\sin^{2} 2 t}{2})$ $= - \frac{1}{2} \times \frac{2}{1} = - 1 .$ $then \lim_{x \to 3 π / 6} {(\tan (\frac{3 x}{2}))}^{\tan (3 x)}$ $= e^{- 1} = \frac{1}{e}$
Commented by i jagooll last updated on 10/May/20
good sir...
Commented by mathmax by abdo last updated on 11/May/20
$let f(x)=(tan(((3x)/2))^(tan(3x)) ⇒ln(f(x)) =tan(3x)ln(tan(((3x)/2))) changement x =−t+(π/6) give ln(f(x)) =tan(3(−t+(π/6)))ln(tan((3/2)(−t+(π/6))) =tan((π/2)−3t)ln(tan((π/4)−((3t)/2)))=((ln(tan((π/4)−((3t)/2))))/(tan(3t))) =((ln(((1−tan(((3t)/2)))/(1+tan(((3t)/2))))))/(tan(3t))) ∼(1/(3t))ln(((1−((3t)/2))/(((3t)/2)+1)))=(1/(3t)){ln(1−((3t)/2))−ln(1+((3t)/2))} ∼(1/(3t)){−((3t)/2)−((3t)/2)} =−1 ⇒lim(f(x)) =−1 ⇒lim_(x→(π/6)) f(x) =e^(−1) =(1/e)$
$l e t f (x) = (t a n {(\frac{3 x}{2})}^{t a n (3 x)} \Rightarrow l n (f (x)) = t a n (3 x) l n (t a n (\frac{3 x}{2}))$ $c h a n g e m e n t x = - t + \frac{π}{6} g i v e l n (f (x)) = t a n (3 (- t + \frac{π}{6})) l n (t a n (\frac{3}{2} (- t + \frac{π}{6}))$ $= t a n (\frac{π}{2} - 3 t) l n (t a n (\frac{π}{4} - \frac{3 t}{2})) = \frac{l n (t a n (\frac{π}{4} - \frac{3 t}{2}))}{t a n (3 t)}$ $= \frac{l n (\frac{1 - t a n (\frac{3 t}{2})}{1 + t a n (\frac{3 t}{2})})}{t a n (3 t)} \sim \frac{1}{3 t} l n (\frac{1 - \frac{3 t}{2}}{\frac{3 t}{2} + 1}) = \frac{1}{3 t} {l n (1 - \frac{3 t}{2}) - l n (1 + \frac{3 t}{2})}$ $\sim \frac{1}{3 t} {- \frac{3 t}{2} - \frac{3 t}{2}} = - 1 \Rightarrow l i m (f (x)) = - 1 \Rightarrow {l i m}_{x \to \frac{π}{6}} f (x) = e^{- 1} = \frac{1}{e}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum