
Question and Answers Forum
Question Number 93892 by frc2crc last updated on 15/May/20
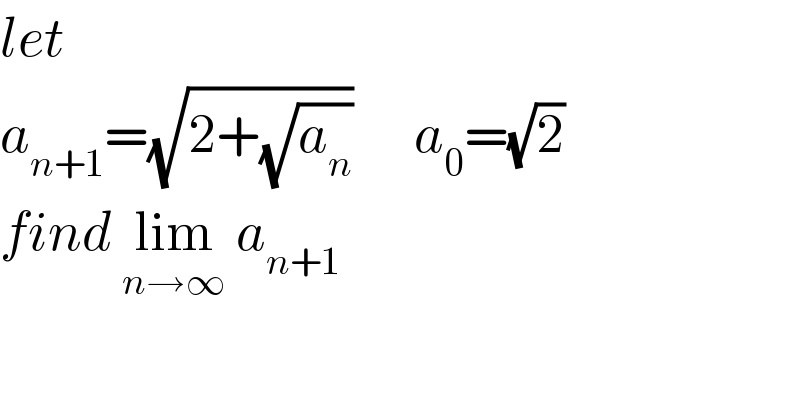
Commented by Tony Lin last updated on 16/May/20

Commented by Tony Lin last updated on 16/May/20

Commented by frc2crc last updated on 16/May/20

| ||
Question and Answers Forum | ||
Question Number 93892 by frc2crc last updated on 15/May/20 | ||
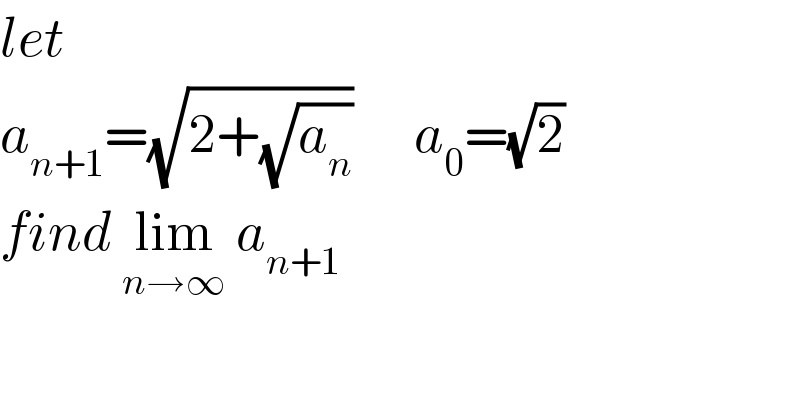 | ||
Commented by Tony Lin last updated on 16/May/20 | ||
 | ||
Commented by Tony Lin last updated on 16/May/20 | ||
 | ||
Commented by frc2crc last updated on 16/May/20 | ||
 | ||