Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 9439 by tawakalitu last updated on 08/Dec/16

Solve for x , y and z (x + 1)(y + 3) = 8 ....... (i) (y + 3)(z − 1) = 3 ......... (ii) (z − 1)(x + 1) = 2 ......... (iii)

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{x}\:,\:\mathrm{y}\:\mathrm{and}\:\mathrm{z} \\ $$$$\left(\mathrm{x}\:+\:\mathrm{1}\right)\left(\mathrm{y}\:+\:\mathrm{3}\right)\:=\:\mathrm{8}\:\:\:.......\:\left(\mathrm{i}\right) \\ $$$$\left(\mathrm{y}\:+\:\mathrm{3}\right)\left(\mathrm{z}\:−\:\mathrm{1}\right)\:=\:\mathrm{3}\:\:.........\:\left(\mathrm{ii}\right) \\ $$$$\left(\mathrm{z}\:−\:\mathrm{1}\right)\left(\mathrm{x}\:+\:\mathrm{1}\right)\:=\:\mathrm{2}\:\:\:.........\:\left(\mathrm{iii}\right) \\ $$

Commented by RasheedSoomro last updated on 09/Dec/16

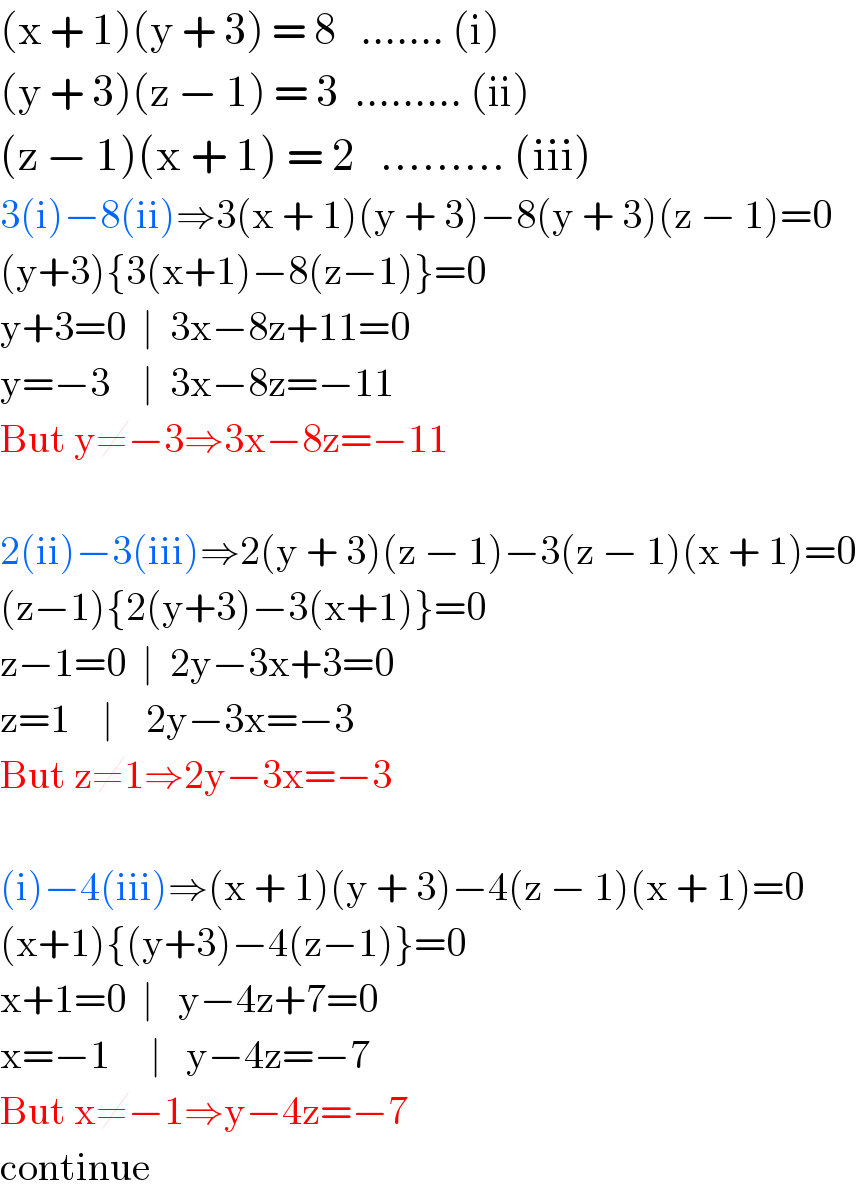
(x + 1)(y + 3) = 8 ....... (i) (y + 3)(z − 1) = 3 ......... (ii) (z − 1)(x + 1) = 2 ......... (iii) 3(i)−8(ii)⇒3(x + 1)(y + 3)−8(y + 3)(z − 1)=0 (y+3){3(x+1)−8(z−1)}=0 y+3=0 ∣ 3x−8z+11=0 y=−3 ∣ 3x−8z=−11 But y≠−3⇒3x−8z=−11 2(ii)−3(iii)⇒2(y + 3)(z − 1)−3(z − 1)(x + 1)=0 (z−1){2(y+3)−3(x+1)}=0 z−1=0 ∣ 2y−3x+3=0 z=1 ∣ 2y−3x=−3 But z≠1⇒2y−3x=−3 (i)−4(iii)⇒(x + 1)(y + 3)−4(z − 1)(x + 1)=0 (x+1){(y+3)−4(z−1)}=0 x+1=0 ∣ y−4z+7=0 x=−1 ∣ y−4z=−7 But x≠−1⇒y−4z=−7 continue

$$\left(\mathrm{x}\:+\:\mathrm{1}\right)\left(\mathrm{y}\:+\:\mathrm{3}\right)\:=\:\mathrm{8}\:\:\:.......\:\left(\mathrm{i}\right) \\ $$$$\left(\mathrm{y}\:+\:\mathrm{3}\right)\left(\mathrm{z}\:−\:\mathrm{1}\right)\:=\:\mathrm{3}\:\:.........\:\left(\mathrm{ii}\right) \\ $$$$\left(\mathrm{z}\:−\:\mathrm{1}\right)\left(\mathrm{x}\:+\:\mathrm{1}\right)\:=\:\mathrm{2}\:\:\:.........\:\left(\mathrm{iii}\right) \\ $$$$\mathrm{3}\left(\mathrm{i}\right)−\mathrm{8}\left(\mathrm{ii}\right)\Rightarrow\mathrm{3}\left(\mathrm{x}\:+\:\mathrm{1}\right)\left(\mathrm{y}\:+\:\mathrm{3}\right)−\mathrm{8}\left(\mathrm{y}\:+\:\mathrm{3}\right)\left(\mathrm{z}\:−\:\mathrm{1}\right)=\mathrm{0} \\ $$$$\left(\mathrm{y}+\mathrm{3}\right)\left\{\mathrm{3}\left(\mathrm{x}+\mathrm{1}\right)−\mathrm{8}\left(\mathrm{z}−\mathrm{1}\right)\right\}=\mathrm{0} \\ $$$$\mathrm{y}+\mathrm{3}=\mathrm{0}\:\:\mid\:\:\mathrm{3x}−\mathrm{8z}+\mathrm{11}=\mathrm{0} \\ $$$$\mathrm{y}=−\mathrm{3}\:\:\:\:\mid\:\:\mathrm{3x}−\mathrm{8z}=−\mathrm{11} \\ $$$$\mathrm{But}\:\mathrm{y}\neq−\mathrm{3}\Rightarrow\mathrm{3x}−\mathrm{8z}=−\mathrm{11} \\ $$$$ \\ $$$$\mathrm{2}\left(\mathrm{ii}\right)−\mathrm{3}\left(\mathrm{iii}\right)\Rightarrow\mathrm{2}\left(\mathrm{y}\:+\:\mathrm{3}\right)\left(\mathrm{z}\:−\:\mathrm{1}\right)−\mathrm{3}\left(\mathrm{z}\:−\:\mathrm{1}\right)\left(\mathrm{x}\:+\:\mathrm{1}\right)=\mathrm{0} \\ $$$$\left(\mathrm{z}−\mathrm{1}\right)\left\{\mathrm{2}\left(\mathrm{y}+\mathrm{3}\right)−\mathrm{3}\left(\mathrm{x}+\mathrm{1}\right)\right\}=\mathrm{0} \\ $$$$\mathrm{z}−\mathrm{1}=\mathrm{0}\:\:\mid\:\:\mathrm{2y}−\mathrm{3x}+\mathrm{3}=\mathrm{0} \\ $$$$\mathrm{z}=\mathrm{1}\:\:\:\:\mid\:\:\:\:\mathrm{2y}−\mathrm{3x}=−\mathrm{3} \\ $$$$\mathrm{But}\:\mathrm{z}\neq\mathrm{1}\Rightarrow\mathrm{2y}−\mathrm{3x}=−\mathrm{3} \\ $$$$ \\ $$$$\left(\mathrm{i}\right)−\mathrm{4}\left(\mathrm{iii}\right)\Rightarrow\left(\mathrm{x}\:+\:\mathrm{1}\right)\left(\mathrm{y}\:+\:\mathrm{3}\right)−\mathrm{4}\left(\mathrm{z}\:−\:\mathrm{1}\right)\left(\mathrm{x}\:+\:\mathrm{1}\right)=\mathrm{0} \\ $$$$\left(\mathrm{x}+\mathrm{1}\right)\left\{\left(\mathrm{y}+\mathrm{3}\right)−\mathrm{4}\left(\mathrm{z}−\mathrm{1}\right)\right\}=\mathrm{0} \\ $$$$\mathrm{x}+\mathrm{1}=\mathrm{0}\:\:\mid\:\:\:\mathrm{y}−\mathrm{4z}+\mathrm{7}=\mathrm{0} \\ $$$$\mathrm{x}=−\mathrm{1}\:\:\:\:\:\mid\:\:\:\mathrm{y}−\mathrm{4z}=−\mathrm{7} \\ $$$$\mathrm{But}\:\mathrm{x}\neq−\mathrm{1}\Rightarrow\mathrm{y}−\mathrm{4z}=−\mathrm{7} \\ $$$$\mathrm{continue} \\ $$

Answered by geovane10math last updated on 08/Dec/16

(y + 3) = (8/((x + 1))) (α) (8/(x + 1))(z − 1) = 3 (ii) (z − 1)(x + 1) = 2 (iii) (iii) : z − 1 = (2/(x + 1)) (β) (8/(x + 1)) ∙ (2/(x + 1)) = 3 (ii) ((16)/((x + 1)^2 )) = 3 ((16)/3) = (x + 1)^2 = x^2 + 2x + 1 x^2 + 2x + 1 = ((16)/3) x^2 + 2x + (3/3) − ((16)/3) = 0 x^2 + 2x − ((13)/3) = 0 Δ = 4 − 4(− ((13)/3)) = 4 + ((52)/3) = ((64)/3) x = ((−2 ± ((8(√3))/3))/2) x_1 = ((((8(√3))/3) − 2)/2) = ((2(((4(√3))/3) − 1))/2) = ((4(√3))/3) − 1 x_1 ≈ 1,3094 x_2 = ((−2 − ((8(√3))/3))/2) = ((2(−1 − ((4(√3))/3)))/2) = = −1 − ((4(√3))/3) = −(1 + ((4(√3))/3)) x_2 ≈ −3,3094 You can use x_1 and x_2 to find y_1 and y_2 in (α). You can use too to find z_1 and z_2 in (β)

$$\left({y}\:+\:\mathrm{3}\right)\:=\:\frac{\mathrm{8}}{\left({x}\:+\:\mathrm{1}\right)}\:\:\left(\alpha\right) \\ $$$$\frac{\mathrm{8}}{{x}\:+\:\mathrm{1}}\left({z}\:−\:\mathrm{1}\right)\:=\:\mathrm{3}\:\:\:\:\:\:\:\left({ii}\right) \\ $$$$\:\left({z}\:−\:\mathrm{1}\right)\left({x}\:+\:\mathrm{1}\right)\:=\:\mathrm{2}\:\:\:\:\:\left({iii}\right) \\ $$$$ \\ $$$$\left({iii}\right)\::\:{z}\:−\:\mathrm{1}\:=\:\frac{\mathrm{2}}{{x}\:+\:\mathrm{1}}\:\left(\beta\right) \\ $$$$\frac{\mathrm{8}}{{x}\:+\:\mathrm{1}}\:\centerdot\:\frac{\mathrm{2}}{{x}\:+\:\mathrm{1}}\:=\:\mathrm{3}\:\:\:\:\left({ii}\right) \\ $$$$\frac{\mathrm{16}}{\left({x}\:+\:\mathrm{1}\right)^{\mathrm{2}} }\:=\:\mathrm{3} \\ $$$$\frac{\mathrm{16}}{\mathrm{3}}\:=\:\left({x}\:+\:\mathrm{1}\right)^{\mathrm{2}} \:=\:{x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\:\mathrm{1} \\ $$$${x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:\:+\:\mathrm{1}\:=\:\frac{\mathrm{16}}{\mathrm{3}} \\ $$$${x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\:\frac{\mathrm{3}}{\mathrm{3}}\:−\:\frac{\mathrm{16}}{\mathrm{3}}\:=\:\mathrm{0} \\ $$$${x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:−\:\frac{\mathrm{13}}{\mathrm{3}}\:=\:\mathrm{0} \\ $$$$\Delta\:=\:\:\mathrm{4}\:−\:\mathrm{4}\left(−\:\frac{\mathrm{13}}{\mathrm{3}}\right)\:=\:\mathrm{4}\:+\:\frac{\mathrm{52}}{\mathrm{3}}\:=\:\frac{\mathrm{64}}{\mathrm{3}} \\ $$$${x}\:=\:\frac{−\mathrm{2}\:\pm\:\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{3}}}{\mathrm{2}}\:\:\:\: \\ $$$${x}_{\mathrm{1}} \:=\:\frac{\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{3}}\:−\:\mathrm{2}}{\mathrm{2}}\:=\:\frac{\mathrm{2}\left(\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\:−\:\mathrm{1}\right)}{\mathrm{2}}\:=\:\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\:−\:\mathrm{1} \\ $$$${x}_{\mathrm{1}} \:\approx\:\mathrm{1},\mathrm{3094} \\ $$$$ \\ $$$${x}_{\mathrm{2}} \:=\:\frac{−\mathrm{2}\:−\:\frac{\mathrm{8}\sqrt{\mathrm{3}}}{\mathrm{3}}}{\mathrm{2}}\:=\:\frac{\mathrm{2}\left(−\mathrm{1}\:−\:\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\right)}{\mathrm{2}}\:=\: \\ $$$$=\:−\mathrm{1}\:−\:\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\:=\:−\left(\mathrm{1}\:+\:\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\right)\: \\ $$$${x}_{\mathrm{2}} \:\approx\:−\mathrm{3},\mathrm{3094} \\ $$$$\mathrm{You}\:\mathrm{can}\:\mathrm{use}\:{x}_{\mathrm{1}} \:\mathrm{and}\:{x}_{\mathrm{2}} \:\mathrm{to}\:\mathrm{find}\:{y}_{\mathrm{1}} \:\mathrm{and}\: \\ $$$${y}_{\mathrm{2}} \:\mathrm{in}\:\left(\alpha\right). \\ $$$$\mathrm{You}\:\mathrm{can}\:\mathrm{use}\:\mathrm{too}\:\mathrm{to}\:\mathrm{find}\:{z}_{\mathrm{1}} \:\mathrm{and}\:{z}_{\mathrm{2}} \:\mathrm{in}\:\left(\beta\right) \\ $$$$ \\ $$$$ \\ $$

Commented by tawakalitu last updated on 08/Dec/16

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Answered by mrW last updated on 08/Dec/16

see answer from sou1618 for Q9312. A=x+1 B=y+3 C=z−1 AB=8 ...(1) BC=3 ...(2) CA=2 ...(3) (1)×(2)×(3): (ABC)^2 =8×3×2=48 ABC=±(√(48))=±4(√(3 )) ...(4) (4)÷(1): C=±((4(√3))/8)=z−1 z=1±((√3)/2) (4)÷(2): A=±((4(√3))/3)=x+1 x=−1±((4(√3))/3) (4)÷(3): B=±((4(√3))/2)=y+3 y=−3±2(√3)

$$\mathrm{see}\:\mathrm{answer}\:\mathrm{from}\:\mathrm{sou1618}\:\mathrm{for}\:\mathrm{Q9312}. \\ $$$$\mathrm{A}=\mathrm{x}+\mathrm{1} \\ $$$$\mathrm{B}=\mathrm{y}+\mathrm{3} \\ $$$$\mathrm{C}=\mathrm{z}−\mathrm{1} \\ $$$$\mathrm{AB}=\mathrm{8}\:\:\:\:...\left(\mathrm{1}\right) \\ $$$$\mathrm{BC}=\mathrm{3}\:\:\:\:...\left(\mathrm{2}\right) \\ $$$$\mathrm{CA}=\mathrm{2}\:\:\:\:...\left(\mathrm{3}\right) \\ $$$$\left(\mathrm{1}\right)×\left(\mathrm{2}\right)×\left(\mathrm{3}\right): \\ $$$$\left(\mathrm{ABC}\right)^{\mathrm{2}} =\mathrm{8}×\mathrm{3}×\mathrm{2}=\mathrm{48} \\ $$$$\mathrm{ABC}=\pm\sqrt{\mathrm{48}}=\pm\mathrm{4}\sqrt{\mathrm{3}\:}\:\:\:\:...\left(\mathrm{4}\right) \\ $$$$\left(\mathrm{4}\right)\boldsymbol{\div}\left(\mathrm{1}\right): \\ $$$$\mathrm{C}=\pm\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{8}}=\mathrm{z}−\mathrm{1} \\ $$$$\mathrm{z}=\mathrm{1}\pm\frac{\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\left(\mathrm{4}\right)\boldsymbol{\div}\left(\mathrm{2}\right): \\ $$$$\mathrm{A}=\pm\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}=\mathrm{x}+\mathrm{1} \\ $$$$\mathrm{x}=−\mathrm{1}\pm\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}} \\ $$$$\left(\mathrm{4}\right)\boldsymbol{\div}\left(\mathrm{3}\right): \\ $$$$\mathrm{B}=\pm\frac{\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{2}}=\mathrm{y}+\mathrm{3} \\ $$$$\mathrm{y}=−\mathrm{3}\pm\mathrm{2}\sqrt{\mathrm{3}} \\ $$

Commented by tawakalitu last updated on 08/Dec/16

I really appreciate .. God bless you sir.

$$\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:..\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Answered by RasheedSoomro last updated on 09/Dec/16

(x + 1)(y + 3) = 8 ....... (i) (y + 3)(z − 1) = 3 ......... (ii) (z − 1)(x + 1) = 2 ......... (iii) (i)/(ii)⇒((x+1)/(z−1))=(8/3)⇒3x−8z=−8−3=−11...(iv) (ii)/(iii)⇒((y+3)/(x+1))=(3/2)⇒3x−2y=−3+6=3......(v) (iii)/(i)⇒((z−1)/(y+3))=(2/8)⇒y−4z=−4−3=−7......(vi) (iv)−(v)⇒2y−8z=−14⇒y−4z=−7 (iv)−2(vi)⇒3x−2y=3 (v)+2(vi)⇒3x−8z=−11 So any one of the three equations[(iv),(v) & (vi)] can be derived from remaining two. This means that only two equations are helpful in solution. But for three variables three equations must be given.

$$\left(\mathrm{x}\:+\:\mathrm{1}\right)\left(\mathrm{y}\:+\:\mathrm{3}\right)\:=\:\mathrm{8}\:\:\:.......\:\left(\mathrm{i}\right) \\ $$$$\left(\mathrm{y}\:+\:\mathrm{3}\right)\left(\mathrm{z}\:−\:\mathrm{1}\right)\:=\:\mathrm{3}\:\:.........\:\left(\mathrm{ii}\right) \\ $$$$\left(\mathrm{z}\:−\:\mathrm{1}\right)\left(\mathrm{x}\:+\:\mathrm{1}\right)\:=\:\mathrm{2}\:\:\:.........\:\left(\mathrm{iii}\right) \\ $$$$\left(\mathrm{i}\right)/\left(\mathrm{ii}\right)\Rightarrow\frac{\mathrm{x}+\mathrm{1}}{\mathrm{z}−\mathrm{1}}=\frac{\mathrm{8}}{\mathrm{3}}\Rightarrow\mathrm{3x}−\mathrm{8z}=−\mathrm{8}−\mathrm{3}=−\mathrm{11}...\left(\mathrm{iv}\right) \\ $$$$\left(\mathrm{ii}\right)/\left(\mathrm{iii}\right)\Rightarrow\frac{\mathrm{y}+\mathrm{3}}{\mathrm{x}+\mathrm{1}}=\frac{\mathrm{3}}{\mathrm{2}}\Rightarrow\mathrm{3x}−\mathrm{2y}=−\mathrm{3}+\mathrm{6}=\mathrm{3}......\left(\mathrm{v}\right) \\ $$$$\left(\mathrm{iii}\right)/\left(\mathrm{i}\right)\Rightarrow\frac{\mathrm{z}−\mathrm{1}}{\mathrm{y}+\mathrm{3}}=\frac{\mathrm{2}}{\mathrm{8}}\Rightarrow\mathrm{y}−\mathrm{4z}=−\mathrm{4}−\mathrm{3}=−\mathrm{7}......\left(\mathrm{vi}\right) \\ $$$$\left(\mathrm{iv}\right)−\left(\mathrm{v}\right)\Rightarrow\mathrm{2y}−\mathrm{8z}=−\mathrm{14}\Rightarrow\mathrm{y}−\mathrm{4z}=−\mathrm{7} \\ $$$$\left(\mathrm{iv}\right)−\mathrm{2}\left(\mathrm{vi}\right)\Rightarrow\mathrm{3x}−\mathrm{2y}=\mathrm{3} \\ $$$$\left(\mathrm{v}\right)+\mathrm{2}\left(\mathrm{vi}\right)\Rightarrow\mathrm{3x}−\mathrm{8z}=−\mathrm{11} \\ $$$$\mathrm{So}\:\mathrm{any}\:\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{three}\:\mathrm{equations}\left[\left(\mathrm{iv}\right),\left(\mathrm{v}\right)\:\&\:\left(\mathrm{vi}\right)\right]\:\mathrm{can}\:\mathrm{be}\:\mathrm{derived}\: \\ $$$$\mathrm{from}\:\mathrm{remaining}\:\mathrm{two}. \\ $$$$\mathrm{This}\:\mathrm{means}\:\mathrm{that}\:\mathrm{only}\:\mathrm{two}\:\mathrm{equations}\:\mathrm{are} \\ $$$$\mathrm{helpful}\:\mathrm{in}\:\mathrm{solution}. \\ $$$$\mathrm{But}\:\mathrm{for}\:\mathrm{three}\:\mathrm{variables}\:\mathrm{three}\:\mathrm{equations} \\ $$$$\mathrm{must}\:\mathrm{be}\:\mathrm{given}. \\ $$

Commented by tawakalitu last updated on 08/Dec/16

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com