Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 9533 by FilupSmith last updated on 14/Dec/16

Determine and prove if true: ∫_0 ^( n) x^2 dx < Σ_(k=1) ^n k^2

$$\mathrm{Determine}\:\mathrm{and}\:\mathrm{prove}\:\mathrm{if}\:\mathrm{true}: \\ $$ $$\int_{\mathrm{0}} ^{\:{n}} {x}^{\mathrm{2}} {dx}\:<\:\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \\ $$

Answered by geovane10math last updated on 14/Dec/16

(n^3 /3) + C < 1 + 4 + 9 + ... + n^2 (n^3 /3) + C < ((n(n + 1)(2n + 1))/6) C < (((n^2 + n)(2n + 1))/6) − (n^3 /3) C < ((2n^3 + n^2 + 2n^2 + n − 2n^3 )/6) C < ((3n^2 + n)/6) It depends of the integration′s constant.. 0 < ((3n^2 + n)/6) 3n^2 + n > 0 n(3n + 1) = 0 n_1 = 0 n_2 = − (1/3) S = {n ∈ R ∣ n < − (1/3) or n > 0}

$$\frac{{n}^{\mathrm{3}} }{\mathrm{3}}\:+\:{C}\:\:<\:\mathrm{1}\:+\:\mathrm{4}\:+\:\mathrm{9}\:+\:...\:+\:{n}^{\mathrm{2}} \\ $$ $$\frac{{n}^{\mathrm{3}} }{\mathrm{3}}\:+\:{C}\:<\:\frac{{n}\left({n}\:+\:\mathrm{1}\right)\left(\mathrm{2}{n}\:+\:\mathrm{1}\right)}{\mathrm{6}} \\ $$ $${C}\:<\:\frac{\left({n}^{\mathrm{2}} \:+\:{n}\right)\left(\mathrm{2}{n}\:+\:\mathrm{1}\right)}{\mathrm{6}}\:−\:\frac{{n}^{\mathrm{3}} }{\mathrm{3}}\: \\ $$ $${C}\:<\:\frac{\mathrm{2}{n}^{\mathrm{3}} \:+\:{n}^{\mathrm{2}} \:+\:\mathrm{2}{n}^{\mathrm{2}} \:+\:{n}\:−\:\mathrm{2}{n}^{\mathrm{3}} \:}{\mathrm{6}} \\ $$ $${C}\:<\:\frac{\mathrm{3}{n}^{\mathrm{2}} \:+\:{n}}{\mathrm{6}} \\ $$ $$\mathrm{It}\:\mathrm{depends}\:\mathrm{of}\:\mathrm{the}\:\mathrm{integration}'\mathrm{s}\:\mathrm{constant}.. \\ $$ $$ \\ $$ $$ \\ $$ $$\mathrm{0}\:<\:\frac{\mathrm{3}{n}^{\mathrm{2}} \:+\:{n}}{\mathrm{6}} \\ $$ $$\mathrm{3}{n}^{\mathrm{2}} \:+\:{n}\:>\:\mathrm{0} \\ $$ $${n}\left(\mathrm{3}{n}\:+\:\mathrm{1}\right)\:=\:\mathrm{0} \\ $$ $${n}_{\mathrm{1}} \:=\:\mathrm{0}\:\:\:\:\:\:\:{n}_{\mathrm{2}} \:=\:−\:\frac{\mathrm{1}}{\mathrm{3}} \\ $$ $${S}\:=\:\left\{{n}\:\in\:\mathbb{R}\:\mid\:{n}\:<\:−\:\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{or}\:{n}\:>\:\mathrm{0}\right\} \\ $$ $$ \\ $$ $$ \\ $$

Commented bygeovane10math last updated on 14/Dec/16

DO NOT CONSIDER THE C, I WRONG SORRY

$${DO}\:{NOT}\:{CONSIDER}\:{THE}\:\boldsymbol{{C}}, \\ $$ $${I}\:{WRONG}\:{SORRY} \\ $$

Answered by sou1618 last updated on 14/Dec/16

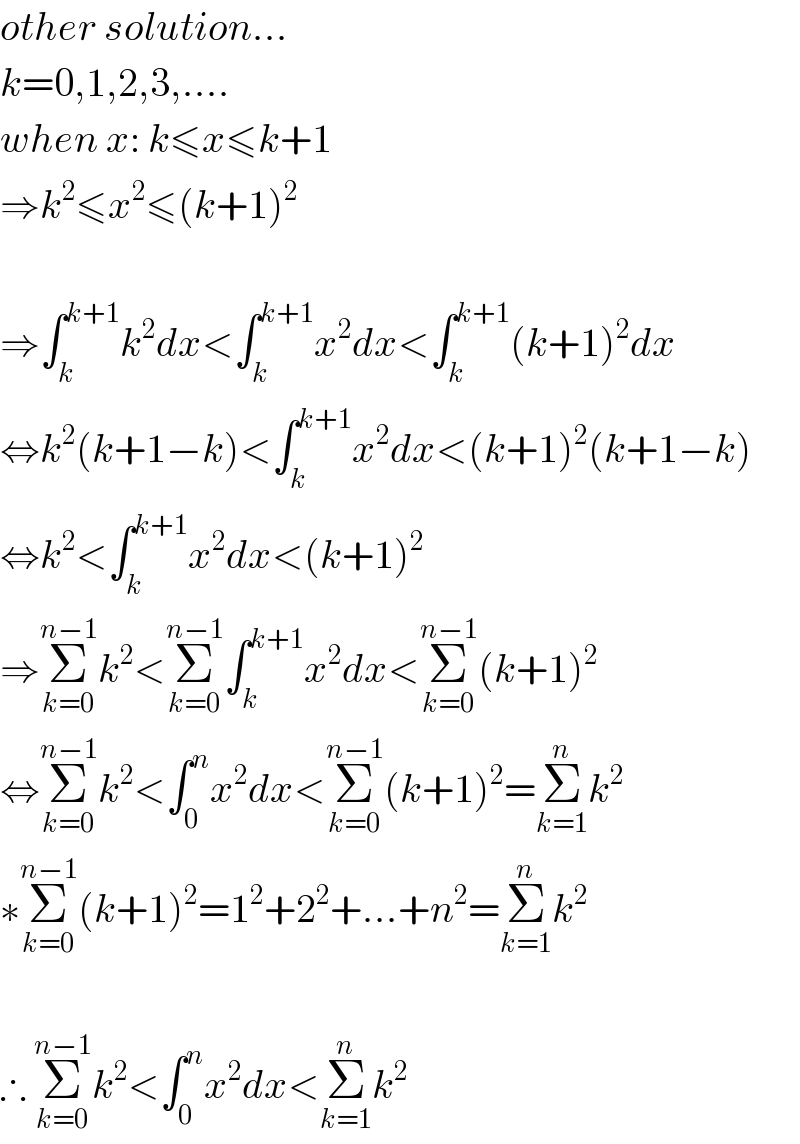
other solution... k=0,1,2,3,.... when x: k≤x≤k+1 ⇒k^2 ≤x^2 ≤(k+1)^2 ⇒∫_k ^(k+1) k^2 dx<∫_k ^(k+1) x^2 dx<∫_k ^(k+1) (k+1)^2 dx ⇔k^2 (k+1−k)<∫_k ^(k+1) x^2 dx<(k+1)^2 (k+1−k) ⇔k^2 <∫_k ^(k+1) x^2 dx<(k+1)^2 ⇒Σ_(k=0) ^(n−1) k^2 <Σ_(k=0) ^(n−1) ∫_k ^(k+1) x^2 dx<Σ_(k=0) ^(n−1) (k+1)^2 ⇔Σ_(k=0) ^(n−1) k^2 <∫_0 ^n x^2 dx<Σ_(k=0) ^(n−1) (k+1)^2 =Σ_(k=1) ^n k^2 ∗Σ_(k=0) ^(n−1) (k+1)^2 =1^2 +2^2 +...+n^2 =Σ_(k=1) ^n k^2 ∴ Σ_(k=0) ^(n−1) k^2 <∫_0 ^n x^2 dx<Σ_(k=1) ^n k^2

$${other}\:{solution}... \\ $$ $${k}=\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3},.... \\ $$ $${when}\:{x}:\:{k}\leqslant{x}\leqslant{k}+\mathrm{1} \\ $$ $$\Rightarrow{k}^{\mathrm{2}} \leqslant{x}^{\mathrm{2}} \leqslant\left({k}+\mathrm{1}\right)^{\mathrm{2}} \\ $$ $$ \\ $$ $$\Rightarrow\int_{{k}} ^{{k}+\mathrm{1}} {k}^{\mathrm{2}} {dx}<\int_{{k}} ^{{k}+\mathrm{1}} {x}^{\mathrm{2}} {dx}<\int_{{k}} ^{{k}+\mathrm{1}} \left({k}+\mathrm{1}\right)^{\mathrm{2}} {dx} \\ $$ $$\Leftrightarrow{k}^{\mathrm{2}} \left({k}+\mathrm{1}−{k}\right)<\int_{{k}} ^{{k}+\mathrm{1}} {x}^{\mathrm{2}} {dx}<\left({k}+\mathrm{1}\right)^{\mathrm{2}} \left({k}+\mathrm{1}−{k}\right) \\ $$ $$\Leftrightarrow{k}^{\mathrm{2}} <\int_{{k}} ^{{k}+\mathrm{1}} {x}^{\mathrm{2}} {dx}<\left({k}+\mathrm{1}\right)^{\mathrm{2}} \\ $$ $$\Rightarrow\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}{k}^{\mathrm{2}} <\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\int_{{k}} ^{{k}+\mathrm{1}} {x}^{\mathrm{2}} {dx}<\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\left({k}+\mathrm{1}\right)^{\mathrm{2}} \\ $$ $$\Leftrightarrow\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}{k}^{\mathrm{2}} <\int_{\mathrm{0}} ^{{n}} {x}^{\mathrm{2}} {dx}<\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\left({k}+\mathrm{1}\right)^{\mathrm{2}} =\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \\ $$ $$\ast\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}\left({k}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{1}^{\mathrm{2}} +\mathrm{2}^{\mathrm{2}} +...+{n}^{\mathrm{2}} =\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \\ $$ $$ \\ $$ $$\therefore\:\underset{{k}=\mathrm{0}} {\overset{{n}−\mathrm{1}} {\sum}}{k}^{\mathrm{2}} <\int_{\mathrm{0}} ^{{n}} {x}^{\mathrm{2}} {dx}<\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \\ $$

Answered by mrW last updated on 14/Dec/16

∫_0 ^( n) x^2 dx=(1/3)[x^3 ]_0 ^n =(n^3 /3) Σ_(k=1) ^n k^2 =((n(n+1)(2n+1))/6) =((2n^3 +3n^2 +n)/6)=(n^3 /3)+((n^2 /2)+(n/6)) >(n^3 /3)=∫_0 ^( n) x^2 dx

$$\int_{\mathrm{0}} ^{\:{n}} {x}^{\mathrm{2}} {dx}=\frac{\mathrm{1}}{\mathrm{3}}\left[\mathrm{x}^{\mathrm{3}} \right]_{\mathrm{0}} ^{\mathrm{n}} =\frac{\mathrm{n}^{\mathrm{3}} }{\mathrm{3}} \\ $$ $$\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} =\frac{\mathrm{n}\left(\mathrm{n}+\mathrm{1}\right)\left(\mathrm{2n}+\mathrm{1}\right)}{\mathrm{6}} \\ $$ $$=\frac{\mathrm{2n}^{\mathrm{3}} +\mathrm{3n}^{\mathrm{2}} +\mathrm{n}}{\mathrm{6}}=\frac{\mathrm{n}^{\mathrm{3}} }{\mathrm{3}}+\left(\frac{\mathrm{n}^{\mathrm{2}} }{\mathrm{2}}+\frac{\mathrm{n}}{\mathrm{6}}\right) \\ $$ $$>\frac{\mathrm{n}^{\mathrm{3}} }{\mathrm{3}}=\int_{\mathrm{0}} ^{\:{n}} {x}^{\mathrm{2}} {dx} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com