calculate ∫_1 ^(+∞) (dx/(x^3 (2x+1)^4 )) 1)without use of decomposition 2)by use of decomposition


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 95584 by turbo msup by abdo last updated on 26/May/20

$c a l c u l a t e \int_{1}^{+ \infty} \frac{d x}{x^{3} {(2 x + 1)}^{4}}$ $1) w i t h o u t u s e o f d e c o m p o s i t i o n$ $2) b y u s e o f d e c o m p o s i t i o n$
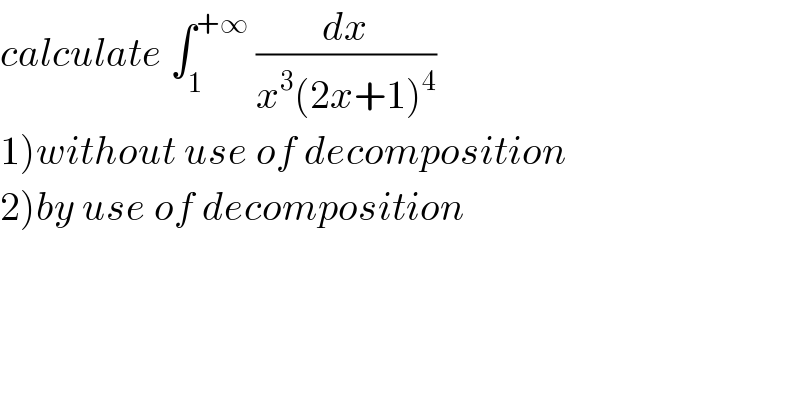
	Answered by MJS last updated on 26/May/20

	$Ostrogradski leads to$ $\frac{960 x^{4} + 1200 x^{3} + 440 x^{2} + 30 x - 3}{6 x^{2} {(2 x + 1)}^{3}} + 40 \int \frac{d x}{x (2 x + 1)} =$ $= \frac{960 x^{4} + 1200 x^{3} + 440 x^{2} + 30 x - 3}{6 x^{2} {(2 x + 1)}^{3}} + 40 \ln \frac{x}{2 x + 1} + C$ $\Rightarrow$ $\underset{1}{\int^{+ \infty}} \frac{d x}{x^{3} {(2 x + 1)}^{4}} = 40 \ln \frac{3}{2} - \frac{2627}{162}$
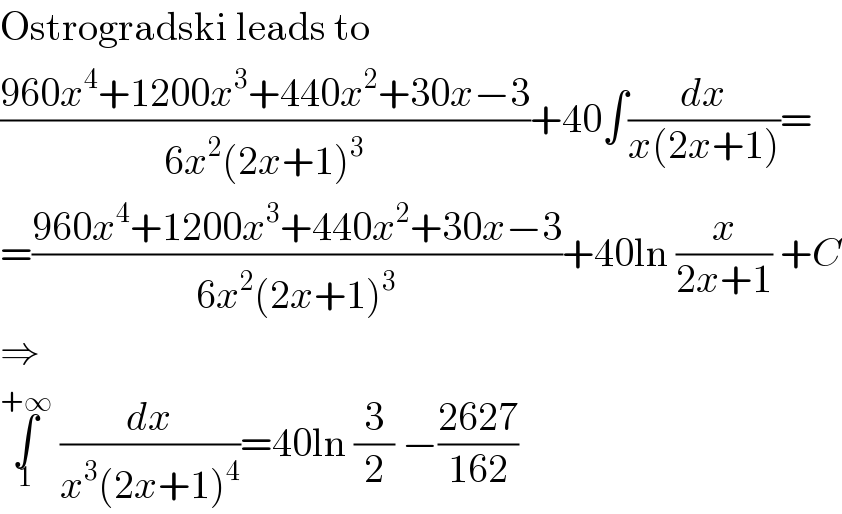
	Commented by mathmax by abdo last updated on 26/May/20

	$thank you sir mjs$
	Answered by mathmax by abdo last updated on 26/May/20
	$A =∫_1 ^(+∞) (dx/(x^3 (2x+1)^4 ))⇒A =∫_1 ^(+∞) (dx/(((x/(2x+1)))^3 (2x+1)^7 )) we do the changement (x/(2x+1)) =t ⇒ x =2tx +t ⇒(1−2t)x =t ⇒x =(t/(1−2t)) ⇒(dx/dt) =((1−2t −t(−2t))/((1−2t)^2 )) =(1/((1−2t)^2 )) and 2x+1 =((2t)/(1−2t)) +1 =(1/(1−2t)) ⇒ A = ∫_(1/3) ^(1/2) (dt/((2t−1)^2 t^3 ((1/(1−2t)))^7 )) =−∫_(1/3) ^(1/2) (((2t−1)^7 )/((2t−1)^2 t^3 ))dt =−∫_(1/3) ^(1/2) (((2t−1)^5 )/t^3 ) dt =−∫_(1/3) ^(1/2) ((Σ_(k=0) ^5 C_5 ^k (2t)^k (−1)^(5−k) )/t^3 )dt =∫_(1/3) ^(1/2) ((Σ_(k=0) ^5 2^k (−1)^k C_5 ^k t^k )/t^3 )dt =Σ_(k=0) ^5 C_5 ^k (−2)^k ∫_(1/3) ^(1/2) t^(k−3) dt =Σ_(k=0 and k≠2) ^5 (−2)^k C_5 ^k [(1/(k−2))t^(k−2) ]_(1/3) ^(1/2) +4 C_5 ^2 [lnt]_(1/3) ^(1/2) =Σ_(k=0 and k≠2) ^5 (−2)^k (C_5 ^k /(k−2)){ ((1/2))^(k−2) −((1/3))^(k−2) }+4C_5 ^2 {ln((1/2))−ln((1/3))}$
	$A = \int_{1}^{+ \infty} \frac{dx}{x^{3} {(2 x + 1)}^{4}} \Rightarrow A = \int_{1}^{+ \infty} \frac{dx}{{(\frac{x}{2 x + 1})}^{3} {(2 x + 1)}^{7}} we do the changement \frac{x}{2 x + 1} = t \Rightarrow$ $x = 2 tx + t \Rightarrow (1 - 2 t) x = t \Rightarrow x = \frac{t}{1 - 2 t} \Rightarrow \frac{dx}{dt} = \frac{1 - 2 t - t (- 2 t)}{{(1 - 2 t)}^{2}}$ $= \frac{1}{{(1 - 2 t)}^{2}} and 2 x + 1 = \frac{2 t}{1 - 2 t} + 1 = \frac{1}{1 - 2 t} \Rightarrow$ $A = \int_{\frac{1}{3}}^{\frac{1}{2}} \frac{dt}{{(2 t - 1)}^{2} t^{3} {(\frac{1}{1 - 2 t})}^{7}} = - \int_{\frac{1}{3}}^{\frac{1}{2}} \frac{{(2 t - 1)}^{7}}{{(2 t - 1)}^{2} t^{3}} dt$ $= - \int_{\frac{1}{3}}^{\frac{1}{2}} \frac{{(2 t - 1)}^{5}}{t^{3}} dt = - \int_{\frac{1}{3}}^{\frac{1}{2}} \frac{\sum_{k = 0}^{5} C_{5}^{k} {(2 t)}^{k} {(- 1)}^{5 - k}}{t^{3}} dt$ $= \int_{\frac{1}{3}}^{\frac{1}{2}} \frac{\sum_{k = 0}^{5} 2^{k} {(- 1)}^{k} C_{5}^{k} t^{k}}{t^{3}} dt$ $= \sum_{k = 0}^{5} C_{5}^{k} {(- 2)}^{k} \int_{\frac{1}{3}}^{\frac{1}{2}} t^{k - 3} dt$ $= \sum_{k = 0 and k \neq 2}^{5} {(- 2)}^{k} C_{5}^{k} {[\frac{1}{k - 2} t^{k - 2}]}_{\frac{1}{3}}^{\frac{1}{2}} + 4 C_{5}^{2} {[lnt]}_{\frac{1}{3}}^{\frac{1}{2}}$ $= \sum_{k = 0 and k \neq 2}^{5} {(- 2)}^{k} \frac{C_{5}^{k}}{k - 2} {{(\frac{1}{2})}^{k - 2} - {(\frac{1}{3})}^{k - 2}} + {4 C}_{5}^{2} {\ln (\frac{1}{2}) - \ln (\frac{1}{3})}$
	Answered by mathmax by abdo last updated on 27/May/20

	$thank you sir mjs$ $2) let decompose F (x) = \frac{1}{x^{3} {(2 x + 1)}^{4}} \Rightarrow$ $2^{4} F (x) = \frac{1}{x^{3} {(x + \frac{1}{2})}^{4}} = \sum_{i = 1}^{3} \frac{a_{i}}{x^{i}} + \sum_{i = 1}^{4} \frac{b_{i}}{{(x + \frac{1}{2})}^{i}}$ $a_{i} ? we find D_{2} (0) for f (x) = {(x + \frac{1}{2})}^{- 4}$ $f (x) = f (0) + \frac{x}{1!} f^{^{'}} (0) + \frac{x^{2}}{2!} f^{(2)} (0) + \frac{x^{3}}{3!} ξ (x)$ $f (0) = \frac{1}{2^{- 4}}, f^{^{'}} (x) = - 4 {(x + \frac{1}{2})}^{- 5} \Rightarrow f^{^{'}} (0) = \frac{- 4}{2^{- 5}}$ $f^{(2)} (x) = 20 {(x + \frac{1}{2})}^{- 6} \Rightarrow f^{(2)} (0) = \frac{20}{2^{- 6}} \Rightarrow$ $f (x) = 2^{4} - 4 . 2^{5} x + 10 . 2^{6} x^{2} + \frac{x^{3}}{3!} ξ (x) \Rightarrow$ $\frac{f (x)}{x^{3}} = \frac{2^{4}}{x^{3}} - \frac{4 . 2^{5}}{x^{2}} + \frac{10 . 2^{6}}{x} + \frac{1}{3!} ξ (x) \Rightarrow a_{1} = 10 . 2^{6}$ $a_{2} = - 4 . 2^{5} and a_{3} = 2^{4} let find b_{i}$ $we determine D_{3} (- \frac{1}{2}) for g (x) = x^{- 3}$ $g (x) = g (- \frac{1}{2}) + \frac{x + \frac{1}{2}}{1!} g^{^{'}} (- \frac{1}{2}) + \frac{{(x + \frac{1}{2})}^{2}}{2!} g^{(2)} (- \frac{1}{2})$ $+ \frac{{(x + \frac{1}{2})}^{3}}{3!} g^{(3)} (- \frac{1}{2}) + \frac{{(x + \frac{1}{2})}^{4}}{4!} ξ (x)$ $g (- \frac{1}{2}) = {(- \frac{1}{2})}^{- 3}, g^{^{'}} (x) = - {3 x}^{- 4} \Rightarrow g^{^{'}} (- \frac{1}{2}) = - 3 {(- \frac{1}{2})}^{- 4}$ $g^{(2)} (x) = 12 x^{- 5} \Rightarrow g^{(2)} (- \frac{1}{2}) = 12 {(- \frac{1}{2})}^{- 5}$ $g^{(3)} (x) = - 60 x^{- 6} \Rightarrow g^{(3)} (- \frac{1}{2}) = - 60 {(- \frac{1}{2})}^{- 6}$ $\Rightarrow g (x) = {(- \frac{1}{2})}^{- 3} - 3 {(- \frac{1}{2})}^{- 4} (x + \frac{1}{2}) + 6 {(- \frac{1}{2})}^{- 5} {(x + \frac{1}{2})}^{2}$ $- 10 {(- \frac{1}{2})}^{- 6} {(x + \frac{1}{2})}^{3} + \frac{{(x + \frac{1}{2})}^{4}}{4!} ξ (x)$ $= - 2^{3} - 3 . 2^{4} (x + \frac{1}{2}) - 6 . 2^{5} {(x + \frac{1}{2})}^{2} - 10 . 2^{6} {(x + \frac{1}{2})}^{3}$ $+ \frac{{(x + \frac{1}{2})}^{4}}{4!} ξ (x) \Rightarrow$ $\frac{g (x)}{{(x + \frac{1}{2})}^{4}} = - \frac{2^{3}}{{(x + \frac{1}{2})}^{4}} - \frac{3 . 2^{4}}{{(x + \frac{1}{2})}^{3}} - \frac{6 . 2^{5}}{{(x + \frac{1}{2})}^{2}} - \frac{10 . 2^{6}}{(x + \frac{1}{2})} + . . \Rightarrow$ $\Rightarrow b_{1} = - 10 . 2^{6}, b_{2} = - 6 . 2^{5}, b_{3} = - 3 . 2^{4}, b_{4} = - 2^{3} \Rightarrow$ $2^{4} F (x) = \frac{10 . 2^{6}}{x} - \frac{4 . 2^{5}}{x^{2}} + \frac{2^{4}}{x^{3}} - \frac{10 . 2^{6}}{(x + \frac{1}{2})} - \frac{6 . 2^{5}}{{(x + \frac{1}{2})}^{2}} - \frac{3 . 2^{4}}{{(x + \frac{1}{2})}^{3}}$ $- \frac{2^{3}}{{(x + \frac{1}{2})}^{4}} \Rightarrow$ $F (x) = \frac{10 . 2^{2}}{x} - \frac{4.2}{x^{2}} + \frac{1}{x^{3}} - \frac{10 . 2^{2}}{(x + \frac{1}{2})} - \frac{6.2}{{(x + \frac{1}{2})}^{2}} - \frac{3}{{(x + \frac{1}{2})}^{3}}$ $- \frac{2^{- 1}}{{(x + \frac{1}{2})}^{4}} now its eazy to find \int_{1}^{+ \infty} F (x) dx . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum