
Question Number 95668 by I want to learn more last updated on 26/May/20
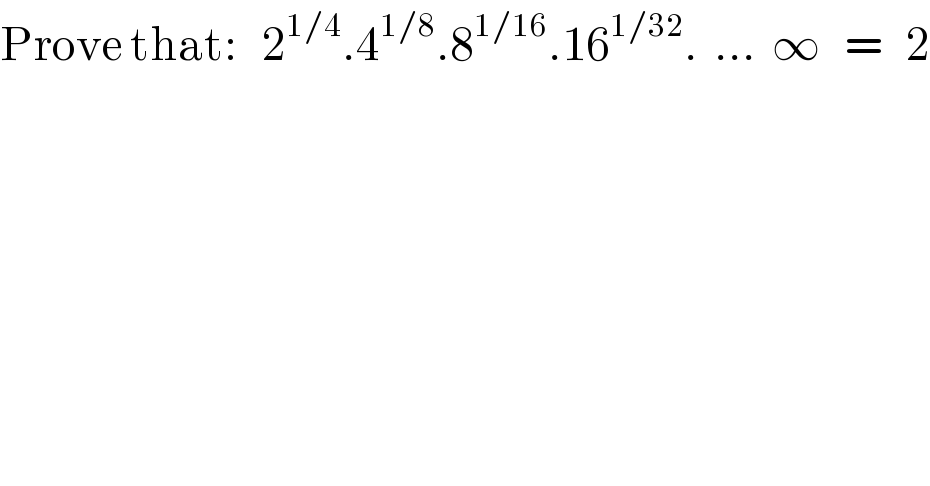
$$\mathrm{Prove}\:\mathrm{that}:\:\:\:\mathrm{2}^{\mathrm{1}/\mathrm{4}} .\mathrm{4}^{\mathrm{1}/\mathrm{8}} .\mathrm{8}^{\mathrm{1}/\mathrm{16}} .\mathrm{16}^{\mathrm{1}/\mathrm{32}} .\:\:...\:\:\infty\:\:\:=\:\:\:\mathrm{2} \\ $$
Commented by Tony Lin last updated on 26/May/20
![2^(1/4) ×4^(1/8) ×8^(1/(16)) ×16^(1/(32)) ×∙∙∙ =2^(1/4) ×(2^2 )^(1/8) ×(2^3 )^(1/(16)) ×(2^4 )^(1/(32)) ×∙∙∙=2^1 so what we need to prove is (1/2^2 )+(2/2^3 )+(3/2^4 )+(4/2^5 )+∙∙∙=1 ⇒Σ_(k=1) ^∞ (k/2^(k+1) )=1 let f(x)=Σ_(k=1) ^∞ k (x^(k−1) /2^(k+1) ) let F(x)=∫_0 ^x f(t)dt=Σ_(k=1) ^∞ (x^k /2^(k+1) )+c =(1/2)[((x/2))^1 +((x/2))^2 +((x/2))^3 +∙∙∙]+c =(1/2)×((x/2)/(1−(x/2)))+c =(x/(4−2x))+c when x=0, F(x)=0 ∴ c=0 ⇒F(x)=(x/(4−2x)) f(x)=F ′(x)=(4/((4−2x)^2 )) plug x=1 ⇒Σ_(k=1) ^∞ (k/2^(k+1) )=(4/((4−2)^2 ))=1 hence proved](Q95675.png)
$$\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{4}}} ×\mathrm{4}^{\frac{\mathrm{1}}{\mathrm{8}}} ×\mathrm{8}^{\frac{\mathrm{1}}{\mathrm{16}}} ×\mathrm{16}^{\frac{\mathrm{1}}{\mathrm{32}}} ×\centerdot\centerdot\centerdot \\ $$$$=\mathrm{2}^{\frac{\mathrm{1}}{\mathrm{4}}} ×\left(\mathrm{2}^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{8}}} ×\left(\mathrm{2}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{16}}} ×\left(\mathrm{2}^{\mathrm{4}} \right)^{\frac{\mathrm{1}}{\mathrm{32}}} ×\centerdot\centerdot\centerdot=\mathrm{2}^{\mathrm{1}} \\ $$$${so}\:{what}\:{we}\:{need}\:{to}\:{prove}\:{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }+\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{3}} }+\frac{\mathrm{3}}{\mathrm{2}^{\mathrm{4}} }+\frac{\mathrm{4}}{\mathrm{2}^{\mathrm{5}} }+\centerdot\centerdot\centerdot=\mathrm{1} \\ $$$$\Rightarrow\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{k}}{\mathrm{2}^{{k}+\mathrm{1}} }=\mathrm{1} \\ $$$${let}\:{f}\left({x}\right)=\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}{k}\:\frac{{x}^{{k}−\mathrm{1}} }{\mathrm{2}^{{k}+\mathrm{1}} } \\ $$$${let}\:{F}\left({x}\right)=\int_{\mathrm{0}} ^{{x}} {f}\left({t}\right){dt}=\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{x}^{{k}} }{\mathrm{2}^{{k}+\mathrm{1}} }+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{1}} +\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} +\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{3}} +\centerdot\centerdot\centerdot\right]+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\frac{{x}}{\mathrm{2}}}{\mathrm{1}−\frac{{x}}{\mathrm{2}}}+{c} \\ $$$$=\frac{{x}}{\mathrm{4}−\mathrm{2}{x}}+{c} \\ $$$${when}\:{x}=\mathrm{0},\:{F}\left({x}\right)=\mathrm{0} \\ $$$$\therefore\:{c}=\mathrm{0} \\ $$$$\Rightarrow{F}\left({x}\right)=\frac{{x}}{\mathrm{4}−\mathrm{2}{x}} \\ $$$${f}\left({x}\right)={F}\:'\left({x}\right)=\frac{\mathrm{4}}{\left(\mathrm{4}−\mathrm{2}{x}\right)^{\mathrm{2}} } \\ $$$${plug}\:{x}=\mathrm{1} \\ $$$$\Rightarrow\underset{{k}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{k}}{\mathrm{2}^{{k}+\mathrm{1}} }=\frac{\mathrm{4}}{\left(\mathrm{4}−\mathrm{2}\right)^{\mathrm{2}} }=\mathrm{1} \\ $$$${hence}\:{proved} \\ $$
Commented by I want to learn more last updated on 06/Jun/20

$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
Answered by Rio Michael last updated on 26/May/20
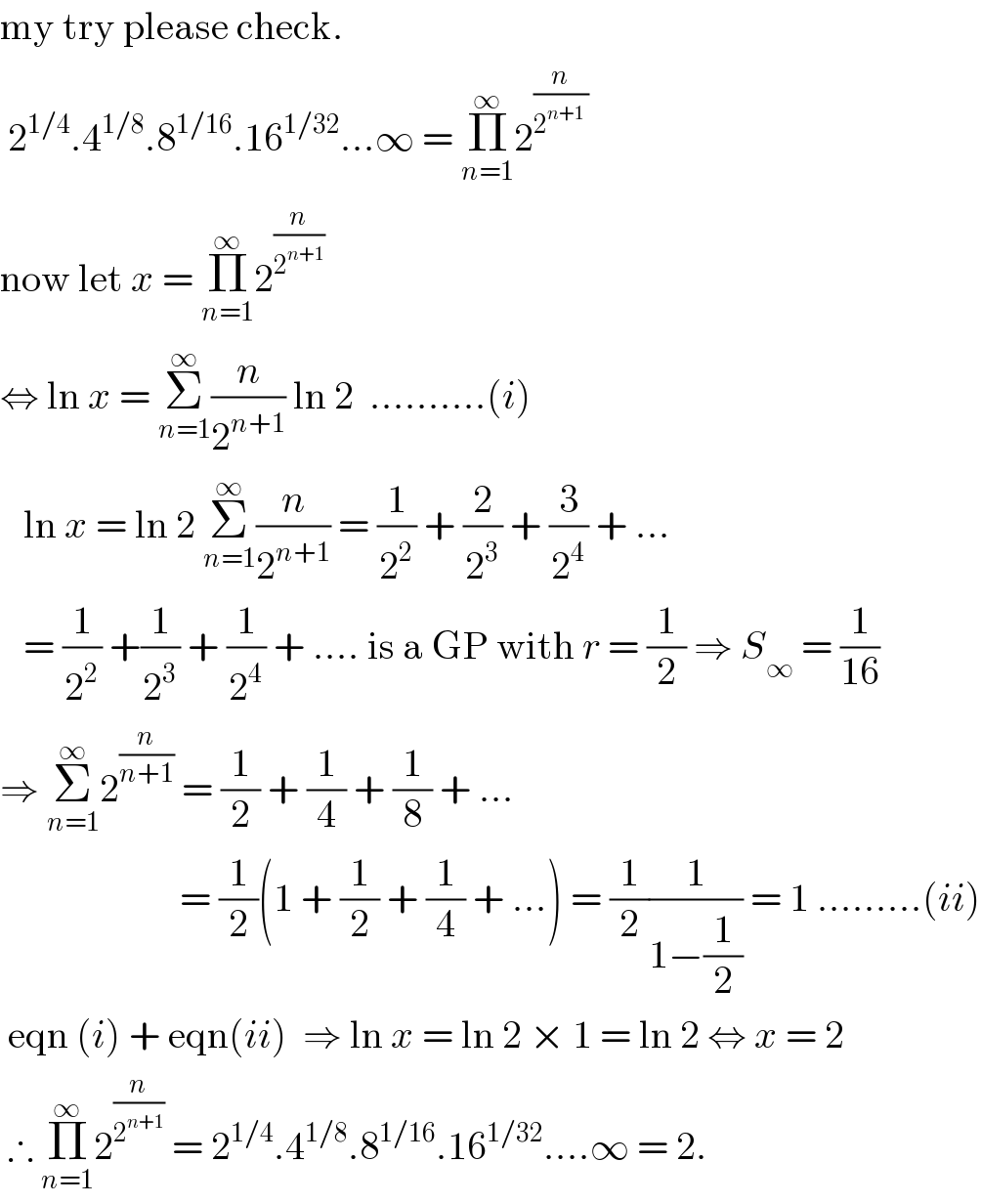
$$\mathrm{my}\:\mathrm{try}\:\mathrm{please}\:\mathrm{check}. \\ $$$$\:\mathrm{2}^{\mathrm{1}/\mathrm{4}} .\mathrm{4}^{\mathrm{1}/\mathrm{8}} .\mathrm{8}^{\mathrm{1}/\mathrm{16}} .\mathrm{16}^{\mathrm{1}/\mathrm{32}} ...\infty\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\mathrm{2}^{\frac{{n}}{\mathrm{2}^{{n}+\mathrm{1}} \:}} \: \\ $$$$\mathrm{now}\:\mathrm{let}\:{x}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\mathrm{2}^{\frac{{n}}{\mathrm{2}^{{n}+\mathrm{1}} }} \: \\ $$$$\Leftrightarrow\:\mathrm{ln}\:{x}\:=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}}{\mathrm{2}^{{n}+\mathrm{1}} }\:\mathrm{ln}\:\mathrm{2}\:\:..........\left({i}\right) \\ $$$$\:\:\:\mathrm{ln}\:{x}\:=\:\mathrm{ln}\:\mathrm{2}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}}{\mathrm{2}^{{n}+\mathrm{1}} }\:=\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\:+\:\frac{\mathrm{2}}{\mathrm{2}^{\mathrm{3}} }\:+\:\frac{\mathrm{3}}{\mathrm{2}^{\mathrm{4}} }\:+\:... \\ $$$$\:\:\:=\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{2}} }\:+\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{3}} }\:+\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{4}} }\:+\:....\:\mathrm{is}\:\mathrm{a}\:\mathrm{GP}\:\mathrm{with}\:{r}\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\:{S}_{\infty} \:=\:\frac{\mathrm{1}}{\mathrm{16}} \\ $$$$\Rightarrow\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\mathrm{2}^{\frac{{n}}{{n}+\mathrm{1}}} \:=\:\frac{\mathrm{1}}{\mathrm{2}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\:+\:\frac{\mathrm{1}}{\mathrm{8}}\:+\:...\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\:+\:...\right)\:=\:\frac{\mathrm{1}}{\mathrm{2}}\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}}}\:=\:\mathrm{1}\:.........\left({ii}\right) \\ $$$$\:\mathrm{eqn}\:\left({i}\right)\:+\:\mathrm{eqn}\left({ii}\right)\:\:\Rightarrow\:\mathrm{ln}\:{x}\:=\:\mathrm{ln}\:\mathrm{2}\:×\:\mathrm{1}\:=\:\mathrm{ln}\:\mathrm{2}\:\Leftrightarrow\:{x}\:=\:\mathrm{2} \\ $$$$\:\therefore\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\prod}}\mathrm{2}^{\frac{{n}}{\mathrm{2}^{{n}+\mathrm{1}} }} \:=\:\mathrm{2}^{\mathrm{1}/\mathrm{4}} .\mathrm{4}^{\mathrm{1}/\mathrm{8}} .\mathrm{8}^{\mathrm{1}/\mathrm{16}} .\mathrm{16}^{\mathrm{1}/\mathrm{32}} ....\infty\:=\:\mathrm{2}. \\ $$
Commented by I want to learn more last updated on 06/Jun/20

$$\mathrm{Thanks}\:\mathrm{sir} \\ $$
