Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

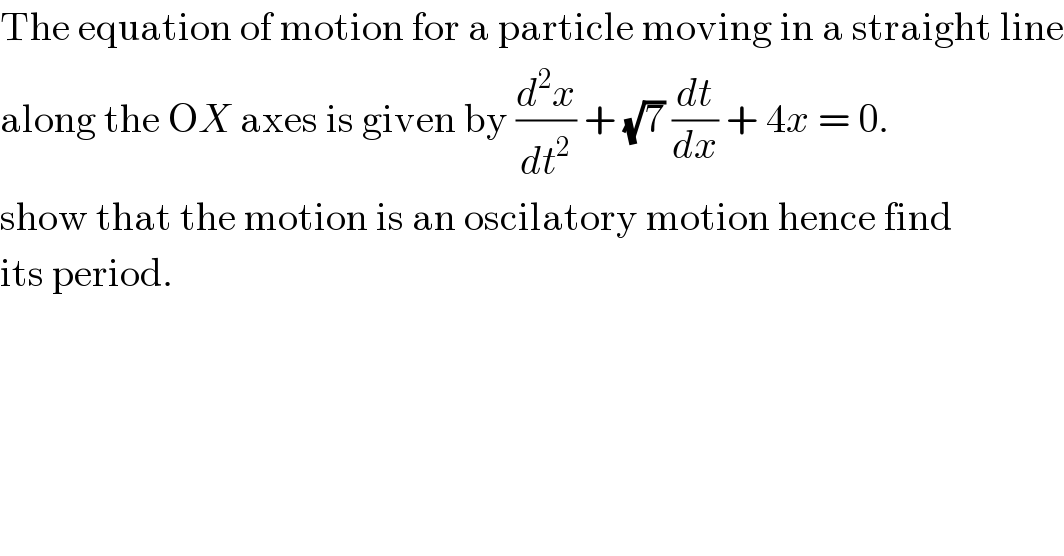
Question Number 95679 by Rio Michael last updated on 26/May/20

$$\mathrm{The}\mathrm{equation}\mathrm{of}\mathrm{motion}\mathrm{for}\mathrm{a}\mathrm{particle}\mathrm{moving}\mathrm{in}\mathrm{a}\mathrm{straight}\mathrm{line}$$$$\mathrm{along}\mathrm{the}\mathrm{O}X\mathrm{axes}\mathrm{is}\mathrm{given}\mathrm{by}\frac{d^{2}x}{{dt}^2}+\sqrt{7}\frac{dt}{dx}+4x=0.$$$$\mathrm{show}\mathrm{that}\mathrm{the}\mathrm{motion}\mathrm{is}\mathrm{an}\mathrm{oscilatory}\mathrm{motion}\mathrm{hence}\mathrm{find}$$$$\mathrm{its}\mathrm{period}.$$

Commented by Tony Lin last updated on 27/May/20

$${\lambda }^2+\sqrt{7}\lambda +4=0$$$$\lambda =\frac{-\sqrt{7}\pm 3i}{2}$$$$x=e^{-\frac{\sqrt{7}}{2}t}(c_{1}cos\frac{3}{2}t+c_{2}sin\frac{3}{2}t)$$$$=e^{-\frac{\sqrt{7}}{2}t}\sqrt{c_1^2+c_2^2}(\frac{c_1}{\sqrt{c_1^2+c_2^2}}cos\frac{3}{2}t+\frac{c_2}{\sqrt{c_1^2+c_2^2}}sin\frac{3}{2}t)$$$$let\frac{c_1}{\sqrt{c_1^2+c_2^2}}=sin\theta$$$$\theta ={sin}^{-1}\frac{c_1}{\sqrt{c_1^2+c_2^2}}$$$$\Rightarrow x=e^{-\frac{\sqrt{7}}{2}t}\sqrt{c_1^2+c_2^2}\left[sin(\frac{3}{2}t+\theta )\right]$$$$\frac{dx}{dt}=-\frac{\sqrt{7}}{2}{e}^{-\frac{\sqrt{7}}{2}t}\sqrt{c_1^2+c_2^2}\frac{3}{2}\left[cos(\frac{3}{2}t+\theta )\right]$$$$\frac{d^{2}x}{{dt}^2}=-\frac{7}{4}{e}^{-\frac{\sqrt{7}}{2}t}\sqrt{c_1^2+c_2^2}\frac{9}{4}\left[sin(\frac{3}{2}t+\theta )\right]$$$$=-\frac{63}{16}x$$$$F=-\frac{63m}{16}x$$$$\to oscillatorymotion$$$$periodT=\frac{2\pi }{\omega }=\frac{2\pi }{\frac{3}{2}}=\frac{4}{3}\pi$$$$butwhent\to \mathrm{\infty },e^{-\frac{\sqrt{7}}{2}t}\to 0$$$$sotheamplitudewoulddecreasegradually$$

Commented by Rio Michael last updated on 27/May/20

$$\mathrm{thank}\mathrm{you}\mathrm{sir}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com