
Question and Answers Forum
Previous in Relation and Functions Next in Relation and Functions
Question Number 95694 by mathmax by abdo last updated on 27/May/20
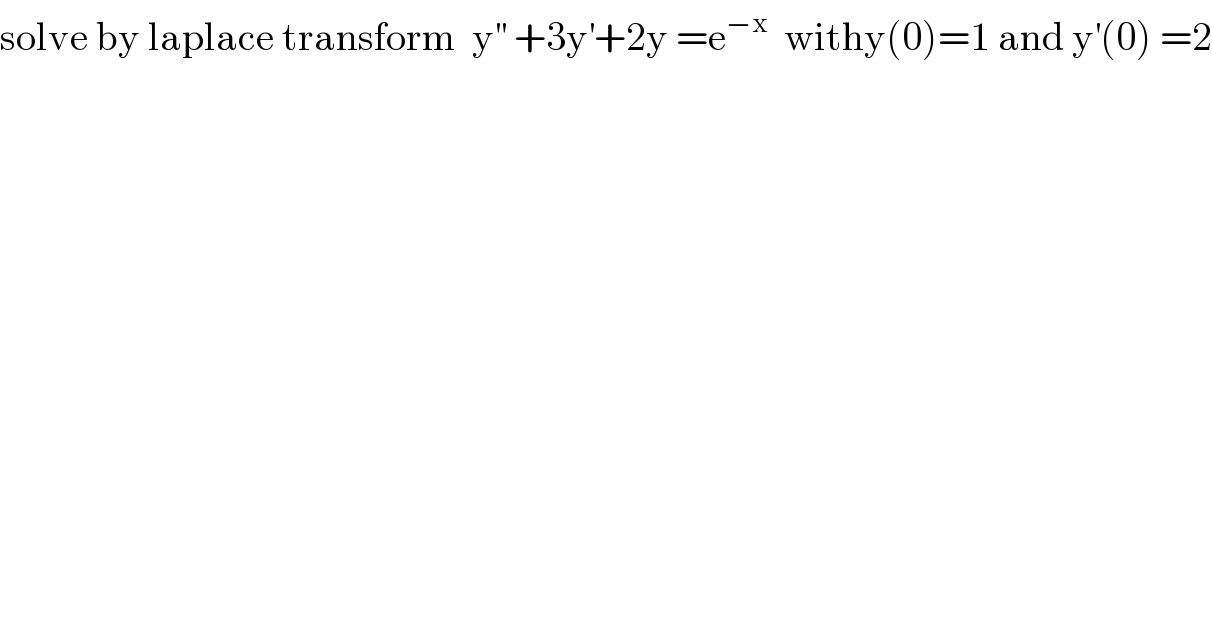
Answered by mathmax by abdo last updated on 27/May/20
![(e)⇒L(y^(′′) )+3L(y^′ )+2L(y) =L(e^(−x) ) ⇒ x^2 L(y)−xy(0)−y^′ (0)+3(xL(y)−y(0))+2L(y) =L(e^(−x) ) ⇒ (x^2 +3x+2)L(y)−x−2−3 =L(e^(−x) ) ⇒(x^2 +3x+2)L(y) =x+5 +L(e^(−x) ) L(e^(−x) ) =∫_0 ^∞ e^(−t) e^(−xt) dt =∫_0 ^∞ e^(−(1+x)t) dt =[−(1/(1+x))e^(−(1+x)t) ]_0 ^∞ =(1/(x+1)) so (e) ⇒(x^2 +3x+2)L(y) =x+5+(1/(x+1)) ⇒ L(y) =((x+5)/(x^2 +3x+2)) +(1/((x+1)(x^2 +3x+2))) ⇒y(x)=L^(−1) (((x+5)/(x^2 +3x+2)))+L^(−1) ((1/((x+1)(x^2 +3x+2)))) x^2 +3x+2 =x^2 −1 +3x+3 =(x−1)(x+1)+3(x+1) =(x+1)(x−1+3) =(x+1)(x+2) ⇒((x+5)/(x^2 +3x+2)) =((x+5)/((x+1)(x+2))) =(a/(x+1)) +(b/(x+2)) a =4 and b =(3/(−1))=−3 ⇒((x+5)/(x^2 +3x+2)) =(4/(x+1))−(3/(x+2)) ⇒ L^(−1) (((x+5)/(x^2 +3x+2))) =4L^(−1) ((1/(x+1)))−3L^(−1) ((1/(x+2))) =4e^(−x) −3e^(−2x) let decompose g(x) =(1/((x+1)(x^2 +3x+2))) ⇒g(x) =(1/((x+1)^2 (x+2))) =(a/(x+1)) +(b/((x+1)^2 )) +(c/(x+2)) b =1 and c =1 ⇒g(x) =(a/(x+1)) +(1/((x+1)^2 )) +(1/(x+2)) lim_(x→+∞) xg(x) =0 =a +1 ⇒a =−1 ⇒g(x) =−(1/(x+1)) +(1/((x+1)^2 )) +(1/(x+2)) ⇒ L^(−1) (g(x)) =−e^(−x) +xe^(−x) +e^(−2x) ⇒ y(x) =4e^(−x) −3e^(−2x) −e^(−x) +xe^(−x) +e^(−2x) =3e^(−x) −2e^(−2x) +xe^(−x) ⇒y(x) =(x+3)e^(−x) −2e^(−2x)](Q95831.png)
| ||
Question and Answers Forum | ||
Previous in Relation and Functions Next in Relation and Functions | ||
Question Number 95694 by mathmax by abdo last updated on 27/May/20 | ||
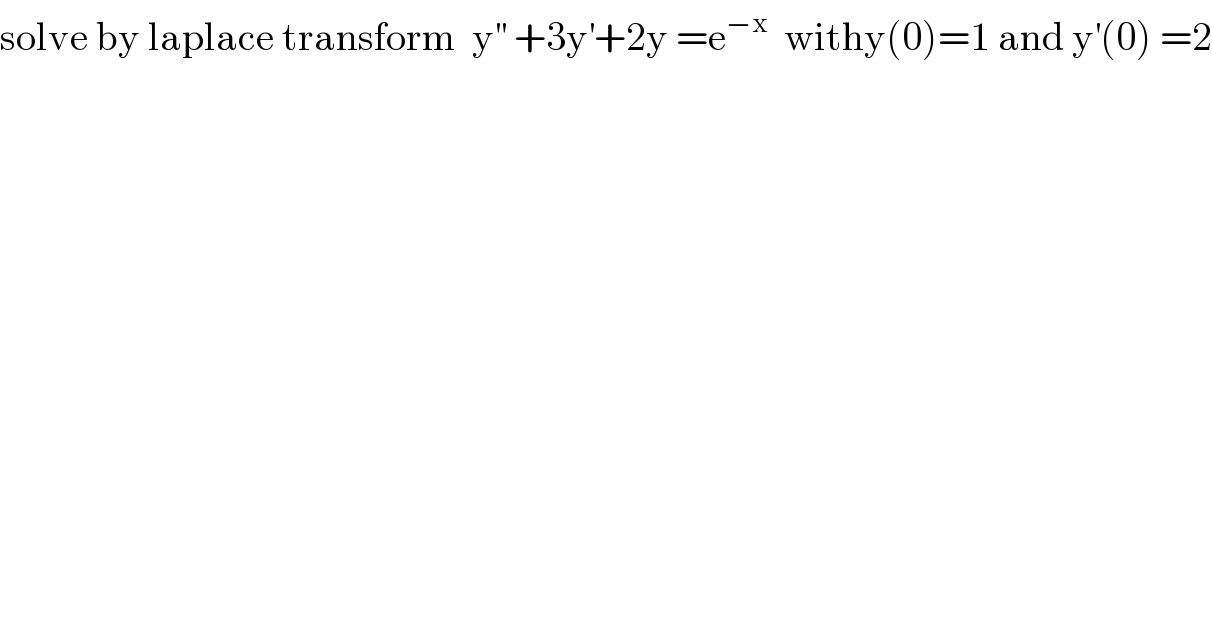 | ||
Answered by mathmax by abdo last updated on 27/May/20 | ||
![(e)⇒L(y^(′′) )+3L(y^′ )+2L(y) =L(e^(−x) ) ⇒ x^2 L(y)−xy(0)−y^′ (0)+3(xL(y)−y(0))+2L(y) =L(e^(−x) ) ⇒ (x^2 +3x+2)L(y)−x−2−3 =L(e^(−x) ) ⇒(x^2 +3x+2)L(y) =x+5 +L(e^(−x) ) L(e^(−x) ) =∫_0 ^∞ e^(−t) e^(−xt) dt =∫_0 ^∞ e^(−(1+x)t) dt =[−(1/(1+x))e^(−(1+x)t) ]_0 ^∞ =(1/(x+1)) so (e) ⇒(x^2 +3x+2)L(y) =x+5+(1/(x+1)) ⇒ L(y) =((x+5)/(x^2 +3x+2)) +(1/((x+1)(x^2 +3x+2))) ⇒y(x)=L^(−1) (((x+5)/(x^2 +3x+2)))+L^(−1) ((1/((x+1)(x^2 +3x+2)))) x^2 +3x+2 =x^2 −1 +3x+3 =(x−1)(x+1)+3(x+1) =(x+1)(x−1+3) =(x+1)(x+2) ⇒((x+5)/(x^2 +3x+2)) =((x+5)/((x+1)(x+2))) =(a/(x+1)) +(b/(x+2)) a =4 and b =(3/(−1))=−3 ⇒((x+5)/(x^2 +3x+2)) =(4/(x+1))−(3/(x+2)) ⇒ L^(−1) (((x+5)/(x^2 +3x+2))) =4L^(−1) ((1/(x+1)))−3L^(−1) ((1/(x+2))) =4e^(−x) −3e^(−2x) let decompose g(x) =(1/((x+1)(x^2 +3x+2))) ⇒g(x) =(1/((x+1)^2 (x+2))) =(a/(x+1)) +(b/((x+1)^2 )) +(c/(x+2)) b =1 and c =1 ⇒g(x) =(a/(x+1)) +(1/((x+1)^2 )) +(1/(x+2)) lim_(x→+∞) xg(x) =0 =a +1 ⇒a =−1 ⇒g(x) =−(1/(x+1)) +(1/((x+1)^2 )) +(1/(x+2)) ⇒ L^(−1) (g(x)) =−e^(−x) +xe^(−x) +e^(−2x) ⇒ y(x) =4e^(−x) −3e^(−2x) −e^(−x) +xe^(−x) +e^(−2x) =3e^(−x) −2e^(−2x) +xe^(−x) ⇒y(x) =(x+3)e^(−x) −2e^(−2x)](Q95831.png) | ||
| ||