Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 95951 by me2love2math last updated on 28/May/20

Commented by me2love2math last updated on 28/May/20

pls help out on this

$${pls}\:{help}\:{out}\:{on}\:{this}\: \\ $$

Commented by prakash jain last updated on 28/May/20

∫(dx/(x^2 (√(4−x^2 )))) x=2sin θ dx=2cos θdθ ∫((2cos θdθ)/((2sin θ)^2 (√(4−4sin^2 θ )))) =∫((2cos θdθ)/(4sin^2 θ∙2cos θ)) =(1/4)∫cosec^2 θdθ =−(1/4)cot θ+C =−(1/4)×((cos θ)/(sin θ))+C =−(1/4)×((√(1−sin^2 θ))/(sin θ))+C =−(1/4)×((√(1−((x/2))^2 ))/(x/2))+C =−(1/4)×((√(4−x^2 ))/x)+C Note: cos θ=(√(1−sin^2 θ)) when −(π/2)<θ<(π/2)

$$\int\frac{{dx}}{{x}^{\mathrm{2}} \sqrt{\mathrm{4}−{x}^{\mathrm{2}} }} \\ $$$${x}=\mathrm{2sin}\:\theta \\ $$$${dx}=\mathrm{2cos}\:\theta{d}\theta \\ $$$$\int\frac{\mathrm{2cos}\:\theta{d}\theta}{\left(\mathrm{2sin}\:\theta\right)^{\mathrm{2}} \sqrt{\mathrm{4}−\mathrm{4sin}^{\mathrm{2}} \theta\:}} \\ $$$$=\int\frac{\mathrm{2cos}\:\theta{d}\theta}{\mathrm{4sin}^{\mathrm{2}} \theta\centerdot\mathrm{2cos}\:\theta}\:\:\:\:\:\: \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\int\mathrm{cosec}^{\mathrm{2}} \theta{d}\theta \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}\mathrm{cot}\:\theta+{C} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}×\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta}+{C} \\ $$$$\: \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}×\frac{\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \theta}}{\mathrm{sin}\:\theta}+{C} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}×\frac{\sqrt{\mathrm{1}−\left(\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} }}{\frac{{x}}{\mathrm{2}}}+{C} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4}}×\frac{\sqrt{\mathrm{4}−{x}^{\mathrm{2}} }}{{x}}+{C} \\ $$$$ \\ $$$${Note}:\:\mathrm{cos}\:\theta=\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \theta}\:{when}\:−\frac{\pi}{\mathrm{2}}<\theta<\frac{\pi}{\mathrm{2}} \\ $$

Commented by me2love2math last updated on 28/May/20

Thanks...pls Q2 and Q3 in particular

$${Thanks}...{pls}\:{Q}\mathrm{2}\:{and}\:{Q}\mathrm{3}\:{in}\:{particular} \\ $$

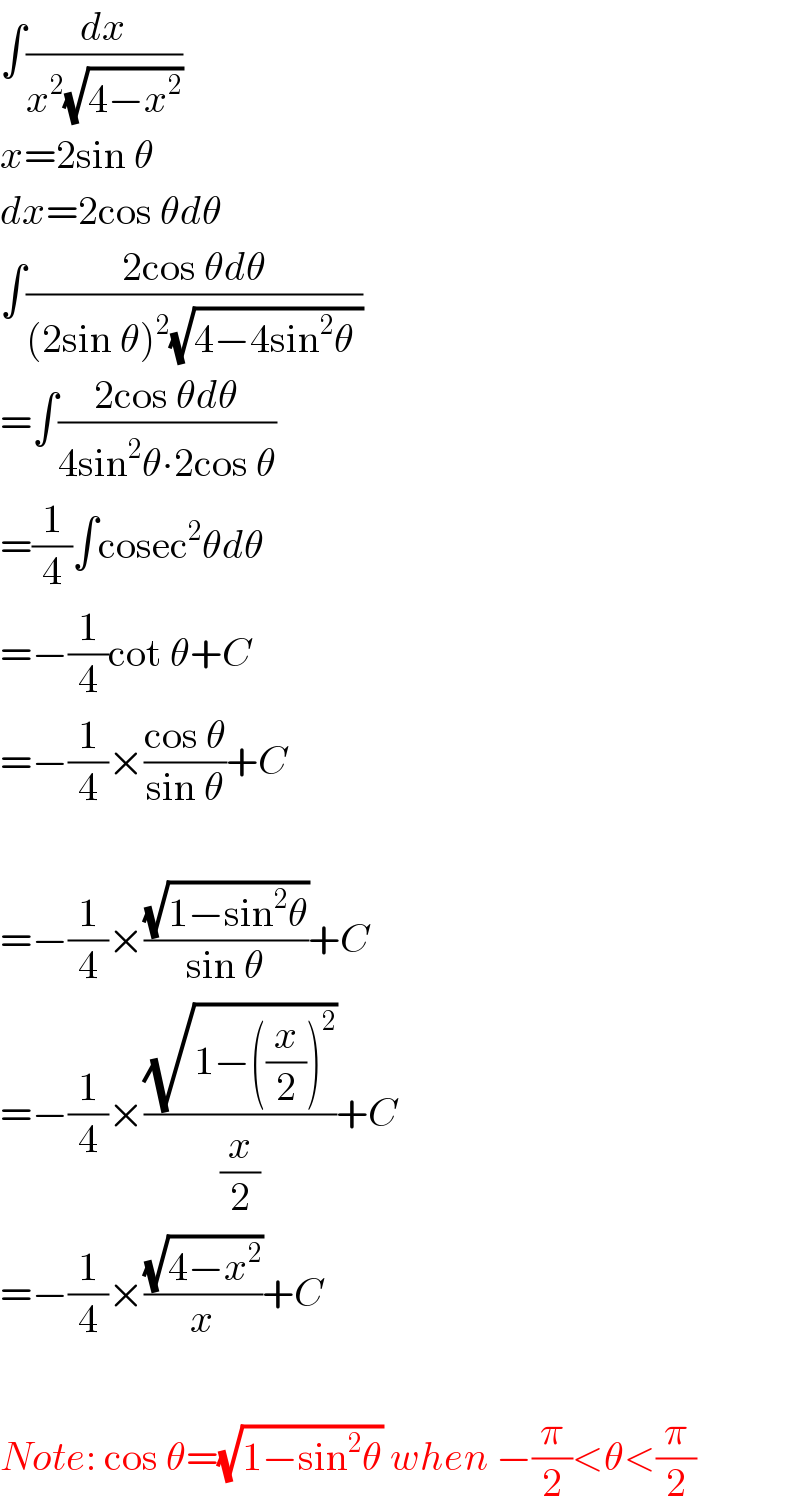
Answered by abdomathmax last updated on 28/May/20

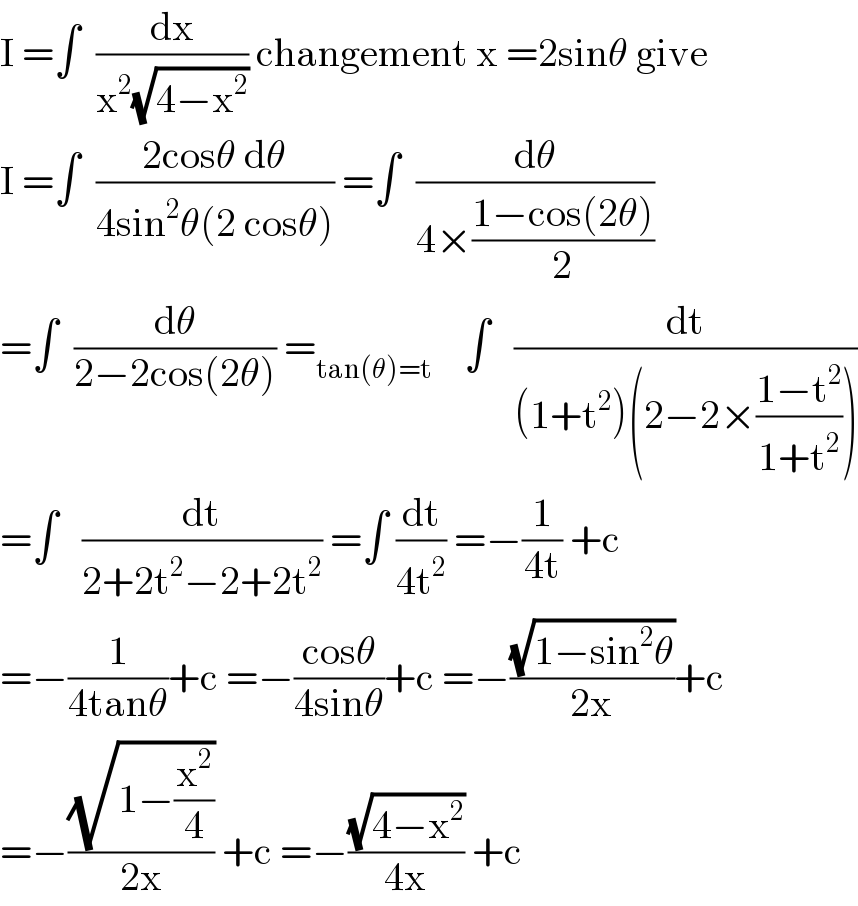
I =∫ (dx/(x^2 (√(4−x^2 )))) changement x =2sinθ give I =∫ ((2cosθ dθ)/(4sin^2 θ(2 cosθ))) =∫ (dθ/(4×((1−cos(2θ))/2))) =∫ (dθ/(2−2cos(2θ))) =_(tan(θ)=t) ∫ (dt/((1+t^2 )(2−2×((1−t^2 )/(1+t^2 ))))) =∫ (dt/(2+2t^2 −2+2t^2 )) =∫ (dt/(4t^2 )) =−(1/(4t)) +c =−(1/(4tanθ))+c =−((cosθ)/(4sinθ))+c =−((√(1−sin^2 θ))/(2x))+c =−((√(1−(x^2 /4)))/(2x)) +c =−((√(4−x^2 ))/(4x)) +c

$$\mathrm{I}\:=\int\:\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} \sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}\:\mathrm{changement}\:\mathrm{x}\:=\mathrm{2sin}\theta\:\mathrm{give} \\ $$$$\mathrm{I}\:=\int\:\:\frac{\mathrm{2cos}\theta\:\mathrm{d}\theta}{\mathrm{4sin}^{\mathrm{2}} \theta\left(\mathrm{2}\:\mathrm{cos}\theta\right)}\:=\int\:\:\frac{\mathrm{d}\theta}{\mathrm{4}×\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}} \\ $$$$=\int\:\:\frac{\mathrm{d}\theta}{\mathrm{2}−\mathrm{2cos}\left(\mathrm{2}\theta\right)}\:=_{\mathrm{tan}\left(\theta\right)=\mathrm{t}} \:\:\:\:\int\:\:\:\frac{\mathrm{dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\mathrm{2}−\mathrm{2}×\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }\right)} \\ $$$$=\int\:\:\:\frac{\mathrm{dt}}{\mathrm{2}+\mathrm{2t}^{\mathrm{2}} −\mathrm{2}+\mathrm{2t}^{\mathrm{2}} }\:=\int\:\frac{\mathrm{dt}}{\mathrm{4t}^{\mathrm{2}} }\:=−\frac{\mathrm{1}}{\mathrm{4t}}\:+\mathrm{c} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{4tan}\theta}+\mathrm{c}\:=−\frac{\mathrm{cos}\theta}{\mathrm{4sin}\theta}+\mathrm{c}\:=−\frac{\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \theta}}{\mathrm{2x}}+\mathrm{c} \\ $$$$=−\frac{\sqrt{\mathrm{1}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{4}}}}{\mathrm{2x}}\:+\mathrm{c}\:=−\frac{\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }}{\mathrm{4x}}\:+\mathrm{c} \\ $$

Commented by me2love2math last updated on 28/May/20

thx...Q2 and Q3 is where i have issues

$${thx}...{Q}\mathrm{2}\:{and}\:{Q}\mathrm{3}\:{is}\:{where}\:{i}\:{have}\:{issues} \\ $$

Answered by Sourav mridha last updated on 28/May/20

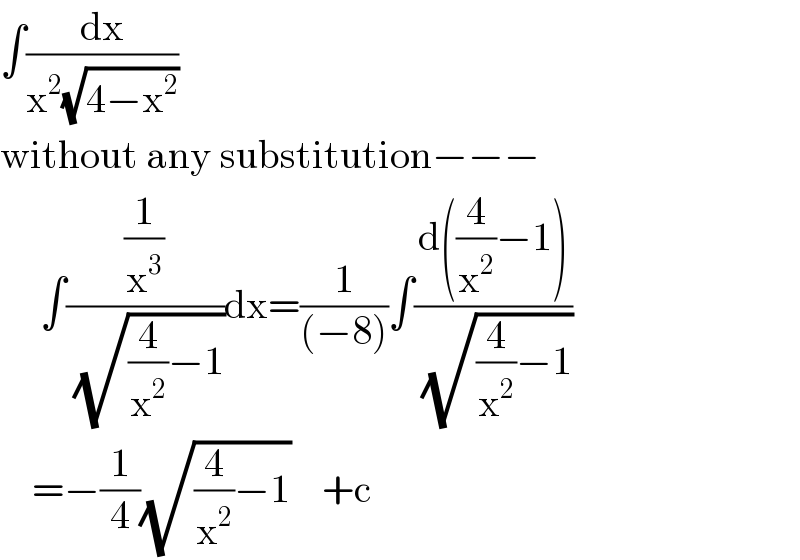
∫(dx/(x^2 (√(4−x^2 )))) without any substitution−−− ∫((1/x^3 )/(√((4/x^2 )−1)))dx=(1/((−8)))∫((d((4/x^2 )−1))/(√((4/x^2 )−1))) =−(1/4)(√((4/x^2 )−1)) +c

$$\int\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} \sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }} \\ $$$$\mathrm{without}\:\mathrm{any}\:\mathrm{substitution}−−−\:\:\: \\ $$$$\:\:\:\:\:\int\frac{\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{3}} }}{\sqrt{\frac{\mathrm{4}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}}}\mathrm{dx}=\frac{\mathrm{1}}{\left(−\mathrm{8}\right)}\int\frac{\mathrm{d}\left(\frac{\mathrm{4}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}\right)}{\sqrt{\frac{\mathrm{4}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}}} \\ $$$$\:\:\:\:=−\frac{\mathrm{1}}{\mathrm{4}}\sqrt{\frac{\mathrm{4}}{\mathrm{x}^{\mathrm{2}} }−\mathrm{1}}\:\:\:\:+\mathrm{c} \\ $$

Answered by john santu last updated on 29/May/20

(2) ∫_0 ^1 [∫_x ^(√x) (60x^2 y−40y^3 )dy] dx the inner bracketed integration is carried out first and evaluated as [ 30x^2 y^2 −10y^4 ]_x ^(√x) = 30x^2 (x−x^2 )−10(x^2 −x^4 ) = 30x^3 −30x^4 −10x^2 +10x^4 = 30x^3 −20x^4 −10x^2 so the integration becomes ∫_0 ^1 (30x^3 −20x^4 −10x^2 ) dx = [((30)/4)x^4 −4x^5 −((10)/3)x^3 ]_0 ^1 = ((15)/2)−4−((10)/3) = ((45−24−20)/6) = (1/6) . done

$$\left(\mathrm{2}\right)\:\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\left[\underset{\mathrm{x}} {\overset{\sqrt{\mathrm{x}}} {\int}}\:\left(\mathrm{60x}^{\mathrm{2}} \mathrm{y}−\mathrm{40y}^{\mathrm{3}} \right)\mathrm{dy}\right]\:\mathrm{dx}\: \\ $$$$\mathrm{the}\:\mathrm{inner}\:\mathrm{bracketed}\:\mathrm{integration} \\ $$$$\mathrm{is}\:\mathrm{carried}\:\mathrm{out}\:\mathrm{first}\:\mathrm{and}\:\mathrm{evaluated} \\ $$$$\mathrm{as}\:\left[\:\mathrm{30x}^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} −\mathrm{10y}^{\mathrm{4}} \:\right]_{\mathrm{x}} ^{\sqrt{\mathrm{x}}} =\: \\ $$$$\mathrm{30x}^{\mathrm{2}} \left(\mathrm{x}−\mathrm{x}^{\mathrm{2}} \right)−\mathrm{10}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{\mathrm{4}} \right)\:= \\ $$$$\mathrm{30x}^{\mathrm{3}} −\mathrm{30x}^{\mathrm{4}} −\mathrm{10x}^{\mathrm{2}} +\mathrm{10x}^{\mathrm{4}} = \\ $$$$\mathrm{30x}^{\mathrm{3}} −\mathrm{20x}^{\mathrm{4}} −\mathrm{10x}^{\mathrm{2}} \\ $$$$\mathrm{so}\:\mathrm{the}\:\mathrm{integration}\:\mathrm{becomes}\: \\ $$$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\left(\mathrm{30x}^{\mathrm{3}} −\mathrm{20x}^{\mathrm{4}} −\mathrm{10x}^{\mathrm{2}} \right)\:\mathrm{dx}\:= \\ $$$$\left[\frac{\mathrm{30}}{\mathrm{4}}\mathrm{x}^{\mathrm{4}} −\mathrm{4x}^{\mathrm{5}} −\frac{\mathrm{10}}{\mathrm{3}}\mathrm{x}^{\mathrm{3}} \:\right]_{\mathrm{0}} ^{\mathrm{1}} = \\ $$$$\frac{\mathrm{15}}{\mathrm{2}}−\mathrm{4}−\frac{\mathrm{10}}{\mathrm{3}}\:=\:\frac{\mathrm{45}−\mathrm{24}−\mathrm{20}}{\mathrm{6}}\: \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{6}}\:.\:\mathrm{done}\:\: \\ $$

Commented by me2love2math last updated on 29/May/20

thx so much

$${thx}\:{so}\:{much} \\ $$

Answered by john santu last updated on 29/May/20

(3)f(x,y) = 2xy−(1/2)(x^2 +y^2 )+x^2 f(x,y) = 2xy +(1/2)x^2 −(1/2)y^2 firstly find all first and second partial derivatives of f(x,y) . they are f_x =2y+x ; f_y =2x−y f_(xx) = 1 ; f_(yy) = −1 f_(xy) =f_(yx) = 2 setting f_x =0 , yields x=−2y substitution into f_y yields −5y=0 y=0 . this signal possible extrema at (0,0) as x=−2y evaluate D at (0,0) D = 4y^2 −4((1/2))(−(1/2)y^2 ) D = 0 , there is an extremum and as f_(xx) > 0 it is a minimum therefore f(0,0) = 0 is a local minimum. (done)

$$\left(\mathrm{3}\right)\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)\:=\:\mathrm{2xy}−\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} \right)+\mathrm{x}^{\mathrm{2}} \\ $$$$\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)\:=\:\mathrm{2xy}\:+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{x}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{y}^{\mathrm{2}} \\ $$$$\mathrm{firstly}\:\mathrm{find}\:\mathrm{all}\:\mathrm{first}\:\mathrm{and}\:\mathrm{second}\: \\ $$$$\mathrm{partial}\:\mathrm{derivatives}\:\mathrm{of}\:\mathrm{f}\left(\mathrm{x},\mathrm{y}\right)\:.\:\mathrm{they} \\ $$$$\mathrm{are}\:{f}_{{x}} =\mathrm{2}{y}+{x}\:;\:{f}_{{y}} =\mathrm{2}{x}−{y} \\ $$$${f}_{{xx}} \:=\:\mathrm{1}\:;\:{f}_{{yy}} \:=\:−\mathrm{1} \\ $$$${f}_{{xy}} ={f}_{{yx}} \:=\:\mathrm{2} \\ $$$${setting}\:{f}_{{x}} =\mathrm{0}\:,\:\mathrm{yields}\:{x}=−\mathrm{2}{y} \\ $$$${substitution}\:{into}\:{f}_{{y}} \:{yields}\:−\mathrm{5}{y}=\mathrm{0} \\ $$$${y}=\mathrm{0}\:.\:{this}\:{signal}\:{possible}\:{extrema} \\ $$$${at}\:\left(\mathrm{0},\mathrm{0}\right)\:\mathrm{as}\:\mathrm{x}=−\mathrm{2y} \\ $$$$\mathrm{evaluate}\:\mathrm{D}\:\mathrm{at}\:\left(\mathrm{0},\mathrm{0}\right)\: \\ $$$$\mathrm{D}\:=\:\mathrm{4y}^{\mathrm{2}} −\mathrm{4}\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\left(−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{y}^{\mathrm{2}} \right) \\ $$$$\mathrm{D}\:=\:\mathrm{0}\:,\:\mathrm{there}\:\mathrm{is}\:\mathrm{an}\:\mathrm{extremum} \\ $$$$\mathrm{and}\:\mathrm{as}\:{f}_{{xx}} \:>\:\mathrm{0}\:{it}\:{is}\:{a}\:{minimum} \\ $$$${therefore}\:{f}\left(\mathrm{0},\mathrm{0}\right)\:=\:\mathrm{0}\:\mathrm{is}\:\mathrm{a}\:\mathrm{local} \\ $$$$\mathrm{minimum}.\:\left(\mathrm{done}\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com