
Question Number 96244 by Don08q last updated on 31/May/20
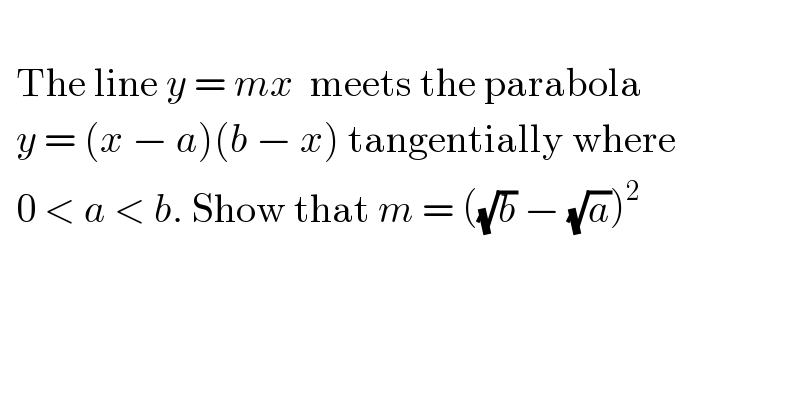
$$ \\ $$ $$\:\:\mathrm{The}\:\mathrm{line}\:{y}\:=\:{mx}\:\:\mathrm{meets}\:\mathrm{the}\:\mathrm{parabola} \\ $$ $$\:\:{y}\:=\:\left({x}\:−\:{a}\right)\left({b}\:−\:{x}\right)\:\mathrm{tangentially}\:\mathrm{where} \\ $$ $$\:\:\mathrm{0}\:<\:{a}\:<\:{b}.\:\mathrm{Show}\:\mathrm{that}\:{m}\:=\:\left(\sqrt{{b}}\:−\:\sqrt{{a}}\right)^{\mathrm{2}} \\ $$ $$ \\ $$
Commented bybobhans last updated on 31/May/20

$$\mathrm{y}\:=\:−{x}^{\mathrm{2}} +{bx}+{ax}−{ab}\: \\ $$ $${y}\:=\:−{x}^{\mathrm{2}} +\left({a}+{b}\right){x}−{ab}\: \\ $$ $${with}\:{slope}\:=\:−\mathrm{2}{x}+{a}+{b}\:=\:{m}\: \\ $$ $${x}\:=\:\frac{{a}+{b}−{m}}{\mathrm{2}}\:\Rightarrow\:−{x}^{\mathrm{2}} +\left({a}+{b}\right){x}−{ab}={mx} \\ $$ $${x}^{\mathrm{2}} +\left({m}−{a}−{b}\right){x}+{ab}\:=\:\mathrm{0} \\ $$ $$\mathrm{set}\:{a}+{b}\:=\:{p} \\ $$ $$\Rightarrow\left(\frac{{p}−{m}}{\mathrm{2}}\right)^{\mathrm{2}} +\left({m}−{p}\right)\left(\frac{{p}−{m}}{\mathrm{2}}\right)+{ab}\:=\:\mathrm{0} \\ $$ $$−\left({p}−{m}\right)^{\mathrm{2}} \:=\:−\mathrm{4}{ab}\:\Rightarrow{p}−{m}\:=\:\pm\:\mathrm{2}\sqrt{{ab}} \\ $$ $${m}\:=\:{p}\:\pm\mathrm{2}\sqrt{{ab}}\:=\:\left({a}+{b}\pm\mathrm{2}\sqrt{{ab}}\right) \\ $$ $${m}\:=\:\left(\sqrt{{a}}\:\pm\:\sqrt{{b}}\:\right)^{\mathrm{2}} \: \\ $$
Commented byDon08q last updated on 31/May/20

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{Sir}. \\ $$
Answered by Smail last updated on 31/May/20

$${mx}=\left({x}−{a}\right)\left({b}−{x}\right)\:{at}\:{the}\:{intersection} \\ $$ $${mx}=−{x}^{\mathrm{2}} +\left({b}+{a}\right){x}−{ab}\: \\ $$ $${x}^{\mathrm{2}} −\left({a}+{b}−{m}\right){x}+{ab}=\mathrm{0} \\ $$ $${Since}\:{the}\:{line}\:{is}\:{tangent}\: \\ $$ $${to}\:{the}\:{parabola}\::\:\Delta=\mathrm{0}=\left({a}+{b}−{m}\right)^{\mathrm{2}} −\mathrm{4}{ab} \\ $$ $${a}+{b}−{m}=\underset{−} {+}\mathrm{2}\sqrt{{ab}} \\ $$ $${a}+{b}\underset{−} {+}\mathrm{2}\sqrt{{ab}}={m} \\ $$ $${m}=\left(\sqrt{{a}}\underset{−} {+}\sqrt{{b}}\right)^{\mathrm{2}} \\ $$ $${Thus},\:{there}\:{are}\:{two}\:{passible} \\ $$ $${lines}\:{whose}\:{slopes}\:{are}\:{m}_{\mathrm{1}} =\left(\sqrt{{a}}−\sqrt{{b}}\right)^{\mathrm{2}} \\ $$ $${and}\:{m}_{\mathrm{2}} =\left(\sqrt{{a}}+\sqrt{{b}}\right)^{\mathrm{2}} \\ $$
Commented byDon08q last updated on 31/May/20

$$\:\mathrm{Thank}\:\mathrm{you}\:\mathrm{Sir}. \\ $$
