Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 96656 by mathmax by abdo last updated on 03/Jun/20

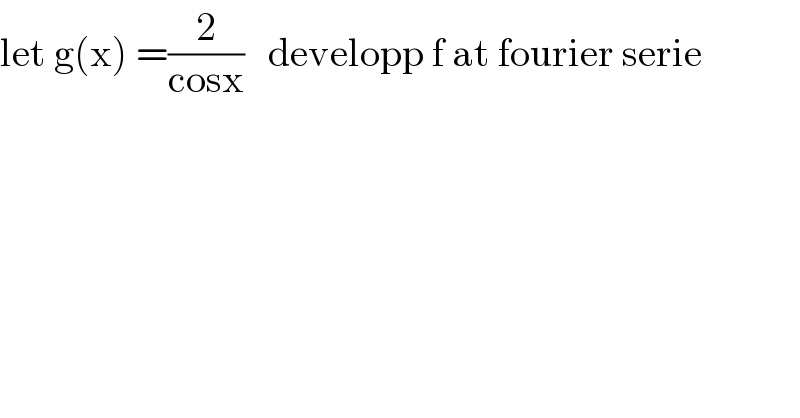
let g(x) =(2/(cosx)) developp f at fourier serie

$$\mathrm{let}\:\mathrm{g}\left(\mathrm{x}\right)\:=\frac{\mathrm{2}}{\mathrm{cosx}}\:\:\:\mathrm{developp}\:\mathrm{f}\:\mathrm{at}\:\mathrm{fourier}\:\mathrm{serie} \\ $$

Answered by mathmax by abdo last updated on 04/Jun/20

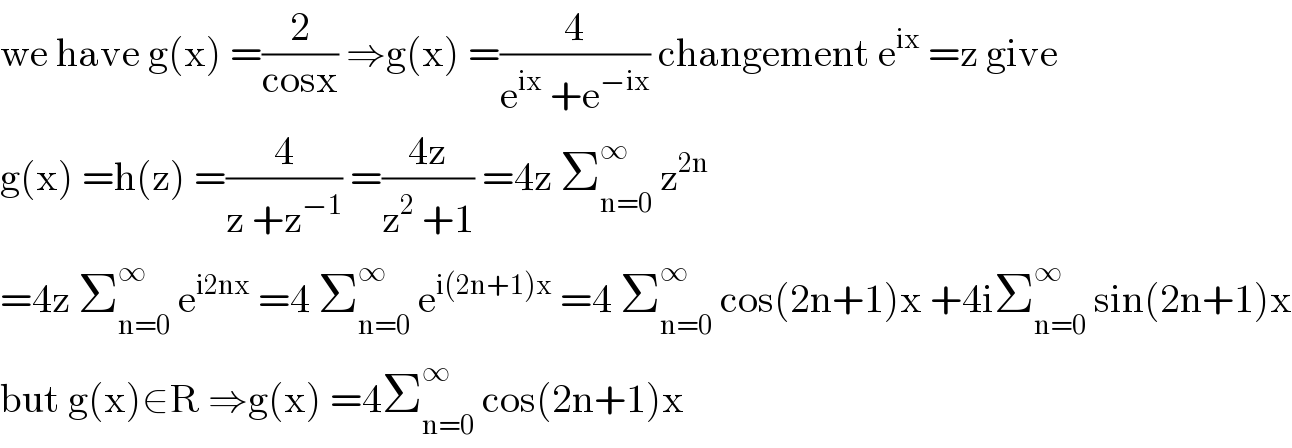
we have g(x) =(2/(cosx)) ⇒g(x) =(4/(e^(ix) +e^(−ix) )) changement e^(ix) =z give g(x) =h(z) =(4/(z +z^(−1) )) =((4z)/(z^2 +1)) =4z Σ_(n=0) ^∞ z^(2n) =4z Σ_(n=0) ^∞ e^(i2nx) =4 Σ_(n=0) ^∞ e^(i(2n+1)x) =4 Σ_(n=0) ^∞ cos(2n+1)x +4iΣ_(n=0) ^∞ sin(2n+1)x but g(x)∈R ⇒g(x) =4Σ_(n=0) ^∞ cos(2n+1)x

$$\mathrm{we}\:\mathrm{have}\:\mathrm{g}\left(\mathrm{x}\right)\:=\frac{\mathrm{2}}{\mathrm{cosx}}\:\Rightarrow\mathrm{g}\left(\mathrm{x}\right)\:=\frac{\mathrm{4}}{\mathrm{e}^{\mathrm{ix}} \:+\mathrm{e}^{−\mathrm{ix}} }\:\mathrm{changement}\:\mathrm{e}^{\mathrm{ix}} \:=\mathrm{z}\:\mathrm{give} \\ $$$$\mathrm{g}\left(\mathrm{x}\right)\:=\mathrm{h}\left(\mathrm{z}\right)\:=\frac{\mathrm{4}}{\mathrm{z}\:+\mathrm{z}^{−\mathrm{1}} }\:=\frac{\mathrm{4z}}{\mathrm{z}^{\mathrm{2}} \:+\mathrm{1}}\:=\mathrm{4z}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{z}^{\mathrm{2n}} \\ $$$$=\mathrm{4z}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{e}^{\mathrm{i2nx}} \:=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{e}^{\mathrm{i}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}} \:=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\:+\mathrm{4i}\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{sin}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x} \\ $$$$\mathrm{but}\:\mathrm{g}\left(\mathrm{x}\right)\in\mathrm{R}\:\Rightarrow\mathrm{g}\left(\mathrm{x}\right)\:=\mathrm{4}\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\: \\ $$

Commented by 1549442205 last updated on 04/Jun/20

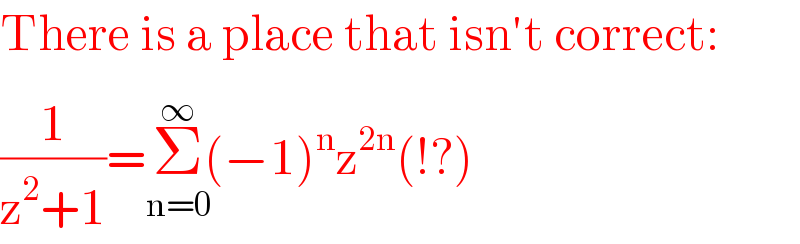
There is a place that isn′t correct: (1/(z^2 +1))=Σ_(n=0) ^(∞) (−1)^n z^(2n) (!?)

$$\mathrm{There}\:\mathrm{is}\:\mathrm{a}\:\mathrm{place}\:\mathrm{that}\:\mathrm{isn}'\mathrm{t}\:\mathrm{correct}: \\ $$$$\frac{\mathrm{1}}{\mathrm{z}^{\mathrm{2}} +\mathrm{1}}=\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\Sigma}}\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{z}^{\mathrm{2n}} \left(!?\right) \\ $$

Commented by Rio Michael last updated on 04/Jun/20

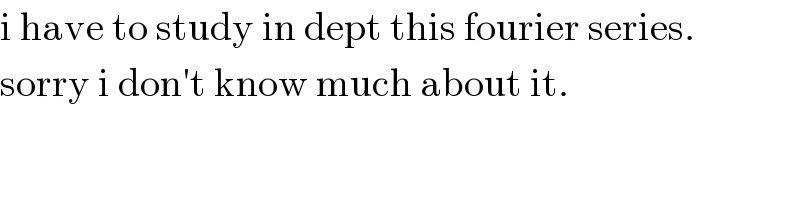
i have to study in dept this fourier series. sorry i don′t know much about it.

$$\mathrm{i}\:\mathrm{have}\:\mathrm{to}\:\mathrm{study}\:\mathrm{in}\:\mathrm{dept}\:\mathrm{this}\:\mathrm{fourier}\:\mathrm{series}. \\ $$$$\mathrm{sorry}\:\mathrm{i}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{much}\:\mathrm{about}\:\mathrm{it}. \\ $$

Commented by abdomathmax last updated on 04/Jun/20

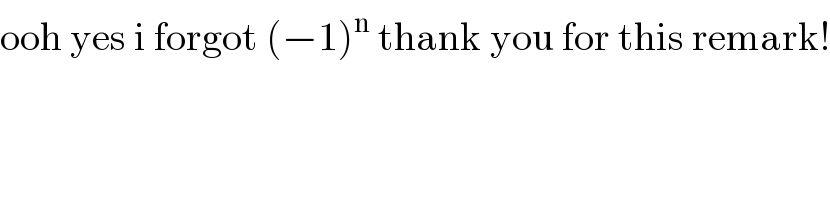
ooh yes i forgot (−1)^n thank you for this remark!

$$\mathrm{ooh}\:\mathrm{yes}\:\mathrm{i}\:\mathrm{forgot}\:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{thank}\:\mathrm{you}\:\mathrm{for}\:\mathrm{this}\:\mathrm{remark}! \\ $$

Commented by abdomathmax last updated on 04/Jun/20

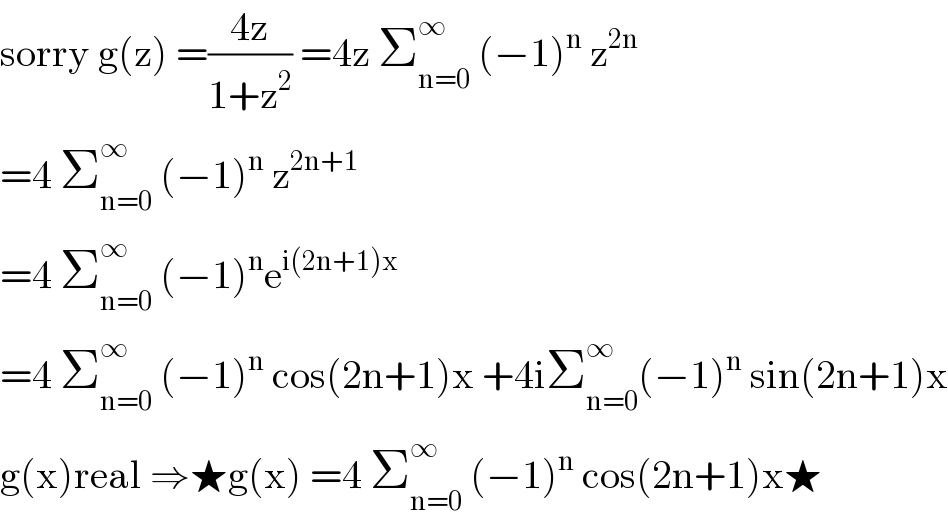
sorry g(z) =((4z)/(1+z^2 )) =4z Σ_(n=0) ^∞ (−1)^n z^(2n) =4 Σ_(n=0) ^∞ (−1)^n z^(2n+1) =4 Σ_(n=0) ^∞ (−1)^n e^(i(2n+1)x) =4 Σ_(n=0) ^∞ (−1)^n cos(2n+1)x +4iΣ_(n=0) ^∞ (−1)^n sin(2n+1)x g(x)real ⇒★g(x) =4 Σ_(n=0) ^∞ (−1)^n cos(2n+1)x★

$$\mathrm{sorry}\:\mathrm{g}\left(\mathrm{z}\right)\:=\frac{\mathrm{4z}}{\mathrm{1}+\mathrm{z}^{\mathrm{2}} }\:=\mathrm{4z}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{z}^{\mathrm{2n}} \\ $$$$=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{z}^{\mathrm{2n}+\mathrm{1}} \\ $$$$=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \mathrm{e}^{\mathrm{i}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}} \\ $$$$=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\:+\mathrm{4i}\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{sin}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x} \\ $$$$\mathrm{g}\left(\mathrm{x}\right)\mathrm{real}\:\Rightarrow\bigstar\mathrm{g}\left(\mathrm{x}\right)\:=\mathrm{4}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{\mathrm{n}} \:\mathrm{cos}\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{x}\bigstar \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com