
Question and Answers Forum
Question Number 97235 by bobhans last updated on 07/Jun/20
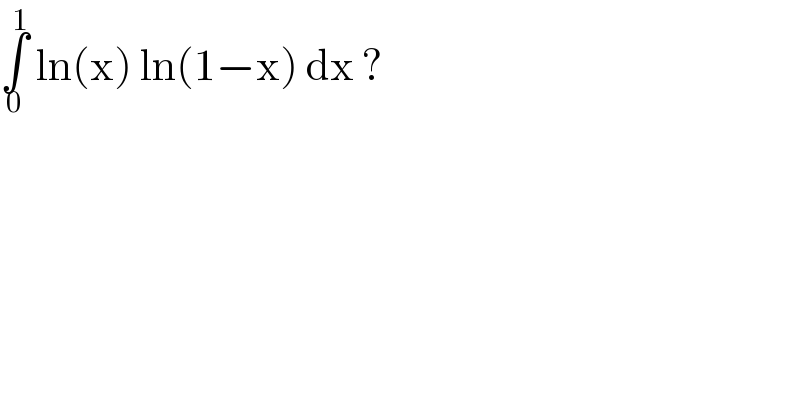
Answered by abdomathmax last updated on 07/Jun/20
![I =∫_0 ^1 lnxln(1−x)dx we have for ∣x∣<1 (d/dx)ln(1−x) =((−1)/(1−x)) =−Σ_(n=0) ^∞ x^n ⇒ ln(1−x) =−Σ_(n=0) ^∞ (x^(n+1) /(n+1)) =−Σ_(n=1) ^∞ (x^n /n) ⇒ I =−∫_0 ^1 ln(x)(Σ_(n=1) ^∞ (x^n /n)) =−Σ_(n=1) ^∞ (1/n) ∫_0 ^1 x^n ln(x)dx by parts A_n =∫_0 ^1 x^n ln(x)dx =[(x^(n+1) /(n+1))ln(x)]_0 ^1 −∫_0 ^1 (x^(n+1) /(n+1))(dx/x) =−(1/(n+1)) ∫_0 ^(1 ) x^n dx =−(1/((n+1)^2 )) ⇒I =Σ_(n=1) ^∞ (1/(n(n+1)^2 )) let decompose F(x)=(1/(x(x+1)^2 )) ⇒ F(x) =(a/x) +(b/(x+1)) +(c/((x+1)^2 )) a =1 , c =−1 lim_(x→+∞) xF(x) =0 =a+b ⇒b=−1 ⇒ F(x) =(1/x)−(1/(x+1))−(1/((x+1)^2 )) ⇒ I =Σ_(n=1) ^∞ ((1/n)−(1/(n+1))) −Σ_(n=1) ^∞ (1/((n+1)^2 )) Σ_(n=1) ^∞ ((1/n)−(1/(n+1)))=lim_(n→∞) Σ_(k=1) ^n ((1/k)−(1/(k+1))) lim_(n→∞) (1−(1/(n+1))) =1 Σ_(n=1) ^∞ (1/((n+1)^2 )) =Σ_(n=2) ^∞ (1/n^2 ) =(π^2 /6)−1 ⇒ I =1−((π^2 /6)−1) =2−(π^2 /6)](Q97278.png)
Commented by bobhans last updated on 07/Jun/20

| ||
Question and Answers Forum | ||
Question Number 97235 by bobhans last updated on 07/Jun/20 | ||
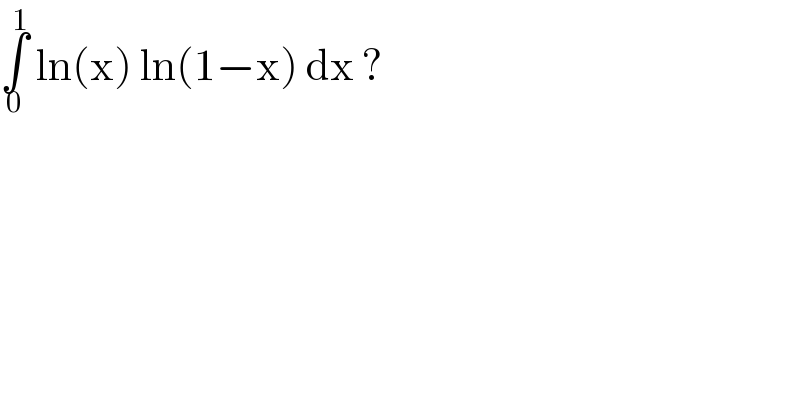 | ||
Answered by abdomathmax last updated on 07/Jun/20 | ||
![I =∫_0 ^1 lnxln(1−x)dx we have for ∣x∣<1 (d/dx)ln(1−x) =((−1)/(1−x)) =−Σ_(n=0) ^∞ x^n ⇒ ln(1−x) =−Σ_(n=0) ^∞ (x^(n+1) /(n+1)) =−Σ_(n=1) ^∞ (x^n /n) ⇒ I =−∫_0 ^1 ln(x)(Σ_(n=1) ^∞ (x^n /n)) =−Σ_(n=1) ^∞ (1/n) ∫_0 ^1 x^n ln(x)dx by parts A_n =∫_0 ^1 x^n ln(x)dx =[(x^(n+1) /(n+1))ln(x)]_0 ^1 −∫_0 ^1 (x^(n+1) /(n+1))(dx/x) =−(1/(n+1)) ∫_0 ^(1 ) x^n dx =−(1/((n+1)^2 )) ⇒I =Σ_(n=1) ^∞ (1/(n(n+1)^2 )) let decompose F(x)=(1/(x(x+1)^2 )) ⇒ F(x) =(a/x) +(b/(x+1)) +(c/((x+1)^2 )) a =1 , c =−1 lim_(x→+∞) xF(x) =0 =a+b ⇒b=−1 ⇒ F(x) =(1/x)−(1/(x+1))−(1/((x+1)^2 )) ⇒ I =Σ_(n=1) ^∞ ((1/n)−(1/(n+1))) −Σ_(n=1) ^∞ (1/((n+1)^2 )) Σ_(n=1) ^∞ ((1/n)−(1/(n+1)))=lim_(n→∞) Σ_(k=1) ^n ((1/k)−(1/(k+1))) lim_(n→∞) (1−(1/(n+1))) =1 Σ_(n=1) ^∞ (1/((n+1)^2 )) =Σ_(n=2) ^∞ (1/n^2 ) =(π^2 /6)−1 ⇒ I =1−((π^2 /6)−1) =2−(π^2 /6)](Q97278.png) | ||
| ||
Commented by bobhans last updated on 07/Jun/20 | ||
 | ||