Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 97794 by abdomathmax last updated on 09/Jun/20

$$\mathrm{solve}{\mathrm{y}}^{{}^{″}}+\mathrm{y}=\frac{1}{\mathrm{cosx}}$$

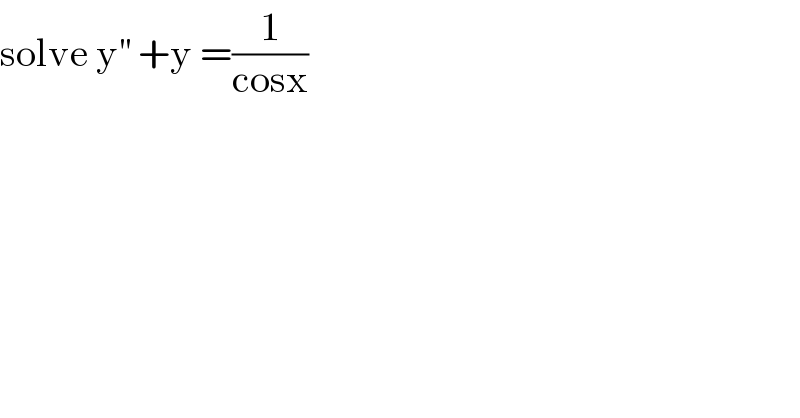
Commented by john santu last updated on 10/Jun/20

$${\mathrm{y}}^{″}+{\mathrm{y}}^{\prime }=\sec \mathrm{x}$$$$\mathrm{homogenous}\mathrm{solution}$$$${\mathrm{y}}_{\mathrm{h}}={\mathrm{C}}_1\cos \mathrm{x}+{\mathrm{C}}_2\sin \mathrm{x}$$$$\mathrm{particular}\mathrm{solution}$$$${\mathrm{y}}_{\mathrm{p}}={\mathrm{v}}_1\left(\mathrm{x}\right)\cos \mathrm{x}+{\mathrm{v}}_2\left(\mathrm{x}\right)\sin \mathrm{x}$$$$\mathrm{where}{\mathrm{v}}_1^{\prime }\cos \mathrm{x}+{\mathrm{v}}_2^{\prime }\sin \mathrm{x}=0$$$$-{\mathrm{v}}_1^{\prime }\sin \mathrm{x}+{\mathrm{v}}_2^{\prime }\cos \mathrm{x}=\sec \mathrm{x}$$$$\mathrm{we}\mathrm{get}\{\begin{array}{l}{\mathrm{v}}_1\left(\mathrm{x}\right)=\int -\tan \mathrm{x}\mathrm{dx}=\ln \mid \cos \mathrm{x}\mid \\ {\mathrm{v}}_2\left(\mathrm{x}\right)=\int \mathrm{dx}=\mathrm{x}\end{array}$$$$\mathrm{so}{\mathrm{y}}_{\mathrm{p}}=(\ln \mid \cos \mathrm{x}\mid )\cos \mathrm{x}+\sin \mathrm{x}$$$$\mathrm{generall}\mathrm{solution}$$$$\mathrm{y}=(\ln \mid \cos \mathrm{x}\mid )\cos \mathrm{x}+\sin \mathrm{x}+{\mathrm{C}}_1\cos \mathrm{x}+{\mathrm{C}}_2\sin \mathrm{x}◼$$

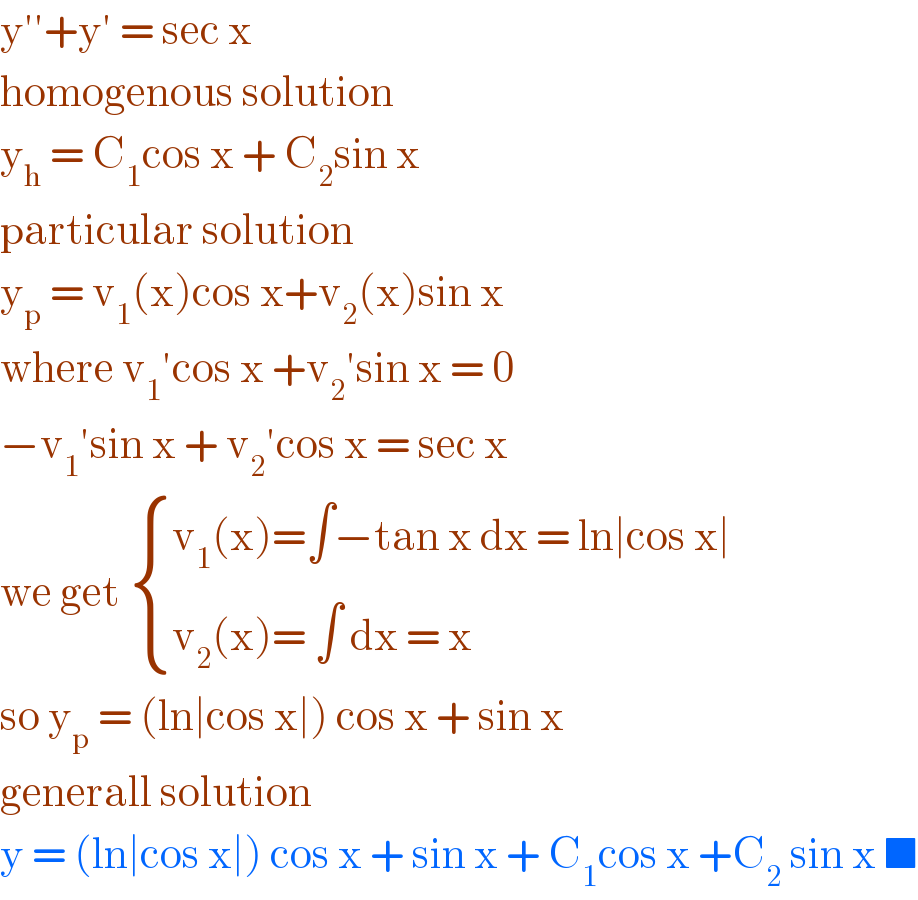
Commented by bobhans last updated on 10/Jun/20

nice.....very...nice

Commented by bemath last updated on 10/Jun/20

very....afdolll

Answered by niroj last updated on 09/Jun/20

$${\mathrm{y}}^{{}^{″}}+\mathrm{y}=\frac{1}{\cos \mathrm{x}}$$$$\frac{{\mathrm{d}}^2\mathrm{y}}{{\mathrm{dx}}^2}+\mathrm{y}=\frac{1}{\cos \mathrm{x}}$$$$({\mathrm{D}}^2+1)\mathrm{y}=\sec \mathrm{x}$$$$\mathrm{A}.\mathrm{E}.,{\mathrm{m}}^2+1=0$$$$\mathrm{m}=0\stackrel{-}{+}1\mathrm{i}$$$$\mathrm{CF}={\mathrm{C}}_1\cos \mathrm{x}+{\mathrm{C}}_2\sin \mathrm{x}$$$$\mathrm{Here},\mathrm{By}\mathrm{the}\mathrm{help}\mathrm{of}\mathrm{variation}\mathrm{parameters}:$$$${\mathrm{y}}_1=\cos \mathrm{x},{\mathrm{y}}_2=\sin \mathrm{x},\mathrm{w}=\sec \mathrm{x}$$$$\mathrm{W}=\left|\begin{array}{c}{\mathrm{y}}_1{\mathrm{y}}_2\\ {\mathrm{y}}_1^{{}^{\prime }}{\mathrm{y}}_2^{{}^{\prime }}\end{array}\right|$$$$\mathrm{W}=\left|\begin{array}{c}\cos \mathrm{x}\sin \mathrm{x}\\ -\sin \mathrm{x}\cos \mathrm{x}\end{array}\right|$$$$\mathrm{W}={\cos }^2\mathrm{x}-(-{\sin }^2\mathrm{x})=1$$$$\mathrm{W}=1$$$$\mathrm{PI}=-{\mathrm{y}}_1\int \frac{{\mathrm{y}}_2\mathrm{x}}{\mathrm{w}}\mathrm{dx}+{\mathrm{y}}_2\int \frac{{\mathrm{y}}_1\mathrm{x}}{\mathrm{w}}\mathrm{dx}$$$$=-\mathrm{cosx}\int \frac{\sin \mathrm{x}\sec \mathrm{x}}{1}\mathrm{dx}+\sin \mathrm{x}\int \frac{\cos \mathrm{x}\sec \mathrm{x}}{1}\mathrm{dx}$$$$=-\cos \mathrm{x}\int \tan \mathrm{x}\mathrm{dx}+\mathrm{sinx}\int \mathrm{dx}$$$$=-\cos \mathrm{x}\log \left(\sec \mathrm{x}\right)+\sin \mathrm{x}.\mathrm{x}$$$$\mathrm{y}=\mathrm{CF}+\mathrm{PI}$$$$\therefore \mathrm{y}={\mathrm{C}}_1\cos \mathrm{x}+{\mathrm{C}}_2\sin \mathrm{x}-\cos \mathrm{x}\log \left(\sec \mathrm{x}\right)+\mathrm{x}\sin \mathrm{xo}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com