
Question and Answers Forum
Question Number 97928 by M±th+et+s last updated on 10/Jun/20

Answered by abdomathmax last updated on 10/Jun/20
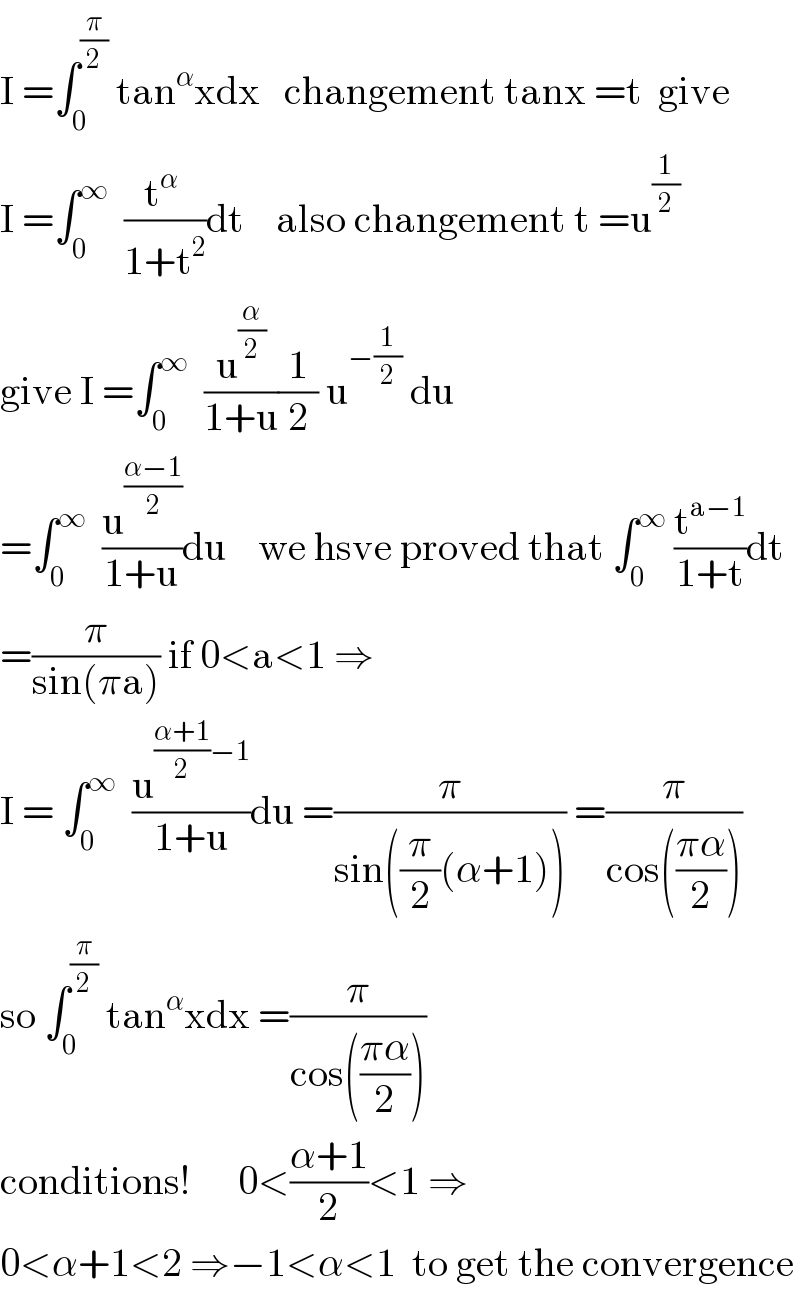
Commented by M±th+et+s last updated on 11/Jun/20

Commented by mathmax by abdo last updated on 11/Jun/20

| ||
Question and Answers Forum | ||
Question Number 97928 by M±th+et+s last updated on 10/Jun/20 | ||
 | ||
Answered by abdomathmax last updated on 10/Jun/20 | ||
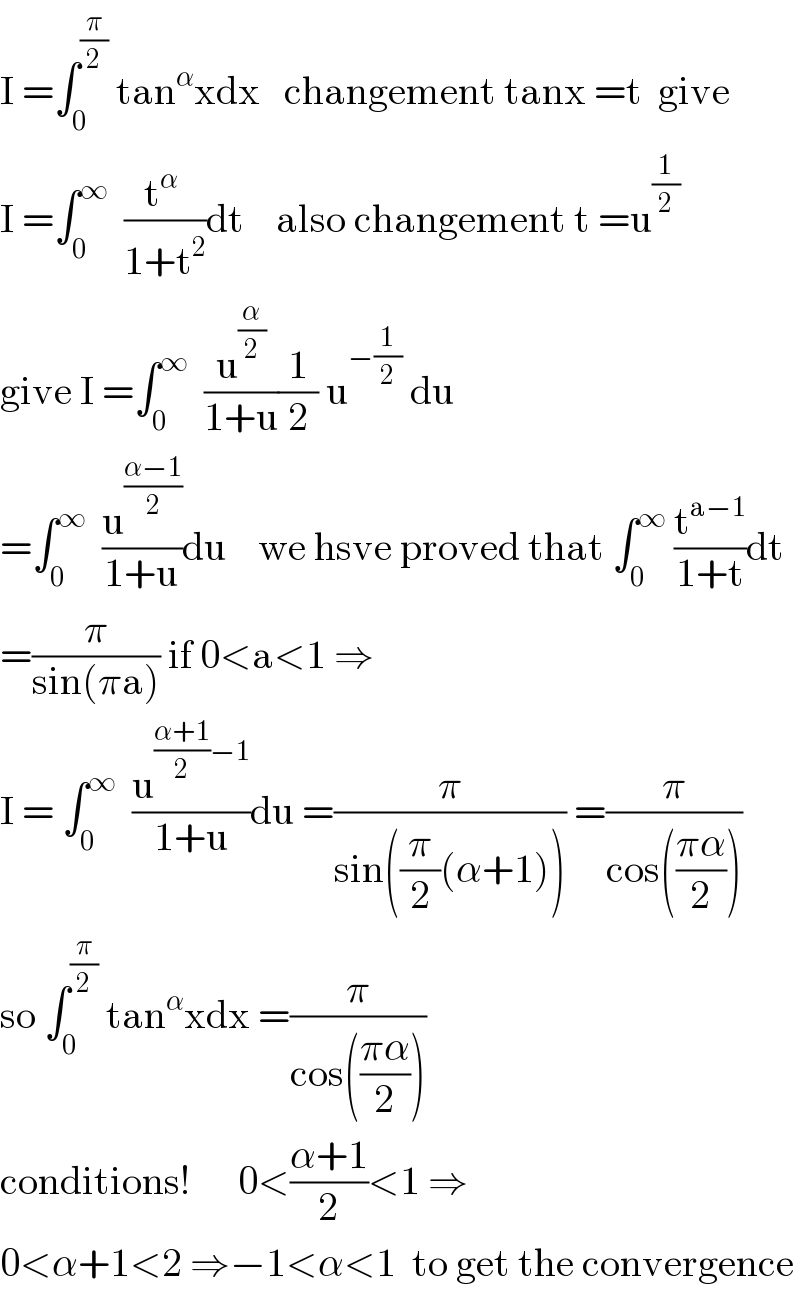 | ||
| ||
Commented by M±th+et+s last updated on 11/Jun/20 | ||
 | ||
Commented by mathmax by abdo last updated on 11/Jun/20 | ||
 | ||