
Question and Answers Forum
Question Number 97956 by M±th+et+s last updated on 10/Jun/20
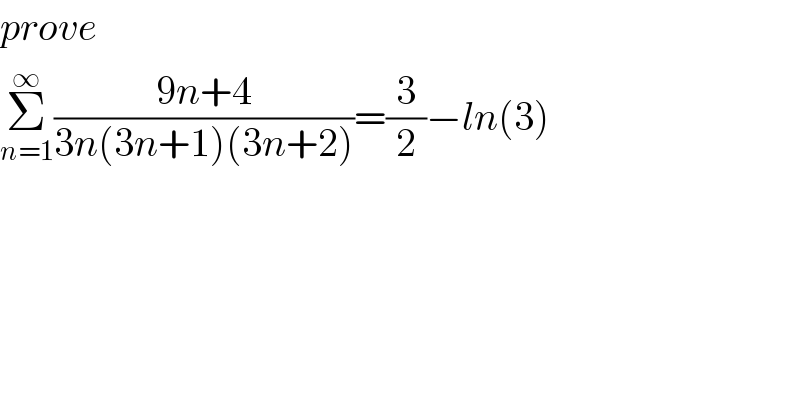
Answered by maths mind last updated on 13/Jun/20
![=Σ_(n≥1) ((4(3n+1)−3n)/(3n(3n+1)(3n+2))) =4Σ(1/(3n(3n+2)))−Σ(1/((3n+1)(3n+2))) =(4/9)Σ_(n≥1) (1/(n(n+(2/3))))−(1/9)Σ_(n≥1) (1/((n+(1/3))(n+(2/3)))) =(4/9)Σ_(n≥0) (1/((n+1)(n+(5/3))))−(1/9)Σ_(n≥0) (1/((n+(4/3))(n+(5/3)))) =(4/9).((Ψ((5/3))−Ψ(1))/((5/3)−1))−(1/9).((Ψ((5/3))−Ψ((4/3)))/((5/3)−(4/3))) S=(2/3)(Ψ((5/3))−Ψ(1))−(1/3)(Ψ((5/3))−Ψ((4/3))) (1/3)(Ψ((5/3))+Ψ((4/3)))−(2/3)Ψ(1) Ψ((p/q))=−γ−ln(2q)−(π/2)cot(((pπ)/q))+2Σ_(k=0) ^([((q−1)/2)]) cos(((2pπk)/q))ln(sin(((pkπ)/q))) Ψ((5/3))=(3/2)+Ψ((2/3)),Ψ((4/3))=3+Ψ((1/3)) Ψ((2/3))=−γ−ln(6)−(π/2)cot(((2π)/3))+2cos(((4π)/3))ln(sin(((2π)/3))) =−γ−ln(6)+(π/(2(√3)))−ln(((√3)/2)) Ψ((1/3))=−γ−ln(6)−(π/(2(√3)))−ln(((√3)/2)) S=(1/3)(−2γ−ln(27)+(3/2)+3)−(2/3)(−γ) S=ln((1/3))+(3/2)=(3/2)−ln(3)](Q98437.png)
Commented by M±th+et+s last updated on 13/Jun/20

| ||
Question and Answers Forum | ||
Question Number 97956 by M±th+et+s last updated on 10/Jun/20 | ||
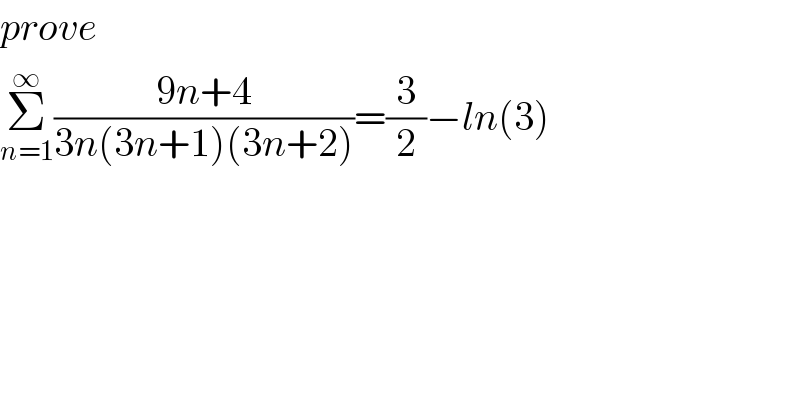 | ||
Answered by maths mind last updated on 13/Jun/20 | ||
![=Σ_(n≥1) ((4(3n+1)−3n)/(3n(3n+1)(3n+2))) =4Σ(1/(3n(3n+2)))−Σ(1/((3n+1)(3n+2))) =(4/9)Σ_(n≥1) (1/(n(n+(2/3))))−(1/9)Σ_(n≥1) (1/((n+(1/3))(n+(2/3)))) =(4/9)Σ_(n≥0) (1/((n+1)(n+(5/3))))−(1/9)Σ_(n≥0) (1/((n+(4/3))(n+(5/3)))) =(4/9).((Ψ((5/3))−Ψ(1))/((5/3)−1))−(1/9).((Ψ((5/3))−Ψ((4/3)))/((5/3)−(4/3))) S=(2/3)(Ψ((5/3))−Ψ(1))−(1/3)(Ψ((5/3))−Ψ((4/3))) (1/3)(Ψ((5/3))+Ψ((4/3)))−(2/3)Ψ(1) Ψ((p/q))=−γ−ln(2q)−(π/2)cot(((pπ)/q))+2Σ_(k=0) ^([((q−1)/2)]) cos(((2pπk)/q))ln(sin(((pkπ)/q))) Ψ((5/3))=(3/2)+Ψ((2/3)),Ψ((4/3))=3+Ψ((1/3)) Ψ((2/3))=−γ−ln(6)−(π/2)cot(((2π)/3))+2cos(((4π)/3))ln(sin(((2π)/3))) =−γ−ln(6)+(π/(2(√3)))−ln(((√3)/2)) Ψ((1/3))=−γ−ln(6)−(π/(2(√3)))−ln(((√3)/2)) S=(1/3)(−2γ−ln(27)+(3/2)+3)−(2/3)(−γ) S=ln((1/3))+(3/2)=(3/2)−ln(3)](Q98437.png) | ||
| ||
Commented by M±th+et+s last updated on 13/Jun/20 | ||
 | ||