
Question and Answers Forum
Question Number 98280 by I want to learn more last updated on 12/Jun/20
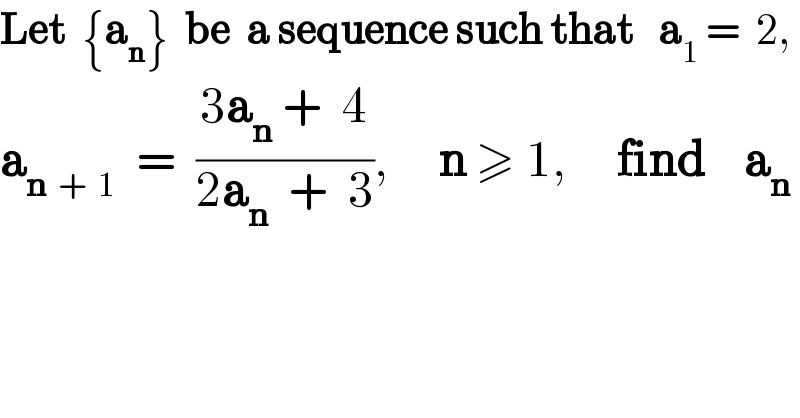
Answered by mr W last updated on 12/Jun/20
![a_(n+1) =((3a_n +4)/(2a_n +3)) a_(n+1) +A=((3a_n +4)/(2a_n +3))+A=(((3+2A)a_n +(4+3A))/(2a_n +3)) a_(n+1) +B=((3a_n +4)/(2a_n +3))+B=(((3+2B)a_n +(4+3B))/(2a_n +3)) ((a_(n+1) +A)/(a_(n+1) +B))=((3+2A)/(3+2B))×((a_n +((4+3A)/(3+2A)))/(a_n +((4+3B)/(3+2B)))) set A=((4+3A)/(3+2A)) ⇒A^2 =2 ⇒A=(√2) ⇒B=−(√2) ((3+2A)/(3+2B))=((3+2(√2))/(3−2(√2)))=(3+2(√2))^2 with b_n =((a_n +(√2))/(a_n −(√2))) b_(n+1) =(3+2(√2))^2 b_n ← G.P. ⇒b_n =b_1 (3+2(√2))^(2(n−1)) b_1 =((2+(√2))/(2−(√2)))=3+2(√2) ⇒b_n =(3+2(√2))^(2n−1) a_n =(((√2)(b_n +1))/(b_n −1))=(√2)(1+(2/(b_n −1))) ⇒a_n =(√2)[1+(2/((3+2(√2))^(2n−1) −1))] we get: a_1 =2 a_2 =((10)/7) a_3 =((58)/(41)) a_4 =((338)/(239))](Q98295.png)
Commented by I want to learn more last updated on 12/Jun/20
| ||
Question and Answers Forum | ||
Question Number 98280 by I want to learn more last updated on 12/Jun/20 | ||
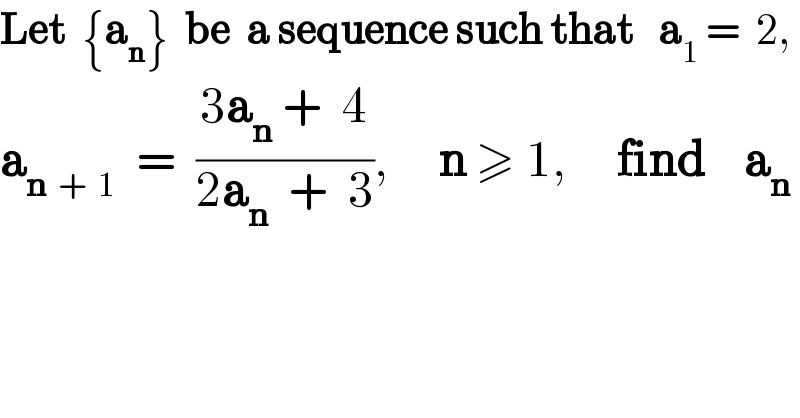 | ||
Answered by mr W last updated on 12/Jun/20 | ||
![a_(n+1) =((3a_n +4)/(2a_n +3)) a_(n+1) +A=((3a_n +4)/(2a_n +3))+A=(((3+2A)a_n +(4+3A))/(2a_n +3)) a_(n+1) +B=((3a_n +4)/(2a_n +3))+B=(((3+2B)a_n +(4+3B))/(2a_n +3)) ((a_(n+1) +A)/(a_(n+1) +B))=((3+2A)/(3+2B))×((a_n +((4+3A)/(3+2A)))/(a_n +((4+3B)/(3+2B)))) set A=((4+3A)/(3+2A)) ⇒A^2 =2 ⇒A=(√2) ⇒B=−(√2) ((3+2A)/(3+2B))=((3+2(√2))/(3−2(√2)))=(3+2(√2))^2 with b_n =((a_n +(√2))/(a_n −(√2))) b_(n+1) =(3+2(√2))^2 b_n ← G.P. ⇒b_n =b_1 (3+2(√2))^(2(n−1)) b_1 =((2+(√2))/(2−(√2)))=3+2(√2) ⇒b_n =(3+2(√2))^(2n−1) a_n =(((√2)(b_n +1))/(b_n −1))=(√2)(1+(2/(b_n −1))) ⇒a_n =(√2)[1+(2/((3+2(√2))^(2n−1) −1))] we get: a_1 =2 a_2 =((10)/7) a_3 =((58)/(41)) a_4 =((338)/(239))](Q98295.png) | ||
| ||
Commented by I want to learn more last updated on 12/Jun/20 | ||
 | ||