
Question and Answers Forum
Question Number 99496 by ~blr237~ last updated on 21/Jun/20

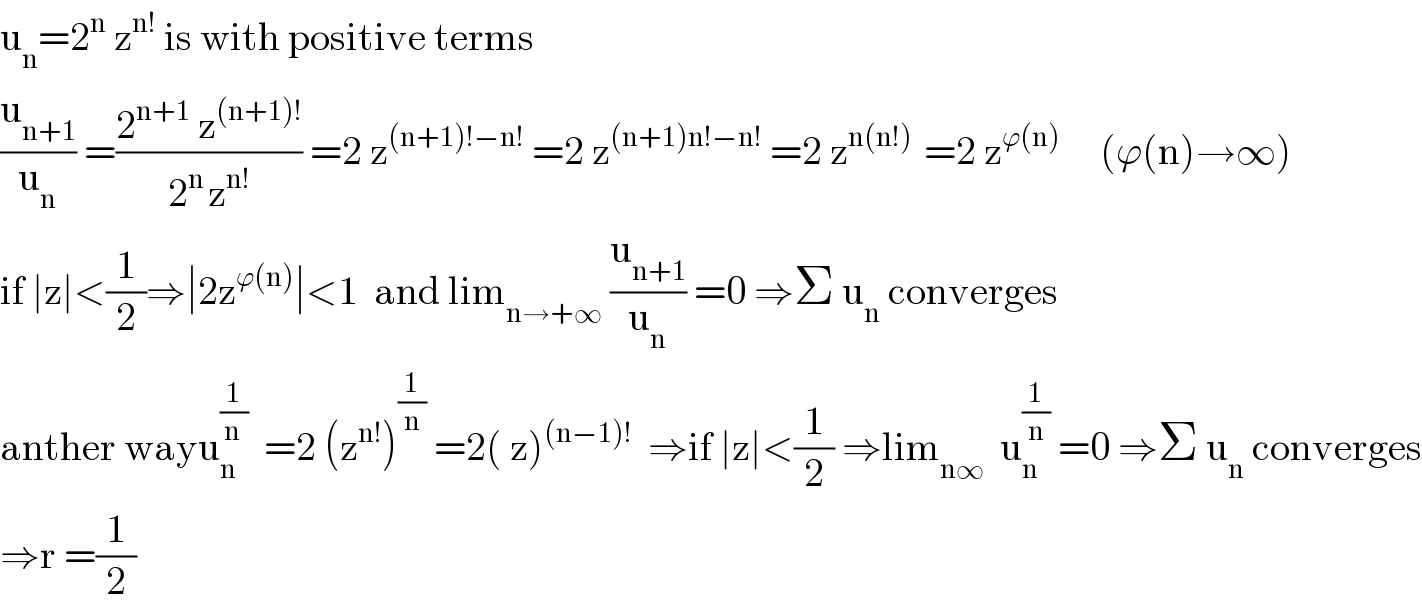
Answered by mathmax by abdo last updated on 21/Jun/20
Commented by maths mind last updated on 21/Jun/20
![sir i think R=1 Σ2^n ((2/3))^(n!) ∃a<1 &∃N∈N ∣ ∀n≥N 2^n ((2/3))^(n!) <a^n ⇔n!ln((2/3))<nln((a/2)) ⇔(n−1)!≥((ln((a/2)))/(ln((2/3)))),a=(1/4) (n−1)!≥((ln(8))/(ln((3/2)))) hase solution since (n−1)!→∞ Σ2^n ((2/3))^(n!) =Σ_(n=0) ^(N−1) 2^n ((2/3))^(n!) +Σ_(k≥N) 2^k ((2/3))^k first finit sum 2nd 2^k ((2/3))^k <((1/4))^n by comparaison Cv let a<1 ∃N_a ∈N∣∀n≥N 2^n (aaaa)^(n!) <((a/2))^n ⇔n!ln(a)<nln((a/4))⇔(n−1)!>((ln((a/4)))/(ln(a))) hase always solution for a∈]0,1[](Q99564.png)
Commented by mathmax by abdo last updated on 22/Jun/20

| ||
Question and Answers Forum | ||
Question Number 99496 by ~blr237~ last updated on 21/Jun/20 | ||
 | ||
Answered by mathmax by abdo last updated on 21/Jun/20 | ||
 | ||
| ||
Commented by maths mind last updated on 21/Jun/20 | ||
![sir i think R=1 Σ2^n ((2/3))^(n!) ∃a<1 &∃N∈N ∣ ∀n≥N 2^n ((2/3))^(n!) <a^n ⇔n!ln((2/3))<nln((a/2)) ⇔(n−1)!≥((ln((a/2)))/(ln((2/3)))),a=(1/4) (n−1)!≥((ln(8))/(ln((3/2)))) hase solution since (n−1)!→∞ Σ2^n ((2/3))^(n!) =Σ_(n=0) ^(N−1) 2^n ((2/3))^(n!) +Σ_(k≥N) 2^k ((2/3))^k first finit sum 2nd 2^k ((2/3))^k <((1/4))^n by comparaison Cv let a<1 ∃N_a ∈N∣∀n≥N 2^n (aaaa)^(n!) <((a/2))^n ⇔n!ln(a)<nln((a/4))⇔(n−1)!>((ln((a/4)))/(ln(a))) hase always solution for a∈]0,1[](Q99564.png) | ||
Commented by mathmax by abdo last updated on 22/Jun/20 | ||
 | ||