A particle of mass m moving with speed u collides perfectly inelastically with a sphere of radius R and same mass, at rest, at an impact parameter d. Find (a) Angle between their final velocities (b) Magnitude of their final velocities


Question and Answers Forum

All Questions Topic List
Others Questions
Previous in All Question Next in All Question
Previous in Others Next in Others
Question Number 25091 by Tinkutara last updated on 03/Dec/17

$A p a r t i c l e o f m a s s m m o v i n g w i t h$ $s p e e d u c o l l i d e s p e r f e c t l y i n e l a s t i c a l l y$ $w i t h a s p h e r e o f r a d i u s R a n d s a m e$ $m a s s, a t r e s t, a t a n i m p a c t p a r a m e t e r$ $d . F i n d$ $(a) A n g l e b e t w e e n t h e i r f i n a l v e l o c i t i e s$ $(b) M a g n i t u d e o f t h e i r f i n a l$ $v e l o c i t i e s$
	Answered by ajfour last updated on 03/Dec/17

	Commented by ajfour last updated on 04/Dec/17

	$c o n s e r v i n g l i n e a r m o m e n t u m$ $m u = 2 m v + m ω R \sin θ$ $a n d \sin θ = d / R, s o$ $2 v + ω d = u . . . (i)$ $c o n s e r v i n g a n g u l a r m o m e n t u m$ $a b o u t g r o u n d p o i n t G :$ $m u (R + d) = m v R + \frac{2}{5} {m R}^{2} ω +$ $m (v + ω R \sin θ) (R + d) + m ω {(R \cos θ)}^{2}$ $. . . . (i i)$ $a n d a s {(R \cos θ)}^{2} = (R + d) (R - d),$ $a l s o R \sin θ = d$ $\Rightarrow u (R + d) = v R + \frac{2}{5} ω R^{2} +$ $(v + ω d) (R + d) + ω (R^{2} - d^{2})$ $f r o m (i) : v + ω d = u - v, s o$ $v d = ω (\frac{7}{5} R^{2} - d^{2})$ $a n d f r o m (i) : 2 v + ω d = u$ $\Rightarrow 2 ω (\frac{7}{5} R^{2} - d^{2}) + ω d^{2} = u d$ $ω = \frac{u d}{(\frac{14}{5} R^{2} + d^{2})}; v = \frac{u (\frac{7}{5} R^{2} - d^{2})}{(\frac{14}{5} R^{2} + d^{2})}$ $\tan ϕ = \frac{ω R \cos θ}{v + ω d} = \frac{d \sqrt{R^{2} - d^{2}}}{(v d / ω) + d^{2}}$ $\tan ϕ = \frac{5 d \sqrt{R^{2} - d^{2}}}{7 R^{2}} .$ $V = \frac{ω R \cos θ}{\sin ϕ} = ω [\frac{\sqrt{R^{2} - d^{2}}}{\sin ϕ}] .$
	Commented by ajfour last updated on 03/Dec/17

	$∠ b e t w e e n t h e i r f i n a l v e l o c i t i e s$ $i s ϕ .$ $f i n a l v e l o c i t y o f s p h e r e v .$ $F i n a l v e l o c i t y o f p a r t i c l e i s V .$
	Commented by Tinkutara last updated on 05/Dec/17

	$A n s w e r i s :$ $v_{1} = \frac{u d}{R}, v_{2} = - v_{3} = \frac{u \sqrt{R^{2} - d^{2}}}{2 R}$
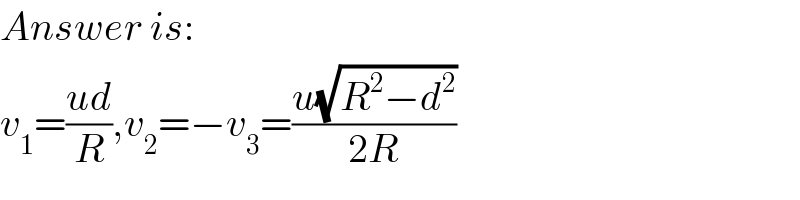
	Commented by ajfour last updated on 05/Dec/17

	$w h i c h a n s w e r i s c o r r e c t f o r$ $d = 0, a n d e v e n d = R ? P l e a s e$ $c h e c k o r i t h i n k i h a v e$ $m i s u n d e r s t o o d t h e q u e s t i o n .$ $m r W s i r c a n h e l p i f h e f i n d s$ $t i m e a n d n o t i c e s m y r e q u e s t .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum