
Question and Answers Forum
AlgebraQuestion and Answers: Page 330






Pg 325 Pg 326 Pg 327 Pg 328 Pg 329 Pg 330 Pg 331 Pg 332 Pg 333 Pg 334
|
Question and Answers Forum |
AlgebraQuestion and Answers: Page 330 |
| find the polynial p wich verify p(x)−p^′ (x)=x^n then calculate ∫_0 ^1 p(x)dx. |
| find tbe value of Π_(k=1) ^n sin(((kπ)/(n+1))). |
| let a∈]0,π[ and A(x)= x^(2n) −2cos(na)x^n +1 1)factorize inside C[x] A(x) 2) factorize inside R[x] A(x). |
| let give p(x)=(x+j)^n −(x−j)^n with j=e^(i((2π)/3)) 1) find roots of p(x) 2) factorize inside C[ x] p(x) 3)factorize inside R[x] p(x). |
| prove that x^2 divide (x+1)^n_ −nx−1 .nintegr. |
| if (xcosθ +sint)^n =Q(x^2 +1) +R find tbe polynomialR |
| find all polynomial p(x) wich verify ∀k∈Z ∫_k ^(k+1) p(x)dx=k+1. |
| let p(x)= x^n +a_(n−1) x^(n−1) +.... a_1 x +a_o if ξ is roots of p(x) prove that ∣ξ∣ ≤ 1+max_(0≤i≤n−1) ∣a_i ∣ |
| simplify p(x)= (1+x^2 )(1+x^4 )....(1+x^(2n) ) with n fromN then find the roots of p(x). |
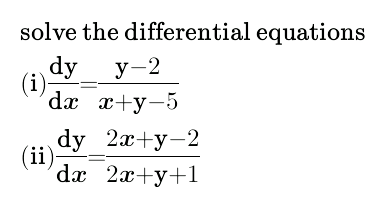
|
| Find sum of S= (2/3) + (4/3^2 ) + (6/3^3 ) + (8/3^4 ) +......+∞ ? |
| The maximum area of the triangle whose sides a,b and c satisfy 0≤a≤1 , 1≤b≤2 , 2≤c≤3 is : A) 1 B) 2 C) 1.5 D) 0.5 ? |
| Find the principal value of z=(1−i)^(1+i) .Hence find the modulus of the result. |
| Find the pricipal value of z=(1−i)^i |

|

|
| Let p and q are the roots of x^2 − 2mx − 5n = 0 and m and n are the roots of x^2 − 2px − 5q = 0 If p ≠ q ≠ m ≠ n, then the value of p + q + m + n is ... |

|
| Find all set of ordered triple/s (x,y,z), x,y,z∈ℜ, such that x−y=1−z 3(x^2 −y^2 )=5(1−z^2 ) 7(x^3 −y^3 )=19(1−z^3 ). Please show your solution. |

|

|

|
| Find the remainder when x^(203) −1 is divided by x^4 −1. |
| let Δ={(x,y)∈N^2 /x+y=n , n∈N} find cardΔ 2) let A= {(x,y)∈N^2 / x+2y=n} find card A. |
| prove that C_n ^o C_n ^p +C_n ^1 C_(n−1) ^(p−1) +...C_n ^p C_(n−p) ^0 =2^p C_n ^p . |
| solve in N^2 9y^2 −(x+1)^2 =32 . |
Pg 325 Pg 326 Pg 327 Pg 328 Pg 329 Pg 330 Pg 331 Pg 332 Pg 333 Pg 334 |