
Question and Answers Forum
AlgebraQuestion and Answers: Page 342
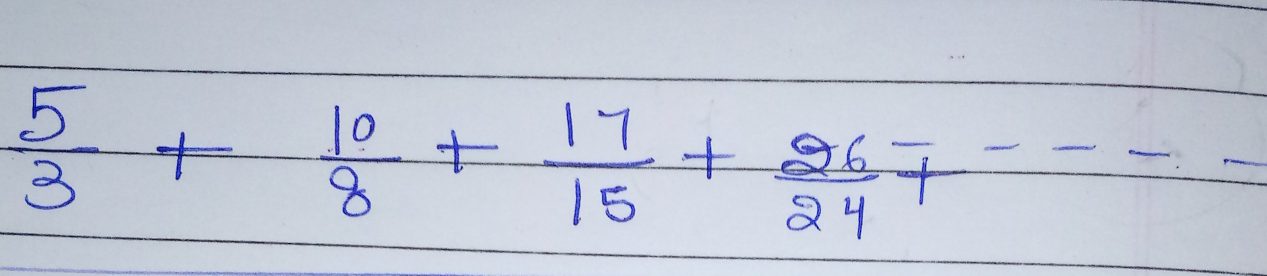


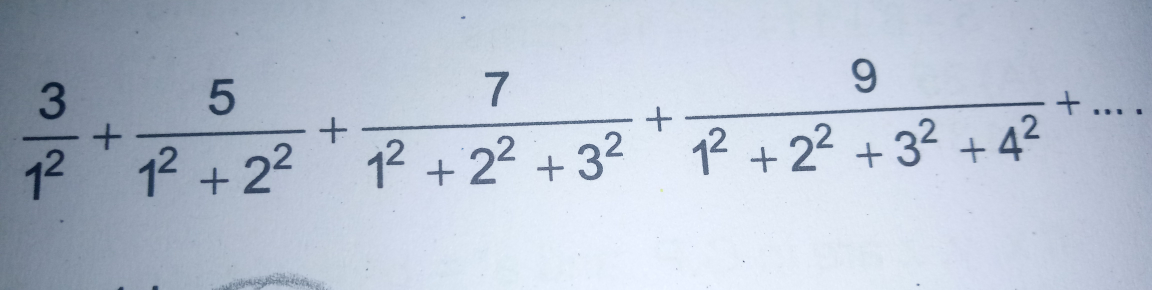

Pg 337 Pg 338 Pg 339 Pg 340 Pg 341 Pg 342 Pg 343 Pg 344 Pg 345 Pg 346
|
Question and Answers Forum |
AlgebraQuestion and Answers: Page 342 |
| Common solution. (d/dy)(u_x +u)+2x^2 y(u_x +u)=0. |
| Prove that ΣΣ_(0≤i<j≤n) ((1/(^n C_i )) + (1/(^n C_j ))) = Σ_(r=0) ^(n−1) ((n − r)/(^n C_r )) + Σ_(r=1) ^n (r/(^n C_r )) |
| Prove that (1/(m!))C_0 +(n/((m+1)!))C_1 +((n(n−1))/((m+2)!))C_2 +...+((n(n−1)...2.1)/((m+n)!))C_n = (((m+n+1)(m+n+2)...(m+2n))/((m+n)!)). |
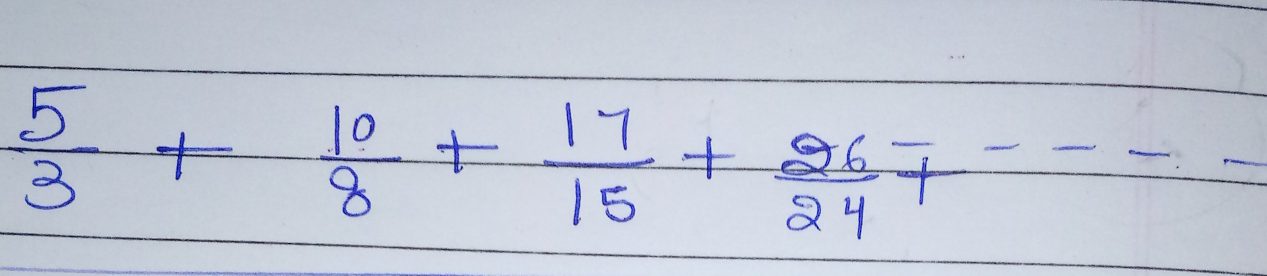
|

|
| If (1 + x)^n = C_0 + C_1 x + C_2 x^2 + C_3 x^3 + ... + C_n x^n , then prove that ΣΣ_(0≤i<j≤n) ((i/(^n C_i )) + (j/(^n C_j ))) = (n^2 /2)(Σ_(r=0) ^n (1/(^n C_r ))). |
| Is it possible to find how many real roots exist in the equation x^4 + ∣x∣ = 3 without find all the value of x? |
| Solve: 3^(x ) = ((27)/x) + 18 |
| Let n be a positive integer and p_1 , p_2 , ..., p_n be n prime numbers all larger than 5 such that 6 divides p_1 ^2 + p_2 ^2 + ... + p_n ^2 . Prove that 6 divides n. |

|
| solve: sin(x)=2, x∈C |
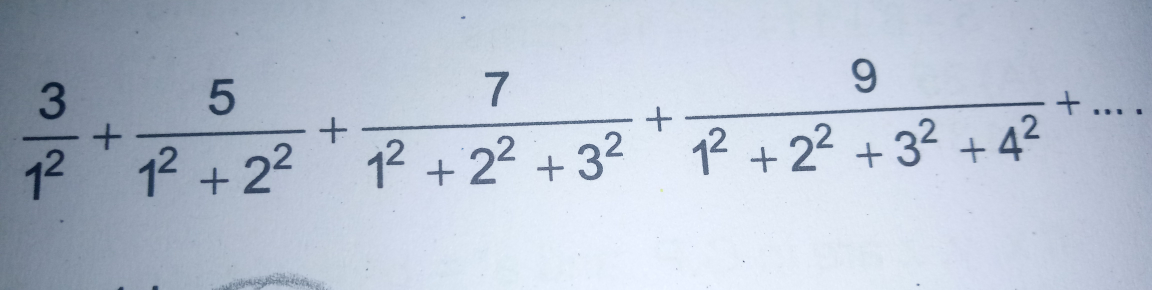
|
| If (1 + x)^n = C_0 + C_1 x + C_2 x^2 + C_3 x^3 + ... + C_n x^n , Prove that ΣΣ_(0≤i<j≤n) (i + j)C_i C_j = n(2^(2n−1) − (1/2)^(2n) C_n ) |

|
| If (1 + x)^n = C_0 + C_1 x + C_2 x^2 + C_3 x^3 + ... + C_n x^n , prove that (2^2 /(1.2))C_0 + (2^3 /(2.3))C_1 + (2^4 /(3.4))C_2 + ... + (2^(n+2) /((n + 1)(n + 2)))C_n = ((3^(n+2) − 2n − 5)/((n + 1)(n + 2))) |
| With usual notation, show that (C_0 /x) − (C_1 /(x+1)) + (C_2 /(x+2)) − .... + (−1)^n (C_n /(x+n))= ((n!)/(x(x + 1)(x + 2)....(x + n))) |
| In the binomial expasion of (a − b)^5 , the sum of 2^(nd) and 3^(rd) term is zero, then (a/b) is |
| If α = (5/(2!3)) + ((5.7)/(3!3^2 )) + ((5.7.9)/(4!3^3 )) ,... then find the value of α^2 + 4α. |
| Find the coefficient of x in the expansion of [(√(1 + x^2 )) − x]^(−1) in ascending power of x when ∣x∣ < 1. |
| If (a + bx)^(−2) = (1/4) − 3x + ..., then (a, b) = |
| The coefficient of x^r in the expansion of (1 − 2x)^(−1/2) is (1) (((2r)!)/((r!)^2 )) (2) (((2r)!)/(2^r (r!)^2 )) (3) (((2r)!)/((r!)^2 2^(2r) )) (4) (((2r)!)/(2^r (r + 1)!(r − 1)!)) |
| Let R = (5(√5) + 11)^(2n+1) and f = R − [R], then prove that Rf = 4^(2n+1) . |
| If x^x ∙y^y ∙z^z = x^y ∙y^z ∙z^x = x^z ∙y^x ∙z^y such that x, y and z are positive integers greater than 1, then which of the following cannot be true for any of the possible value of x, y and z? (1) xyz = 27 (2) xyz = 1728 (3) x + y + z = 32 (4) x + y + z = 12 |
| If a_r is the coefficient of x^r in the expansion (1 + x + x^2 )^n , then a_1 − 2a_2 + 3a_3 − ....... 2na_(2n) = |
| Solve for real x: (1/([x])) + (1/([2x])) = (x) + (1/3), where [x] is the greatest integer less than or equal to x and (x) = x − [x], [e.g. [3.4] = 3 and (3.4) = 0.4]. |
| { (((√(x^2 −4xy))+(√(y^2 +2xy+9))=10)),((x−y=7)) :} How many real roots of the equtions system? |
Pg 337 Pg 338 Pg 339 Pg 340 Pg 341 Pg 342 Pg 343 Pg 344 Pg 345 Pg 346 |