
Question and Answers Forum
AlgebraQuestion and Answers: Page 96




Pg 91 Pg 92 Pg 93 Pg 94 Pg 95 Pg 96 Pg 97 Pg 98 Pg 99 Pg 100
|
Question and Answers Forum |
AlgebraQuestion and Answers: Page 96 |
| solve (√(25−x^3 ))+(√(144−x^3 ))=13 |
| Calculas: ((1^2 + 2^2 + 3^2 + ... + 16^2 − 16)/(1∙3 + 2∙4 + 3∙5 + ... + 15∙17)) |
| if x^2 +xy+y^2 =5, find the minimum and maximum of x^2 −xy+y^2 . |
| Resoudre l equation (a/(cos^3 x))+(b/(cos x))=(c/(sin x)) a,b,c reels x#{k(π/2) k∈Z} |
| x, y are different positive integers with (1/x)+(1/y)=(1/5). find x+y=? |

|
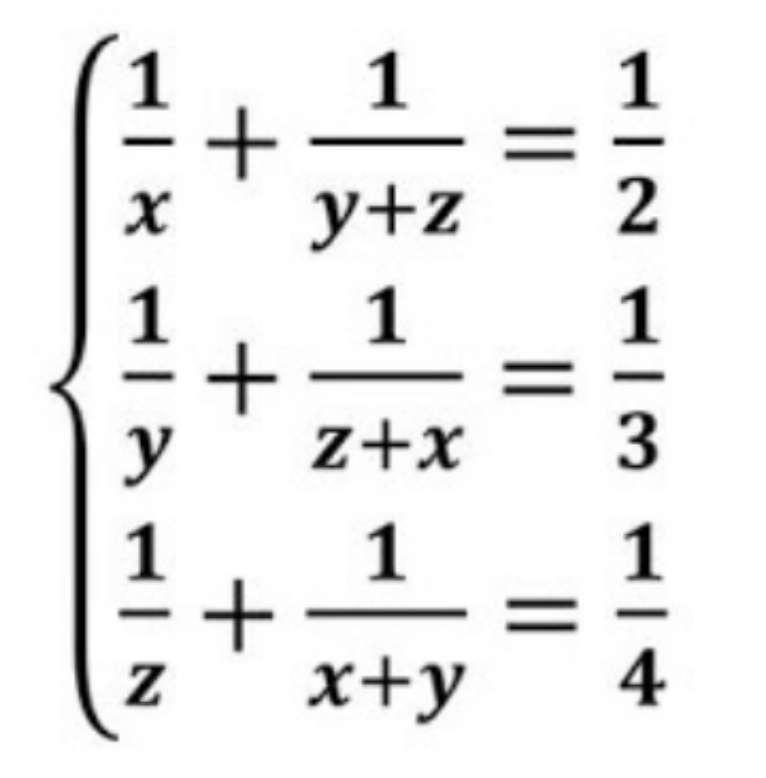
|
| solve for R a+bcd=100 b+cda=100 c+dab=100 d+abc=100 |
| if (√(x+(√(x+(√(x+(√(x+(√x)))))))))=2022 find [x]=? |
| x^3 −(3/4)x+(1/8)=0 x=? |
| { ((a^3 =3ab^2 +11)),((b^3 =3a^2 b+2)) :} ; a,b ∈R ⇒a^2 +b^2 =? |
| f(x)+3f((1/x))=(1/x) f(x)=? |

|
| Find a, b, c ∈ N ; 2^( a) + 4^( b) + 8^( c) = 328 |

|
| a_(n+2) −5a_(n+1) +6a_n =3n+5^n a_1 =1, a_2 =0 find a_n |
| In △ABC the following relationship holds: 6r Σ_(cyc) (r_a /(s + n_a )) + Σ_(cyc) n_a ≥ 3s |
| x^3 +y^3 =z^2 x^3 +z^3 =y^2 y^3 +z^3 =x^2 obviously x=y=z=0∨(1/2) trying to totally solve it let y=px∧z=qx (p^3 +1)x^3 =q^2 x^2 (q^3 +1)x^3 =p^2 x^2 (p^3 +q^3 )x^3 =x^2 ⇒ x=0 ⇒ y=0∧z=0 ★ x=(q^2 /(p^3 +1)) x=(p^2 /(q^3 +1)) x=(1/(p^3 +q^3 )) ⇒ (p^2 /(q^3 +1))=(q^2 /(p^3 +1)) (p^2 /(q^3 +1))=(1/(p^3 +q^3 )) ========== p^5 +p^2 −q^5 −q^2 =0 p^5 +p^2 q^3 −q^3 −1=0 subtracting both p^2 (q^3 −1)+q^5 −q^3 +q^2 −1=0 (q−1)(p^2 (q^2 +q+1)+q^4 +q^3 +q+1)=0 ⇒ q=1 ⇒ p^5 +p^2 −2=0 (p−1)(p^4 +p^3 +2p+2)=0 ⇒ p=1 ⇒ x=y=z=(1/2) ★ p^4 +p^3 +2p+2=0 no useable exact solutions p≈−.975564±.528237i ⇒ x≈.33635∓.515329i ∧ y≈−.0559113±.680406i ∧ z=x ★ p≈.475564±1.18273i ⇒ x≈−.586346±.562464i ∧ y≈−.944089∓.426001i ∧ z=x ★ p^2 (q^2 +q+1)+q^4 +q^3 +q+1=0 p^2 =−(((q+1)^2 (q^2 −q+1))/(q^2 +q+1)) we can be sure that (q≠−1⇒p=0) ⇒ p^2 <0 ⇒ p=±(√((q^2 −q+1)/(q^2 +q+1)))(q+1)i ...I′ll continue later |

|
| If x^3 +(1/x^3 )=1, prove that x^5 +(1/x^5 )=−(x^4 +(1/x^4 )) |
| { ((p^3 +q^3 =r^2 )),((p^3 +r^3 =q^2 )),((q^3 +r^3 =p^2 )) :} ⇒20pqr =? |
| Solve the equation: 2^x + 3^x − 4^x + 6^x − 9^x = 1 |
| x^3 +(1/x^3 )=1 (((x^5 +(1/x^5 ))^3 −1)/(x^5 +(1/x^5 )))=? Q#176387 reposted for a new answer. |
| 4^(x^2 −2x+2) −2^(x^2 −2x+3) +2=2^(x^2 −2x+2) x=? |
| ABCD−convex quadrilateral M∈Int(ABCD) , F−area , s−semiperimetr a , b , c−sides. Prove that: ((MA^4 )/b) + ((MB^4 )/c^4 ) + ((MC^4 )/d) + ((MD^4 )/a) ≥ ((2F^2 )/s) |
| x + (1/x) =ϕ (Golden ratio) then x^( 2000) +(( 1)/x^( 2000) ) = ? |
Pg 91 Pg 92 Pg 93 Pg 94 Pg 95 Pg 96 Pg 97 Pg 98 Pg 99 Pg 100 |