
Question and Answers Forum
AllQuestion and Answers: Page 1146
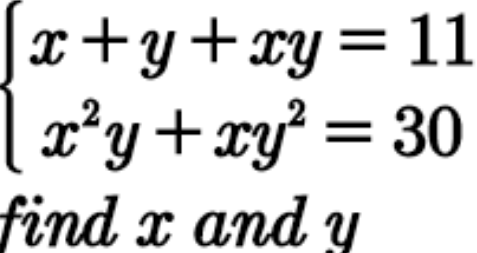

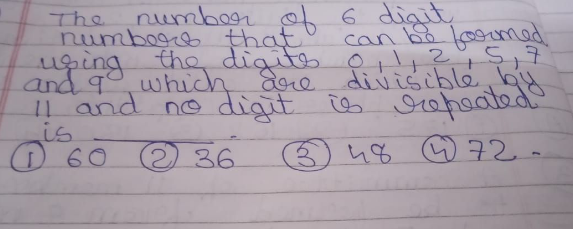



Pg 1141 Pg 1142 Pg 1143 Pg 1144 Pg 1145 Pg 1146 Pg 1147 Pg 1148 Pg 1149 Pg 1150
|
Question and Answers Forum |
AllQuestion and Answers: Page 1146 |
| Show that if a function is even and derivable then f′(x) is an odd function. |
| solve ∣x+(1/x)∣ > 2 |
| ∫_1 ^5 x(∫f(x)dx) dx = 24 ∫_1 ^3 (2−f(x))dx ? |
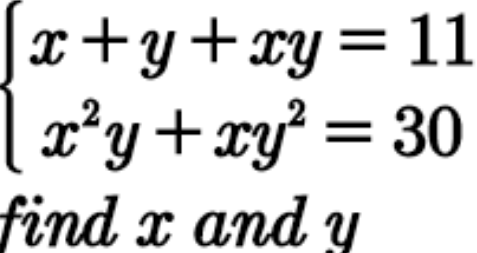
|

|
| 1z 1 |
| solve the differential equation L(d^2 q/dt^2 )+R(dq/dt)+(1/C)q=εcosωt which is in R.L.C circuit with forced oscillation where L is inductance R is resistance C is capacitanace q is charge ε is motion emf t is time |
| If sin^(−1) x_1 +sin^(−1) x_2 +...+sin^(−1) x_(2n) =nπ, then Σ^(2n) _(i, j=1_(i ≠ j) ) x_i x_j = |
| The value of cosec^2 (π/7)+cosec^2 ((2π)/7)+cosec^2 ((3π)/7) is |
| if f(x) = ((sin x)/((1 + x^2 +x^6 )^2 )) is odd, find ∫_(−3) ^3 f(x) dx |
| find ∫ (dx/(x^n (x+1)^m )) m and n integr |
| calculate ∫_2 ^(+∞) (dx/((x−1)^4 (x^2 +x+1)^2 )) |
| calculate ∫_1 ^(+∞) (dx/(x^3 (2x+1)^4 )) 1)without use of decomposition 2)by use of decomposition |
| ∫ln∣cot(x/2)∣dx |
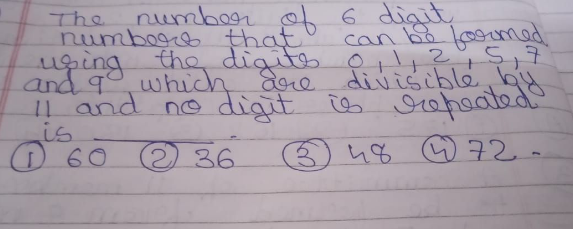
|
| ∫((ax^2 +bx+c)/((x−p)(x−q)(x−r)))dx |
| show that ∫((sin (x−θ))/(sin x))dx=xcos x−sin θlog sin x |

|

|
| ∫_(−π) ^π ∣cos^3 x∣dx |

|
| ∫((2x^3 dx)/(2x^2 −4x+3))=? |
| ∫x^2 (√(a^2 +x^2 ))dx=? |
| ((tanx×ctg2x)/(tan^2 x−1))=? |
| (1/(sin10))−((√3)/(cos10))=? |
| For 0<x<(π/(6 )), all the values of tan^2 (3x)cos^2 (x)−4tan (3x)sin (2x)+16sin^2 (x) lie in the interval (a). (0,((121)/(36))) (b).(1,((121)/9)) (c). (−1,0) (d). None of these. |
Pg 1141 Pg 1142 Pg 1143 Pg 1144 Pg 1145 Pg 1146 Pg 1147 Pg 1148 Pg 1149 Pg 1150 |