
Question and Answers Forum
AllQuestion and Answers: Page 1364
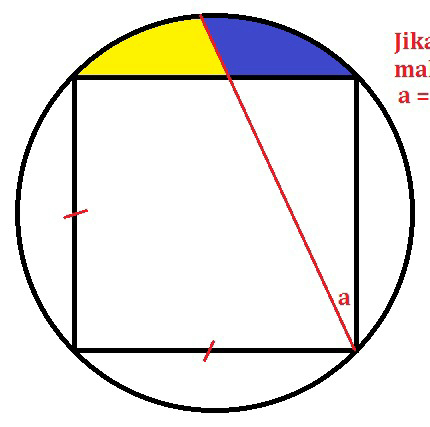







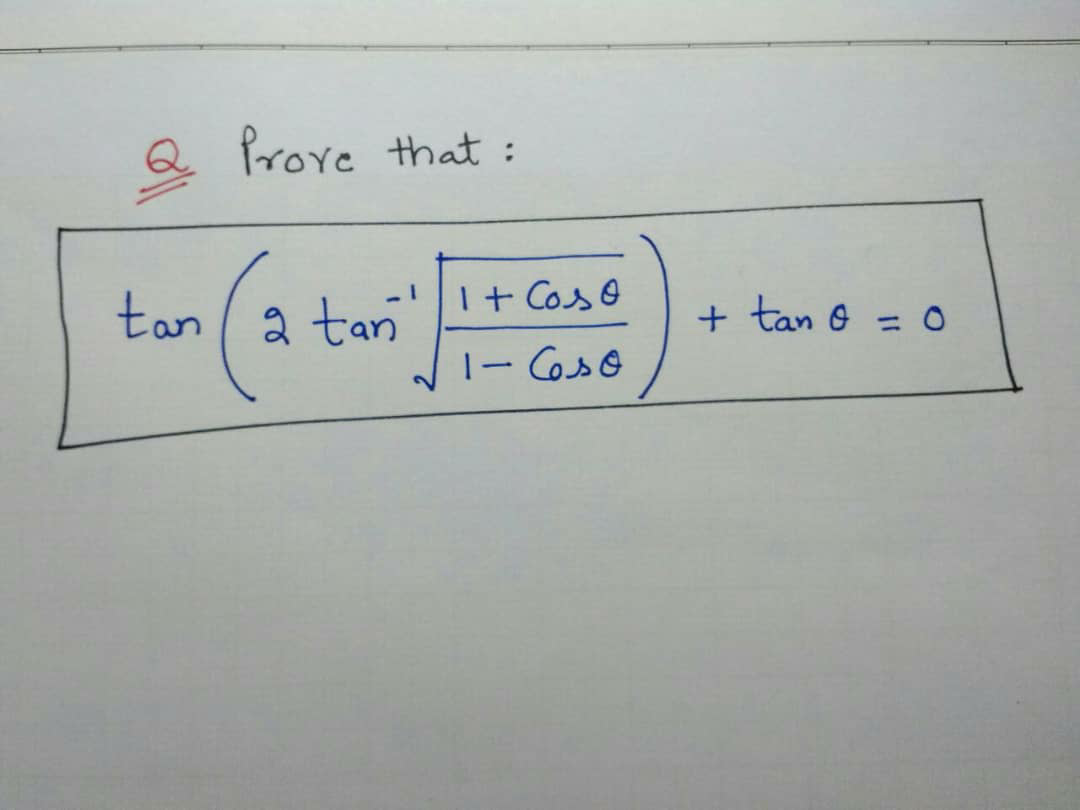

Pg 1359 Pg 1360 Pg 1361 Pg 1362 Pg 1363 Pg 1364 Pg 1365 Pg 1366 Pg 1367 Pg 1368
|
Question and Answers Forum |
AllQuestion and Answers: Page 1364 |
| My question is about the analogical axiams of the foundation geometry in mathematocs. As it Is a well knowen axum in geometry starts from the sefinition of a point which gives gives the path analogically to line, plane, and solids. Know my truoble comes at these axiumes areise from not ne being they are aziyma ^ but the analogu effect at giving the definatiom of the solid} 1−Apoimt is a dimenstin less. mathematixal abstruct. 2− a line is the collextom of points which has only one dimension. 3− a plane is the collection of lines which have onlu?two dimensions 3−a solid is the collwxripm of plans which has three dimensions. Now the first three definationa arsties are mathe are mathematical ideasor abstruct while the last mathematical abstruct is real. ow on earth a real object is formed from the collextion of unreal planes |
| sin 1^° = ? |
| I=∫_0 ^( 1) (√((c−x^2 )/(x(1−x^2 ))))dx (c >1) |

|
| find the value of ∫_0 ^∞ ((arctan(2x^2 ))/(x^2 +4))dx |
| let f(x) =∫_x^ ^(x^2 −x) arctan(e^(−x−t) )dt calculate f^′ (x) and f^′ (0). |
| ((sin 72°)/(sin 42°)) = p tan 12° = ? |

|
| cos (x−60)+cos (x−30)=sin x prove |

|
| hello i search som lectur about hypergeometric fonction2F_1 (a,b,c,x)=((Γ(c))/(Γ(a)Γ(b)))Σ_(n≥0) ((Γ(a+n)Γ(b+n))/(Γ(c+n)n!))x^n |

|

|

|

|
| Is it possible to find any value for a,b,c from below system of equetions? { ((sina+sinb=sinc)),((cosa+cosb=cosc)) :} |
| calculate ∫_0 ^(+∞) ((arctan(x^2 ))/(1+x^2 ))dx |
| There are a few problem reported. Notification: google discontinued Google Cloud Messaging so notifications are not working. Other Problems: There has been several new phone models and android version updates. And newer android version or phone models might have other issues. We are working on app updates for supporting these and also migrating to new messaging platform. We will address these problems as soon as we can. |

|
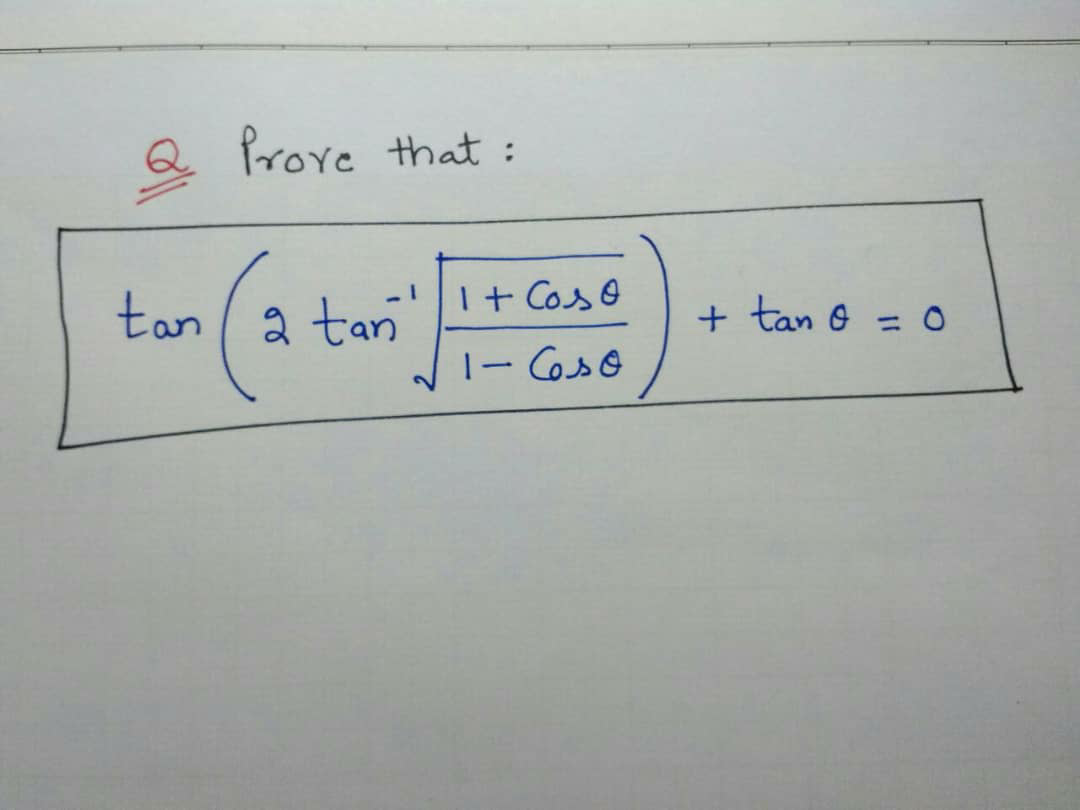
|

|
| (1/(x+1)) = y lim_(x+1 → ∞) x tan ((1/(2x+2))) |
| lim_(x,y→(0,0)) ((x^4 − x^2 y^2 + y^4 )/(x^2 + x^4 y^4 + y^2 )) |
| If sin x+sin^2 x=1, then value of cos^2 x+cos^4 x is |
| The value of ((1−tan^2 15°)/(1+tan^2 15°)) is |
| If 2x^2 +(2p−13)x+2=0 is exactly divisible by x−3, then the value of p is |
Pg 1359 Pg 1360 Pg 1361 Pg 1362 Pg 1363 Pg 1364 Pg 1365 Pg 1366 Pg 1367 Pg 1368 |