
Question and Answers Forum
AllQuestion and Answers: Page 1440




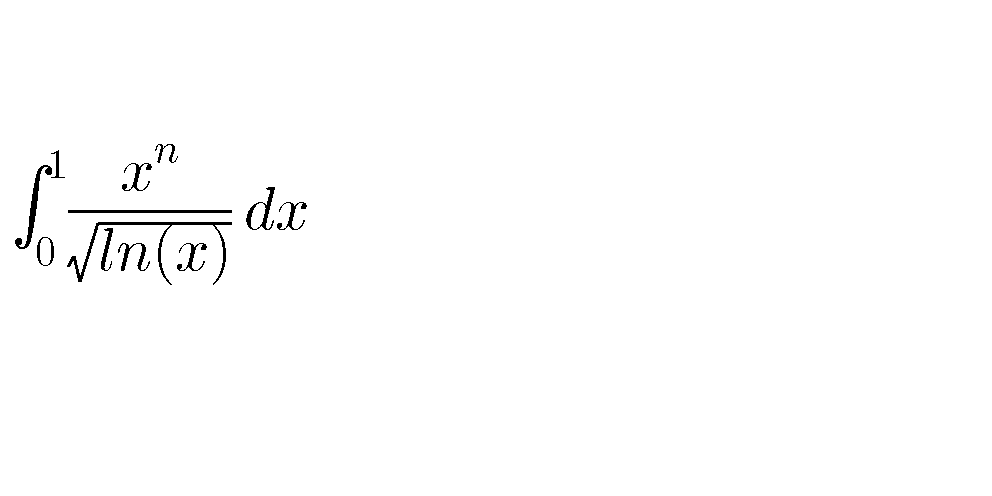
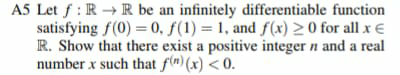



Pg 1435 Pg 1436 Pg 1437 Pg 1438 Pg 1439 Pg 1440 Pg 1441 Pg 1442 Pg 1443 Pg 1444
|
Question and Answers Forum |
AllQuestion and Answers: Page 1440 |
| Find all integer solution(s): 615+x^2 =2^y |
| let V be a vector space and let H and K be subspace of V. show that , H+K={x:x=h+k, where h∈H and k∈K} is a subspace of V. |
| consider the space Pn with H={f:f⊂Pn and ∫_0 ^1 f(x)∂x=0} . Show that H is a SUBSPACE of Pn. |
| consider the triple of real numbers (x,y,z) defined by the addittion (x,y,z)+(x′,y′,z′)=(x+x′,y+y′,z+z′) and scalar multiplication by 𝛂(x,y,z)=(0,0,0). Show that all axioms for a vector space are satisfied except axiom 8. |
| ∫_(0 ) ^1 ((3x^3 −x^2 +2x−4)/(√(x^2 −3x+2))) dx |
| If α = Cis(2π/7) and f(x) = A_0 + Σ_(n=1) ^(14) A_n x^n Then prove that Σ_(α=0) ^6 f(α^n x)= 7(A_0 +A_7 x^7 +A_(14) x^(14) ) where Cisθ = Cosθ + iSinθ |

|

|
| If D,E and F are midpoints of the sides BC,CA and AB respectively of the △ABC and O be any point.Prove that OA^→ + OB^→ +OC^→ =OD^→ +OE^→ +OF^→ |
| Find (dy/dx) y = sin^(−1) (((1−x^2 )/(1+x^2 ))), 0<x<1 |
| 2H_2 S+SO_2 =3S+H_2 O is this a disproportionation reaction? |

|

|
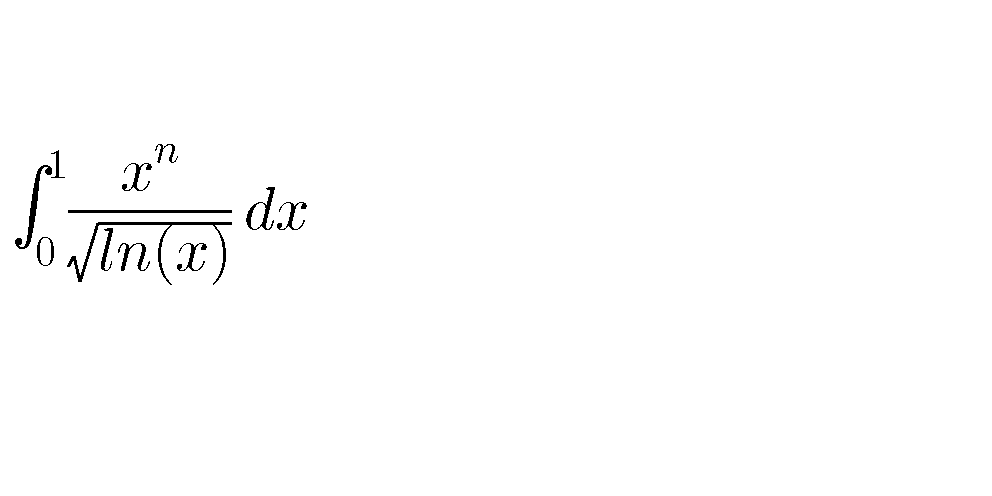
|
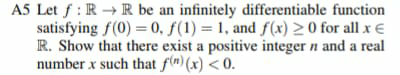
|
| calculate Σ_(n=2) ^∞ Σ_(k=2) ^∞ (1/(k^n k!)) |
| find ∫_0 ^∞ (x^2 /(e^x^2 −1))dx |
| ∫_(2π) ^(4π) (√(1−cos(x))) dx |
| If a = Cosα −iSinα and b = Cosβ −iSinβ Prove that (((a+b)(1−ab))/((a−b)(1+ab))) = ((Sinα+Sinβ)/(Sinα−Sinβ)) |

|
| lim_(x → ∞) (6^x /(2^x + 4^x )) = ∞ ? |
| ∫_0 ^(√(3−x^2 )) ((xy(4−x^2 −y^2 )(√(4−x^2 −y^2 ))−xy)/3) dy |
| Any integer(s) which fulfill n^5 − 5n^3 + 5n + 1 ∣ n! ? |
| Any integer(s) which fulfill n^3 − 5n^2 + 5n + 1 ∣ n! ? |

|

|
Pg 1435 Pg 1436 Pg 1437 Pg 1438 Pg 1439 Pg 1440 Pg 1441 Pg 1442 Pg 1443 Pg 1444 |