
Question and Answers Forum
AllQuestion and Answers: Page 1490







Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489 Pg 1490 Pg 1491 Pg 1492 Pg 1493 Pg 1494
|
Question and Answers Forum |
AllQuestion and Answers: Page 1490 |
| let f(x) =∫_x^2 ^(1+x) (dt/(1+t+t^2 )) 1) calculate f(x) interms of x 2) calculate lim_(x→0) f(x) and lim_(x→+∞) f(x) |
| 1) let f(x) =∫_0 ^(2π) ((cost)/(3 +sin(xt)))dt find a explicit form of f(x) 2) calculate g(x) =∫_0 ^(2π) ((tcos(xt)cost)/((3 +sin(xt))^2 ))dt 3) calculate ∫_0 ^(2π) ((cost)/(3+sint)) and ∫_0 ^(2π) ((t cos^2 t)/((3+sint)^2 ))dt |
| let f(x)=x^n arctan(x^2 ) with n integr natural 1) calculate f^((n)) (x) and f^((n)) (0) 2)developp f at integr serie . |
| let f(x) =(2x+1)ln(1−x^2 ) 1) calculate f^((n)) (x) and f^((n)) (0) 2)developp f at integr serie. 3) calculate ∫_0 ^1 f(x)dx . |
| calculate Σ_(n=0) ^∞ (((−1)^n )/(4n+3)) |

|

|
| 3x+5y=?_ |
| 1) calculate f(x)=∫_0 ^(π/4) ln(1+xtanθ)dθ 2) find the values of integrals ∫_0 ^(π/4) ln(1+tanθ) and ∫_0 ^(π/4) ln(1+2tanθ)dθ . 1) we have f^′ (x)=∫_0 ^(π/4) ((tanθ)/(1+xtanθ)) dθ =∫_0 ^(π/4) (((sinθ)/(cosθ))/(1+x((sinθ)/(cosθ))))dθ =∫_0 ^(π/4) ((sinθ)/(cosθ +xsinθ)) dθ =_(tan((θ/2))=t) ∫_0 ^((√2)−1) (((2t)/(1+t^2 ))/(((1−t^2 )/(1+t^2 )) +((2xt)/(1+t^2 )))) ((2dt)/(1+t^2 )) =∫_0 ^((√2)−1) ((4t)/((1+t^2 )(1−t^2 +2xt)))dt =−∫_0 ^((√2)−1) ((4t)/((t^2 +1)(t^2 −2xt −1)))dt let decompose F(t) = ((4t)/((t^2 +1)(t^2 −2xt −1))) roots of t^2 −2xt −1 Δ^′ =x^2 +1 ⇒t_1 =x+(√(x^2 +1)) and t_2 =x−(√(x^2 +1)) F(t)=(a/(t−t_1 )) +(b/(t−t_2 )) +((ct +d)/(t^2 +1)) a =lim_(t→t_1 ) (t−t_1 )F(t)=((4t_1 )/((t_1 ^2 +1)(t_1 −t_2 ))) =α b =lim_(t→t_2 ) (t−t_2 )F(t) =((4t_2 )/((t_2 ^2 +1)(t_2 −t_1 ))) =β ⇒F(t)=(α/(t−t_1 )) +(β/(t−t_2 )) +((ct +d)/(t^2 +1)) F(0) =0=−(α/t_1 ) −(β/t_2 ) +d ⇒d =(α/t_1 ) +(β/t_2 ) F(1)=(2/(−2x)) =−(1/x)=(α/(1−t_1 )) +(β/(1−t_2 )) +((c+d)/2) ⇒(1/x) =(α/(t_1 −1)) +(β/(t_2 −1)) −(c/2) −(d/2) ⇒(c/2) =(α/(t_1 −1)) +(β/(t_2 −1)) −(d/2) −(1/x) ⇒c =((2α)/(t_1 −1)) +((2β)/(t_2 −1)) −d−(2/x) ∫ F(t)dt =αln∣t−t_1 ∣ +βln∣t−t_2 ∣ +(c/2)ln(t^2 +1) +d arctan(t) ⇒ ∫_0 ^((√2)−1) F(t)dt =[αln∣t−t_1 ∣+βln∣t−t_2 ∣ +(c/2)ln(t^2 +1)]_0 ^((√2)−1) =αln∣(√2)−1−t_1 ∣ +βln∣(√2)−1−t_2 ∣ +(c/2)ln(4−2(√2)) =αln∣(√2)−1−x−(√(1+x^2 )))+βln∣(√2)−1−x+(√(1+x^2 ))) +((ln(4−2(√2)))/2)c =f^′ (x) ⇒ f(x)=∫ αln∣(√2)−1−x−(√(1+x^2 ))∣)dx+β∫ ln∣(√2)−1+(√(1+x^2 ))∣dx +((cx)/2)ln(4−2(√2)) +C ....be continued... |
| simplify the following expression if x<0 ∣4x−(√((3x−1)^2 ))∣ |

|

|
| make r the subject of the relation m=((4(√(u+r)))/(v−r)) |
| ∫_0 ^3 ∫_1 ^2 (x^3 +y^2 )dxdy |
| calculate lim_(ξ→0) ∫_1 ^(1+ξ) ((arctan(ξt))/t) dt . |
| calculate lim_(n→+∞) ∫_0 ^n (e^(nx) /(1+nx^2 )) dx . |
| log_2 (x^2 +7x−2)=log_2 (x^2 +3x−6)+log_4 8 find x |
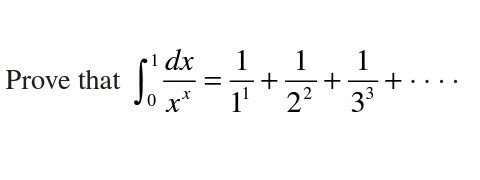
|

|
| let U_n =∫_(1/n) ^1 (√(x^2 +(3/n)))dx .calculate lim_(n→+∞) U_n |

|
| Please, can you help me with the following? A boat B is found at 40km at at the east of a boat A. A is moving at 30° east at 20km/h, B is moving qt 10km/h at 30°west. After how many hours will the two boats be the closest to each other? What will therefore be the position of the boat B and the boat A? Thank you |
| x^5 −4x^4 +6x^3 +8x−32=0 Find at least one root. |
| ∫(dα/(1−sin^3 α))=? ∫(dβ/(1−cos^3 β))=? ∫(dγ/(1−tan^3 γ))=? |
| x^2 +ax+(√2)b=0,has 2 roots:c and d,also x^2 +cx+(√2)d=0,has 2 roots:a and b.such that:a, b, c, d,are defferent non zero numbers. find possible value(s) for:a^2 +b^2 +c^2 +d^2 . |

|
Pg 1485 Pg 1486 Pg 1487 Pg 1488 Pg 1489 Pg 1490 Pg 1491 Pg 1492 Pg 1493 Pg 1494 |