
Question and Answers Forum
AllQuestion and Answers: Page 1495





Pg 1490 Pg 1491 Pg 1492 Pg 1493 Pg 1494 Pg 1495 Pg 1496 Pg 1497 Pg 1498 Pg 1499
|
Question and Answers Forum |
AllQuestion and Answers: Page 1495 |

|
| Find all functions y=f(x) such that y′y′′=y′′′. |
|

|

|
| find the difference of the roots of the following quadratic equation (3+2(√(2 )))x^2 +(1+(√2))x =2 |
| known function f diferensiable continues at [a, b] If f(a)=f(b)=0 and ∫_a ^b [f(x)]^2 dx=1 Prove that ∫_a ^b x^2 [f′(x)]^2 dx ≥(1/4) |
| Prove the following statements: If for every n , f_n form ascend function and {f_n } uniform convergences to f at [a, b], then lim_(n→∞) ∫_a ^b f_n (x) dx →∫_a ^b f(x) dx |
| Studies of convergences the numbers real sequence {x_n }, with x_1 =1 and x_(n+1) =((x_n ^2 +2)/(2x_n )), n≥1 |
| known real numbers sequence {a_n } and {b_n } both of them convergences to 0. If {b_n } monotonous descend and lim_(n→∞) ((a_(n+1) −a_n )/(b_(n+1) −b_n )) . then lim_(n→∞) (a_n /(2b_n ))=.. |
| Known a ∈ R and function f : R→R satiesfied ∣xf(x)+a∣ < sin^2 (x−a). For all x ∈ R value of lim_(x→a) f(x) .. |
| For all n ∈ N f_n (x)= { ((((nx)/(2n−1)), x ∈ [0, ((2n−1)/n)])),((1 , x ∈[((2n−1)/n), 2])) :} then for n→∞ ∫_1 ^2 f_n (x) dx convergences to.. |
| Value of lim_(n→∞) n ∫_0 ^1 ((2x^n )/(x+x^(2n+1) )) dx=.. |
| known function f:[−5, 4]→R continues, then E={x ∈ [−5, 4] : f(x)}, then closure from E is... |
| Series Σ_(n=1) ^(∞) (1/n^2 )=.. |
| If lim_(x→c) ((a_0 +a_1 (x−c)+a_2 (x−c)^2 +...+a_n (x−c)^n )/((x−c)^n ))=0 then a_0 +a_1 +a_2 +..+a_n =.. |
| Known set A⊆R not empty, If Sup A=Inf A, then set A is.. |
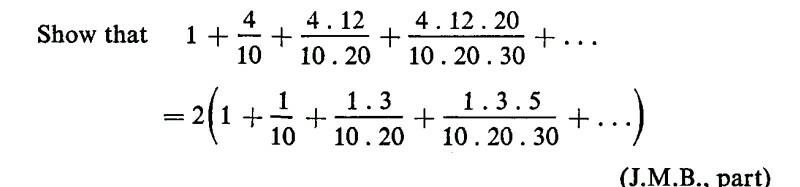
|
| In an A.P, the sum of the first 50 terms is 6275. Write this A.P . knowing that the ratio is 5. |
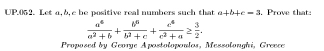
|
| The function pogof(x) = x^4 + 2x^3 + 2x^2 is divisible by the half of the function of p. Find g(x). |
| let F(α)=∫_α ^(1+α^2 ) ((sin(αx))/(1+αx^2 ))dx 1) calculate (dF/dα)(α) 2) calculate lim_(α→0) F(α) |

|
| lim_(x→π/3) ((cos x−sin (π/6))/((π/6)−(x/2)))=.. |
| If 12% of a number is equal to s, what is the e% of s? A. ((es)/(12)) B. ((es)/(88)) C. ((12s)/e) D. ((12e)/s) |

|
Pg 1490 Pg 1491 Pg 1492 Pg 1493 Pg 1494 Pg 1495 Pg 1496 Pg 1497 Pg 1498 Pg 1499 |